When two concentric shells are connected by a thin conducting wire, whole of the charge of inner shell transfers to the outer shell and potential difference between them becomes zero. Surface charge densities of two thin concentric spherical shells are $σ$ and $- σ$ respectively. Their radii are R and $2 R$ . Now they are connected by a thin wire.

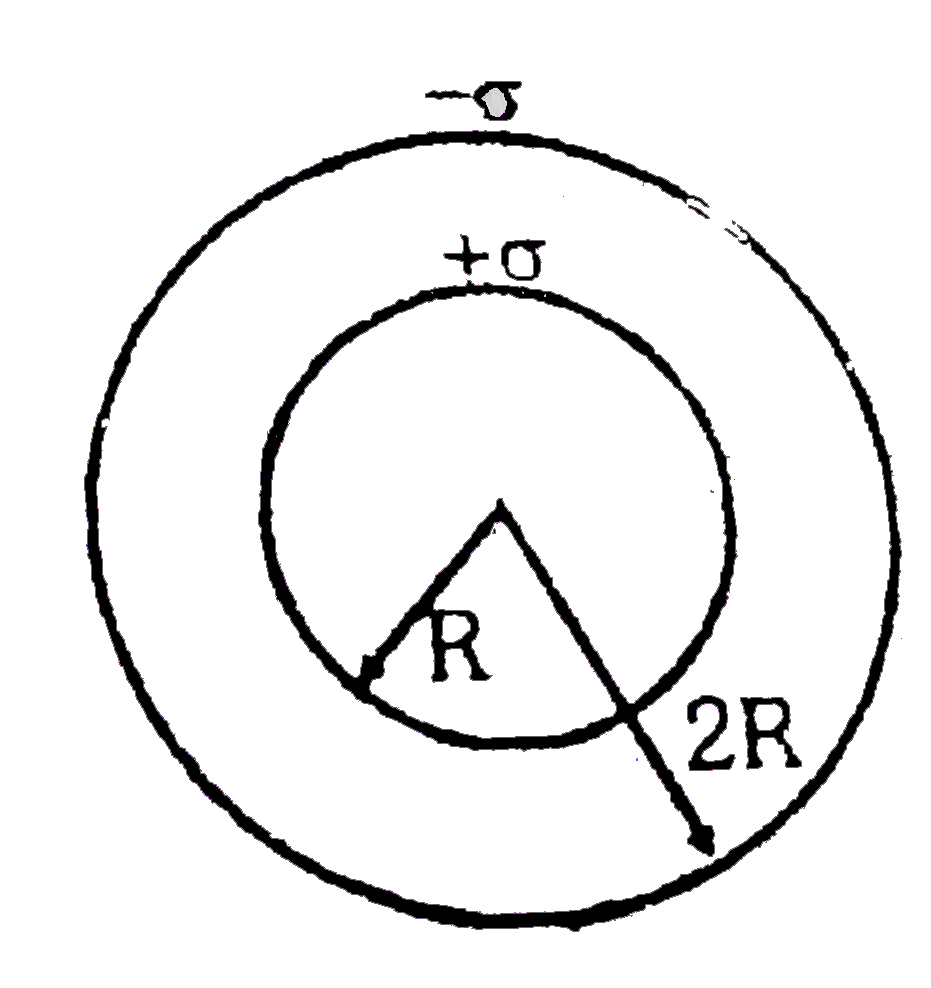
Potential on either of the shells will be :-

$- \frac{3 σ R}{2 ε_{0}}$

$\frac{2 σ R}{ε_{0}}$

$- \frac{σ R}{2 ε_{0}}$

zero

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Updated on:21/07/2023

Answer

Step by step video, text & image solution for When two concentric shells are connected by a thin conducting wire, whole of the charge of inner shell transfers to the outer shell and potential difference between them becomes zero. Surface charge densities of two thin concentric spherical shells are sigma and -sigma respectively. Their radii are R and 2R. Now they are connected by a thin wire. Potential on either of the shells will be :- by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 PHYSICS ELECTROSTATICS

Topper's Solved these Questions

Knowledge Check

When two concentric shells are connected by a thin conducting wire, whole of the charge of inner shell transfers to the outer shell and potential difference between them becomes zero. Surface charge densities of two thin concentric spherical shells are $σ$ and $- σ$ respectively. Their radii are R and $2 R$ . Now they are connected by a thin wire.

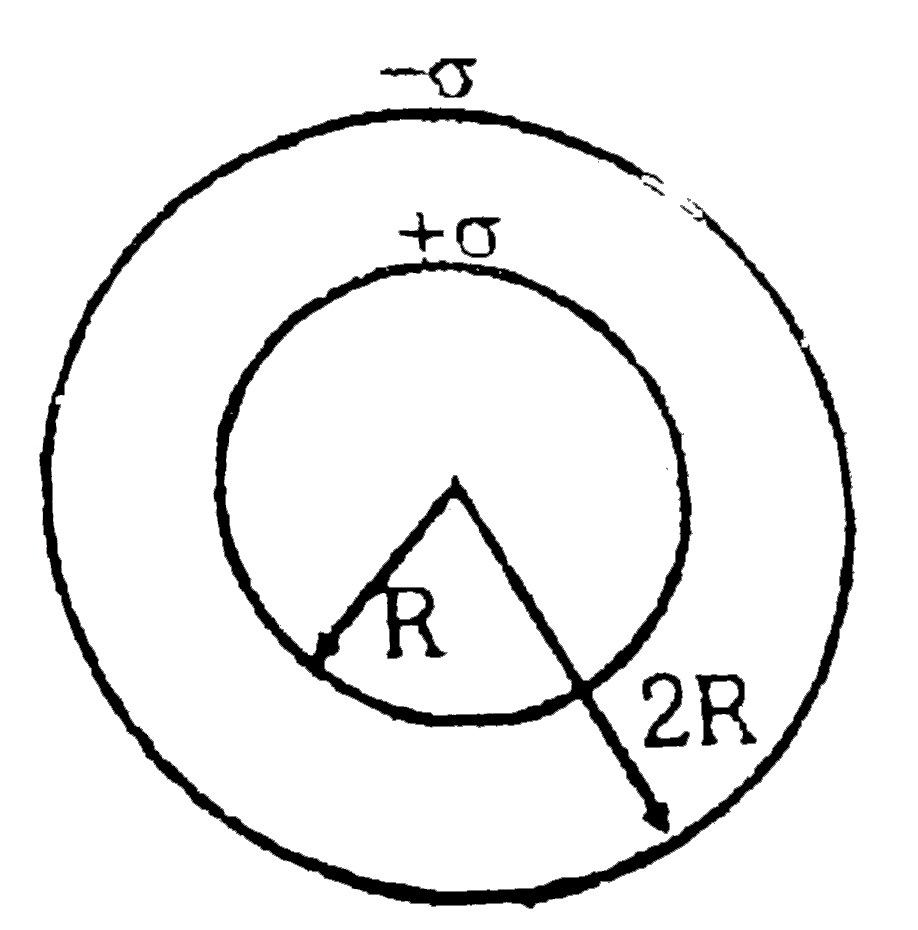
Suppose electric field at a distance $r (> 2 R)$ was $E_{1}$ before connecting the two shells and $E_{2}$ after connecting the two shells, then $∣ ∣ ∣ \frac{E_{2}}{E_{1}} ∣ ∣ ∣$ is :-
Azero
B1
C2
D $.^{1} /_{2}$
When two concentric shells are connected by a thin conducting wire, whole of the charge of inner shell transfers to the outer shell and potential difference between them becomes zero. Surface charge densities of two thin concentric spherical shells are $σ$ and $- σ$ respectively. Their radii are R and $2 R$ . Now they are connected by a thin wire.

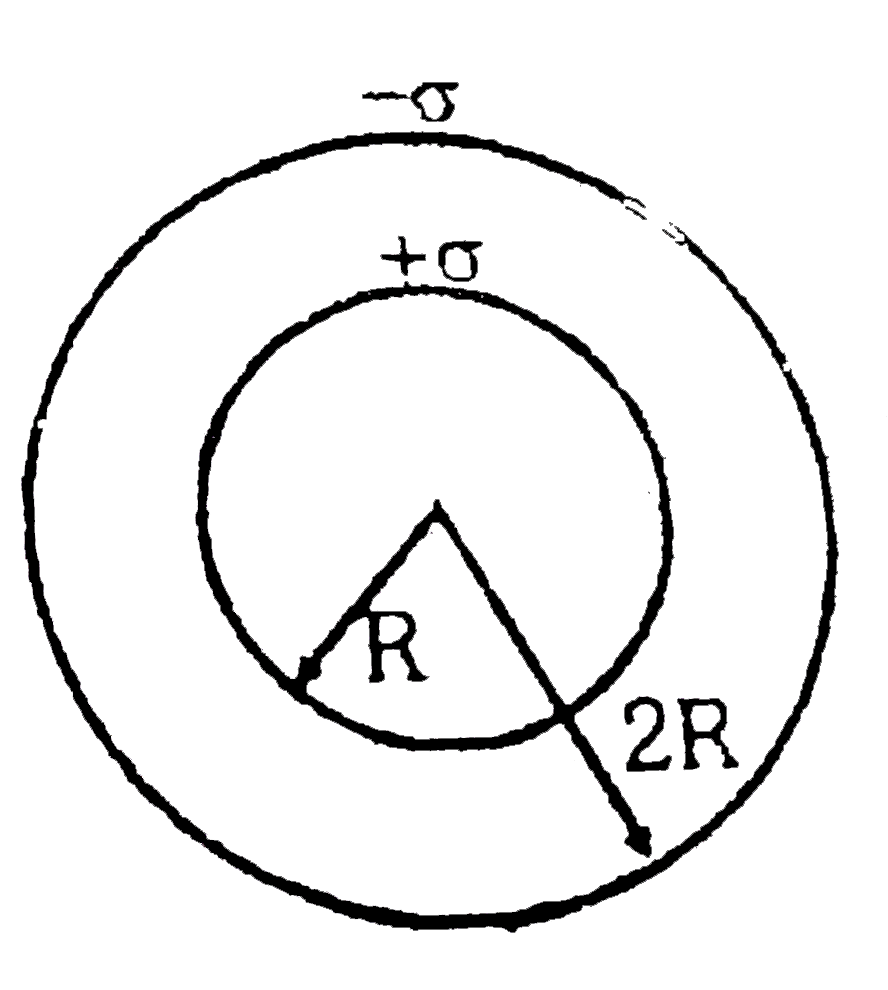
Suppose electric field at a distance $r = \frac{3 R}{2}$ was $E_{1}$ before connecting the two shells and $E_{2}$ after connecting the two shells, then $∣ ∣ ∣ \frac{E_{2}}{E_{1}} ∣ ∣ ∣$ is :-
Azero
B1
C $9 / 8$
D $8 / 9$
There are two concentric conducting shells. The potential of outer shell is 10 V and that of inner shell is 15 V. If the outer shell is grounded, the potential of inner shell becomes $/$ remains.
A25 V
B15 V
C10 V
D5 V

DC PANDEY-ELECTROSTATICS-Comprehension

Home
Ask Doubt
Profile

The correct Answer is:A

Topper's Solved these Questions

Similar Questions

Knowledge Check

There are two concentric conducting shells. The potential of outer shell is 10 V and that of inner shell is 15 V. If the outer shell is grounded, the potential of inner shell becomes / remains.

DC PANDEY-ELECTROSTATICS-Comprehension

There are two concentric conducting shells. The potential of outer shell is 10 V and that of inner shell is 15 V. If the outer shell is grounded, the potential of inner shell becomes $/$ remains.