A person speacking normally produces a sound intensity of 40dB AT A IDISTANCE OF 1M. If the threshold intensity for reasonable audiblity is 20dB, the maximum distance at which a person can be heard clearly is (2x) meter . Find the value of x.
The correct Answer is:5
To solve the problem step by step, we will use the relationship between sound intensity levels in decibels (dB) and the corresponding intensities.
Step 1: Understand the relationship between intensity and decibels
The sound intensity level in decibels (dB) is given by the formula:
β=10log(II0)
where:
- β is the sound intensity level in dB,
- I is the sound intensity,
- I0 is the reference intensity, typically 10−12W/m2.
Step 2: Calculate the intensity at 40 dB
Given that a person speaking normally produces a sound intensity of 40 dB at a distance of 1 meter, we can set up the equation:
40=10log(I1I0)
Dividing both sides by 10:
4=log(I1I0)
Exponentiating both sides gives:
I1I0=104
This implies:
I1=104I0
Step 3: Calculate the intensity at 20 dB
Next, we calculate the intensity at the threshold of reasonable audibility, which is 20 dB:
20=10log(I2I0)
Dividing both sides by 10:
2=log(I2I0)
Exponentiating both sides gives:
I2I0=102
This implies:
I2=102I0
Step 4: Find the ratio of intensities
Now we can find the ratio of the two intensities:
I1I2=104I0102I0=104102=102=100
Step 5: Relate intensity to distance
The intensity of sound is inversely proportional to the square of the distance from the source:
I1I2=R22R21
Where R1=1m (the distance at which the intensity is 40 dB) and R2 is the distance at which the intensity is 20 dB. Substituting the values we have:
100=R2212
This simplifies to:
R22=100
Taking the square root gives:
R2=10m
Step 6: Find the value of x
According to the problem, the maximum distance at which a person can be heard clearly is 2x:
R2=2x
Substituting R2=10m:
10=2x
Dividing both sides by 2 gives:
x=5
Final Answer
The value of x is:
5
The sound intensity level in decibels (dB) is given by the formula:
β=10log(II0)
where:
- β is the sound intensity level in dB,
- I is the sound intensity,
- I0 is the reference intensity, typically 10−12W/m2.
Given that a person speaking normally produces a sound intensity of 40 dB at a distance of 1 meter, we can set up the equation:
40=10log(I1I0)
Dividing both sides by 10:
4=log(I1I0)
Exponentiating both sides gives:
I1I0=104
This implies:
I1=104I0
Next, we calculate the intensity at the threshold of reasonable audibility, which is 20 dB:
20=10log(I2I0)
Dividing both sides by 10:
2=log(I2I0)
Exponentiating both sides gives:
I2I0=102
This implies:
I2=102I0
Now we can find the ratio of the two intensities:
I1I2=104I0102I0=104102=102=100
The intensity of sound is inversely proportional to the square of the distance from the source:
I1I2=R22R21
Where R1=1m (the distance at which the intensity is 40 dB) and R2 is the distance at which the intensity is 20 dB. Substituting the values we have:
100=R2212
This simplifies to:
R22=100
Taking the square root gives:
R2=10m
According to the problem, the maximum distance at which a person can be heard clearly is 2x:
R2=2x
Substituting R2=10m:
10=2x
Dividing both sides by 2 gives:
x=5
The value of x is:
5
Topper's Solved these Questions
Similar Questions
Loudness of sound from an isotropic point source at a distance of 10m is 20dB. If the distance at which it is not heard is 10K in meters find K.
View SolutionAirport authority has made the regulation that maximum allowable intensity level detected by a microphone situated at the end of 1630 m long runway can be 100 dB. An aeroplane when flying at a height of 200 m produces an intensity level of 100 dB on ground. while taking off, this aeroplane makes an angle of 30∘ with horizontal. Find the maximum distance this aeroplane can cover on the runway, so that the regulation are not violated (assume no reflection).
View Solution
Knowledge Check
A person speaking normally produces a sound intensity of 40 dB at a distance of 1 m. If the threshold intensity for reasonable audibility is 20 dB, the maximum distance at which he can be heard clearly is
A10 mB5 mC4 mD20 mA person speaking normally produces a sound intensity of 40dB at a distance of 1m. If the threshold intensity for reasonable audibility is 20dB, the maximum distance at which he can be heard cleary is.
A4 mB5 mC10 mD20 mTwo particles having masses m and 4m are separated by distance l. The distance of the centre of mass from m is x1andx2 is the distance of point at which gravitational field intensity is zero. Find the value of x1x2
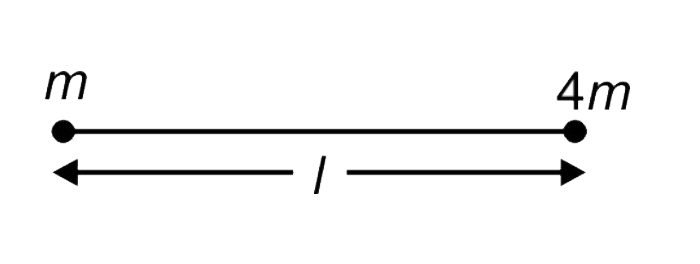 A1B2C2.4D3.6
A1B2C2.4D3.6
The block is always at rest, the maximum force which can be applied for this is 24x. Find the value of x. Take g = 10 m/s2{coefficient of friction = 0.3}
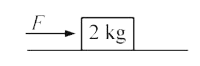
View SolutionTwo speakeer connected to the same source of fixed frequency are placed 2m apart in a box. A sensitive microphone placed at a distance of 4m from the midpoint alon the perpendicular bisector shown maximum response. The box is slowly rotated till the speaker are in line with the microphone, The distance between the midpoint of the speakers and the microphone remains unchanged. Exactly 5 maximum responses (inculuding the initial and last one) and observed in the microphone in doing this. The wavelength of the sound wave is (o.x) meter. Find the value of x.
View SolutionA particle is moving along positive X direction and is retarding uniformly. The particle crosses the origin at time t=0 and crosses the point x=4.0m at t=2s.
(a) Find the maximum speed that the particle can possess at x=0.
(b) Find the maximum value of retardation that the particle can have.
View Solution(a) A source of sound S and a detector D are placed at some distance from one another. A big cardboard is placed near the detector and perpendicular to the line SD as shown in figure. It is gradually moved away and it is found that the intensity changes from a maximum to a minimum as the board is moved through a distance of 20cm. Find the frequency of the sound emitted. Velocity of sound in are is 336m/s.

(b) A source emitting sound of frequency 180Hz is placed in front of a wall at a distance of 2m from it. A detector is also placed in front of the wall at the same distance from it. find the minimum distance between the source and the detector for which the detector detects a maximum of sound . Speed of sound in air =360m/s.
View SolutionThe faintest sound the human ear can detect at a frequency of 1000 Hz correspond to an intensity of about 1.00×10−12Wm2, which is called threshold of hearing. The loudest sounds the ear can tolerate at this frequency correspond to an intensity of about 1.00Wm2, the threshold of pain. Detemine the pressure amplitude and displacement amplitude associated with these two limits. Take speed of sound=342ms and density of air=1.20kgm3
View Solution
