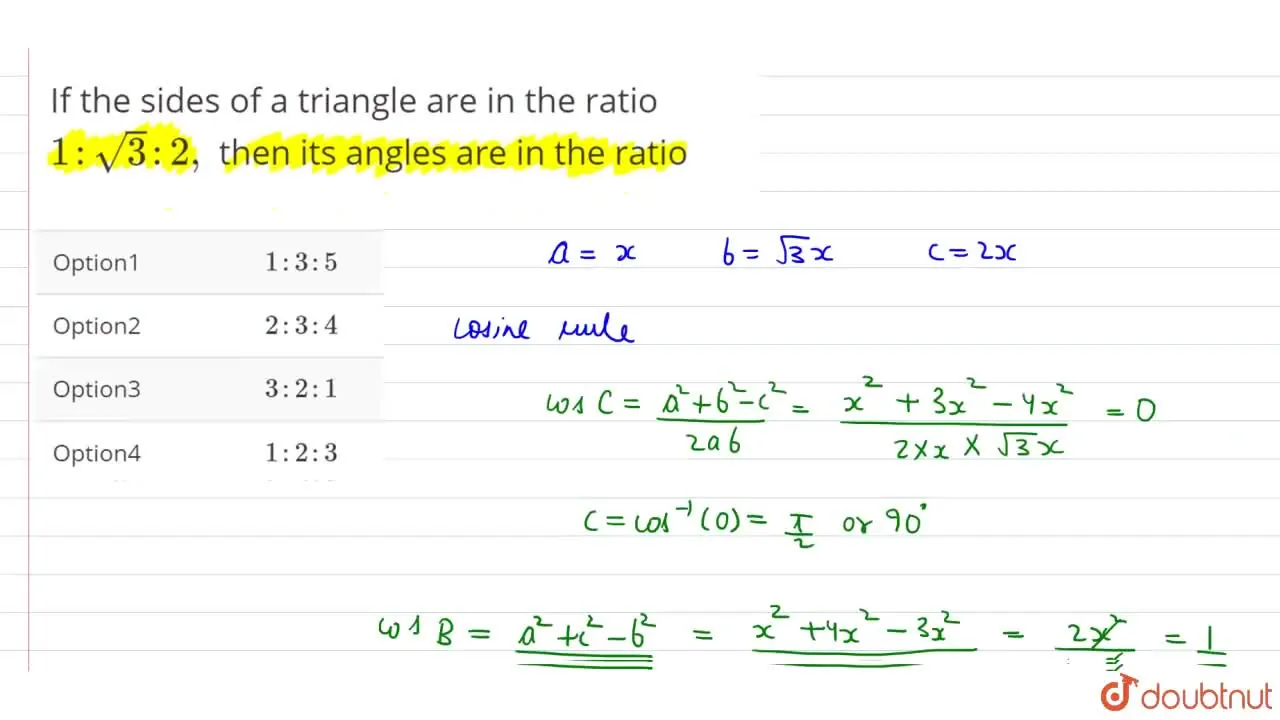
If the sides of a triangle are in the ratio 1:√3:2, then its angles are in the ratio
1:3:5
2:3:4
3:2:1
1:2:3
The correct Answer is:D
Step by step video, text & image solution for If the sides of a triangle are in the ratio 1 : sqrt(3) : 2, then its angles are in the ratio by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - MARVEL PUBLICATIONCHAPTER - TRIGONOMETRIC FUNCTIONSEXERCISE - MULTIPLE CHOICE QUESTIONS - PART - A : BUILDING-UP THE BASE152videos
TRIGONOMETRIC FUNCTIONS
- BOOK - MARVEL PUBLICATIONCHAPTER - TRIGONOMETRIC FUNCTIONSEXERCISE - MULTIPLE CHOICE QUESTIONS - PART - A : BUILDING-UP THE BASE (B) Properties of triangles27videos
TRIGONOMETRIC FUNCTIONS
- BOOK - MARVEL PUBLICATIONCHAPTER - TRIGONOMETRIC FUNCTIONSEXERCISE - TEST YOUR GRASP: CHAPTER 3-3.313videos
TRIGONOMETRIC FUNCTIONS
- BOOK - MARVEL PUBLICATIONCHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - MULTIPLE CHOICE QUESTIONS39videos
THREE DIMENSIONAL GEOMETRY
- BOOK - MARVEL PUBLICATIONCHAPTER - VECTORSEXERCISE - TEST YOUR GRASP20videos
VECTORS
Similar Questions
The sides of a triangle are in the ratio 1:√3:2. Then the angles are in the ratio
View SolutionThe sides of a triangle are in the ratio 2:√6:√3+1, then its angles are
View SolutionIf the sides of a triangle are in the ratio 1 : √3 : 2, then the angles of the triangle are in the ratio
View SolutionThe sides of a triangle are in the ratio 1:√3:2 then the angles of the triangle are in the ratio
View SolutionConsider the following statement :
1. There exixts no triangle ABC for which sin A + sin B = sin C.
2. If the angle of a triangle are in the ratio 1 : 2 : 3, then its sides will be in the ratio 1:√3:2.
Which of the above statement is/are correct?View SolutionThe angles of a triangle are in the ratio 1:2:3 , then the sides of a triangle are in the ratio
View SolutionIf the angles of a triangle are in the ratio 2 : 3 : 7 ,then the sides are in the ratio
View SolutionIn the sides of a triangle are in the ratio 1:√3:2, then the measure of its greatest angle is π6 (b)π3 (c) π2 (d) 2π3
View SolutionIf the sides a triangle are in the ratio 2:√6:(√3+1), then the largest angle of the triangle will be
View SolutionIf the angles of a triangle are in the ratio 2 : 3 : 7 ,then the sides are in the ratio
View Solution