A solid cylinder rolls up an inclined plane of angle of inclination 30∘. At the bottom of the inclined plane, the centre of mass of the cylinder has a speed of 5m/s.
(a) How far will the cylinder go up the plane ? (B) How long will it take to return to the bottom ?
104m
310m
413m
154m
The correct Answer is:D
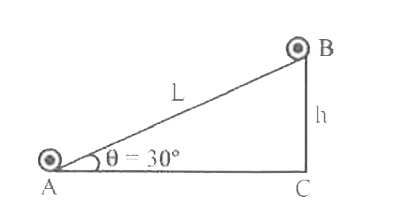
sinθ=hL ∴ L=hsinθ=h1/2=2h
Suppose that the solid cylinder of a radius R goes from A to B, where AB = L and height h =BC .
At A, the rolling energy of te cylinder
=12m2+12Iω2
For the solid cylinder, I=mR22andω=vR
∴ ERolling=12mv2+12.mR22×v2R2=34mv2
It slops at B and has the P.E. = mgh
By the principle of conservation of energy,
∴ mgh =34mv2 ∴h=3v24g
∴ L=2h=3v22g=3×5×52×10=154m
Topper's Solved these Questions
Similar Questions
A sphere rolls up an inclined plane whose inclination is 30∘. At the bottom of the inclined plane, the center of mass of the sphere has a translational speed of 5ms−1 (a) How far does the sphere travel up the plane? (b) How long does it take to return to the bottom?
View SolutionA solid cylinder rolls up an inclined plane of angle of inclinátion 30∘. At the bottom of the inclined plane the centre of mass of the cylinder has a speed of 5 m / s. How long (in seconds) will it take to return to the bottom? [Take g=10 m / (s)2],
View Solution
Knowledge Check
A solid cylinder rolls up an inclined plane of angle of inclination 30∘ . At the bottom of the inclined plane the centre of mass of the cylinder has a speed of 5ms−1 . How far will cylinder go up the plane ?
(Take g = 10ms−2)A154mB415 mC103 mD310 mIf a solid cylinder rolls down an inclined plane, then its:
Arotational energy is 1/3 and the translational energy 2/3 of its total K.E.Btranslational energy is 1/3 and rotational energy is 2/3 of its total K.E.Crotational and translational energies are equal.Dentire energy is rotational K.E.Choose the most appropriate option.
A cylinder rolls up an inclined plane at an angle of 30∘. At the bottom of the inclined plane, the centre of mass of the cylinder has speed of 5 m/s. How long will it take to return to the bottom?A2 sB3 sC1.5sD4 s
A cylinder rolls down an inclined plane of inclination 30∘, the acceleration of cylinder is
View SolutionA solid cylinder is rolling down a rough inclined plane of inclination θ. Then
View SolutionA solid cylinder of mass 2 kg and radius 50 cm rolls up an inclined plane of angle of inclination 30∘. The centre of mass of the cylinder has speed of 4 m/s. The distance travelled by the cylinder on the inclined surface will be, [ take g=10m/s2]
View SolutionA cylinder is rolling down on a inclined plane of inclination60∘. What is iths acceleration?
View SolutionA solid cylinder rolls up an inclined plane of inclination θ with an initial velocity v. How far does the cylinder go up the plane ?
View Solution