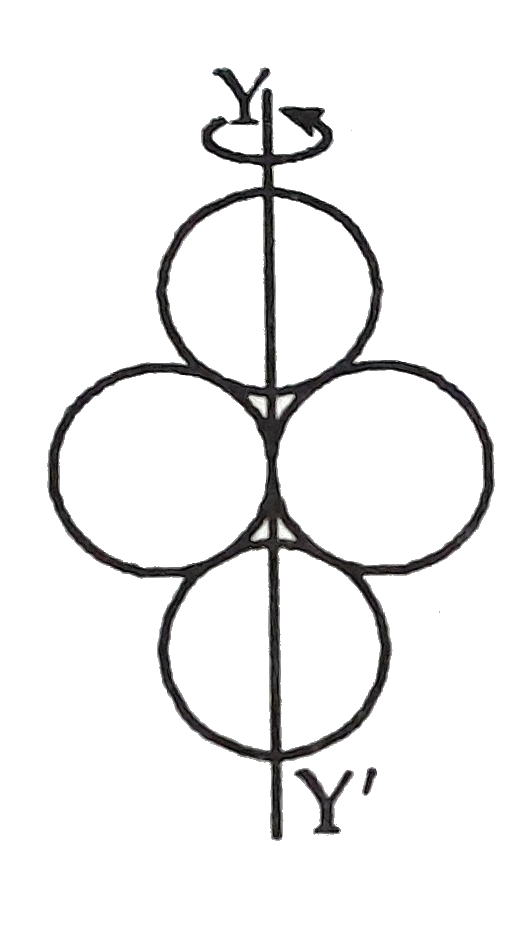
Two identical concentric rings each of mass m and radius R are placed perpendicularly. What is the moment of inertia of the system about the axis of one of the rings ?
3MR2
32MR2
14MR2
2MR2
The correct Answer is:B
To find the moment of inertia of the system consisting of two identical concentric rings placed perpendicularly about the axis of one of the rings, we can follow these steps:
Step 1: Understand the Configuration
We have two identical rings, each with mass m and radius R. One ring lies in the xy-plane, and the other lies in the xz-plane, making them perpendicular to each other.
Step 2: Moment of Inertia of One Ring
The moment of inertia I of a single ring about an axis passing through its center and perpendicular to its plane is given by the formula:
I=mR2
For the first ring (lying in the xy-plane), the moment of inertia about its own axis is:
I1=mR2
Step 3: Moment of Inertia of the Second Ring
For the second ring (lying in the xz-plane), we need to find the moment of inertia about the same axis as the first ring. Since the second ring is also a ring with mass m and radius R, we can use the perpendicular axis theorem.
According to the perpendicular axis theorem, the moment of inertia about an axis perpendicular to the plane of the ring is the sum of the moments of inertia about two perpendicular axes in the plane of the ring. Thus, the moment of inertia about the axis of the first ring is:
I2=12mR2+12mR2=mR2
Step 4: Total Moment of Inertia
Now, we can find the total moment of inertia of the system about the axis of one of the rings. Since the moment of inertia of each ring about the same axis is mR2, we can add them together:
Itotal=I1+I2=mR2+mR2=2mR2
Step 5: Conclusion
Thus, the moment of inertia of the system about the axis of one of the rings is:
Itotal=2mR2
We have two identical rings, each with mass m and radius R. One ring lies in the xy-plane, and the other lies in the xz-plane, making them perpendicular to each other.
The moment of inertia I of a single ring about an axis passing through its center and perpendicular to its plane is given by the formula:
I=mR2
For the first ring (lying in the xy-plane), the moment of inertia about its own axis is:
I1=mR2
For the second ring (lying in the xz-plane), we need to find the moment of inertia about the same axis as the first ring. Since the second ring is also a ring with mass m and radius R, we can use the perpendicular axis theorem.
I2=12mR2+12mR2=mR2
Now, we can find the total moment of inertia of the system about the axis of one of the rings. Since the moment of inertia of each ring about the same axis is mR2, we can add them together:
Itotal=I1+I2=mR2+mR2=2mR2
Thus, the moment of inertia of the system about the axis of one of the rings is:
Itotal=2mR2
Topper's Solved these Questions
Similar Questions
Knowledge Check
Two identical concentric rings each of mass m and radius R are placed perpendicularly. What is the moment of inertia of the system about the axis of one of the rings ?
A32MR2B2MR2CMR2D14MR2Three rings each of mass M and radius R are arranged as shown in the figure. The moment of inertia of the system about YY¢ will be
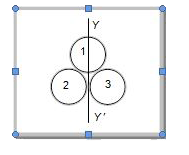 A3MR2B32MR2C5MR2D72MR2
A3MR2B32MR2C5MR2D72MR2Three rings, each of mass m and radius r, are so placed that they touch each other. Find the moment of inertia about the axis as shown in Fig.
 A5mr2B57mr2C7mr2D72mr2
A5mr2B57mr2C7mr2D72mr2
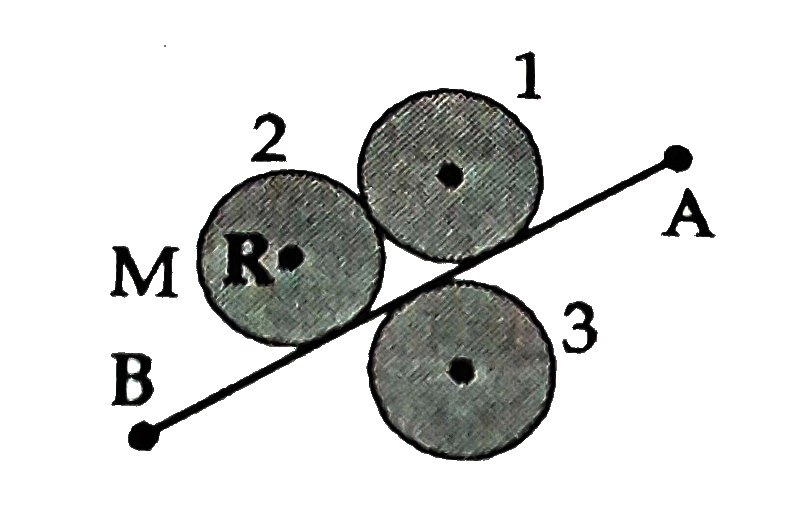
Three identical solid discs, each of mass M and radius R, are arranged as shown in figure. The moment of inertia of the system about an axis AB will be
View SolutionWhat is the moment of inertia of a ring about a tangent to the periphery of the ring?
View SolutionThree rings each of mass M and radius R are arranged according to the figure. The moment of inertia of this system about an axis XX' on its plane would be

View SolutionThree rings each of mass M and radius R are placed in contact with each other as shown . Then MI of the system about YY' axis is
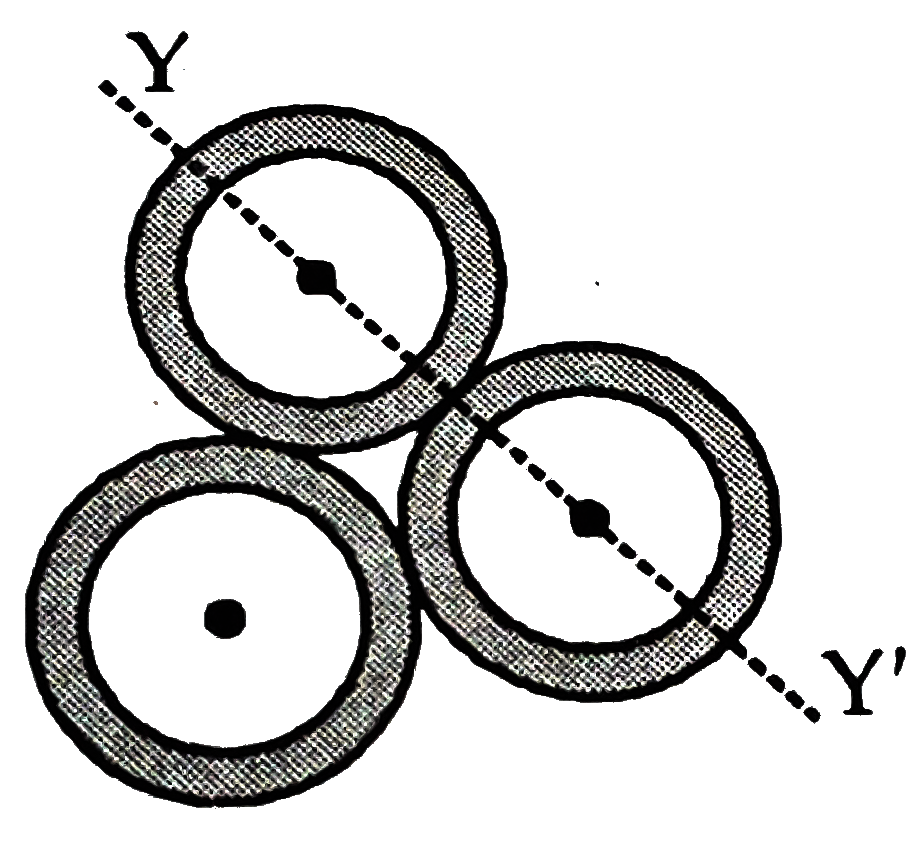
View SolutionThree rings each of mass m and radius r are so placed that they touch each other. The radius of gyration of the system about the axis as shown in the figure is
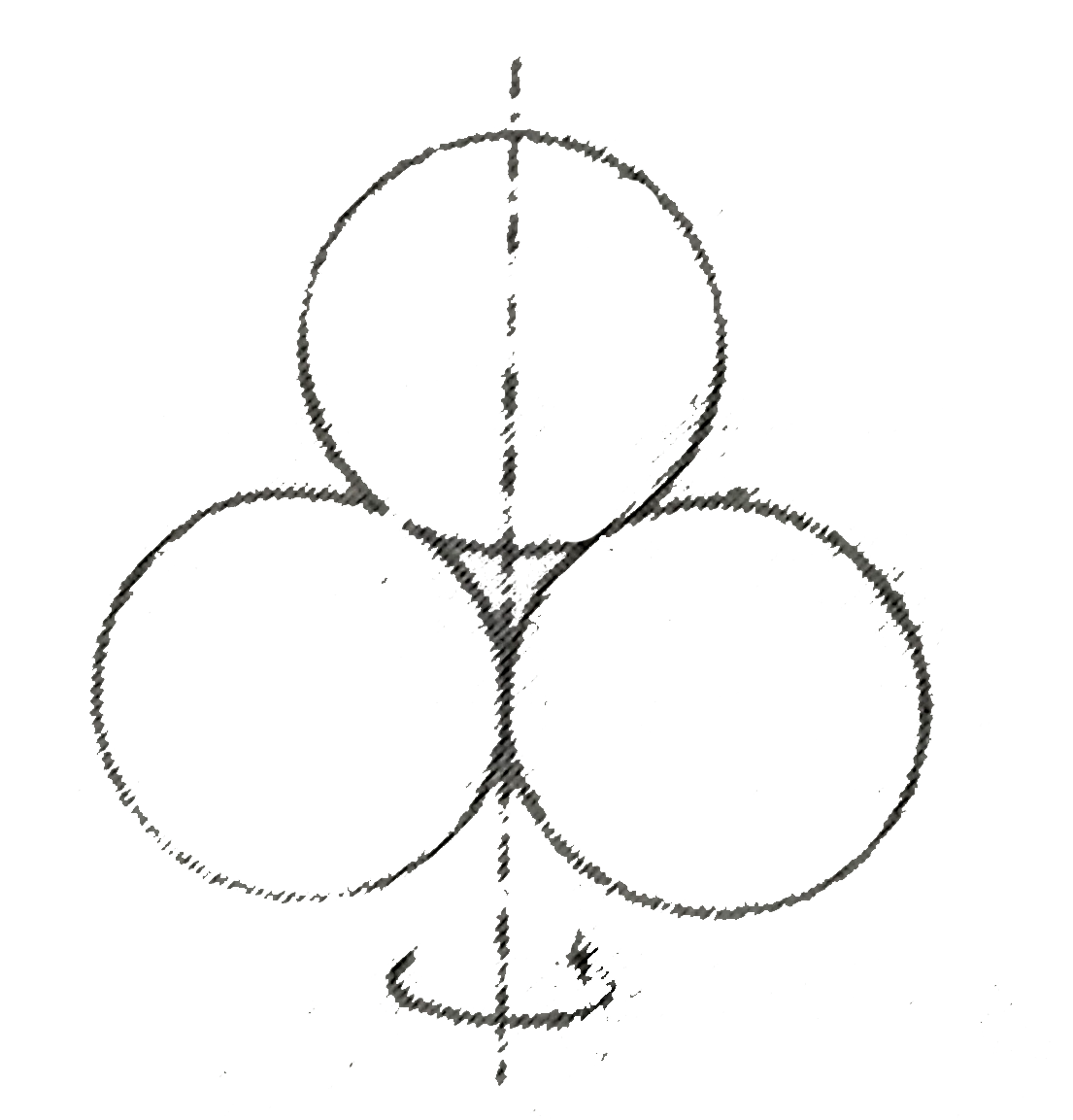
View Solution