If rho is the density of its material, then its rotational K.E. is giv

If $ρ$ is density of the material of a sphere of radius R . Then its moment of inertia about its diameter is
A $\frac{8 π}{15} ρ R^{5}$
B $\frac{4 π}{3} ρ R^{5}$
C $\frac{2 π}{5} ρ R^{5}$
D $\frac{4 π}{3} ρ R^{4}$
A material has normal density $ρ$ and bulk modulus $K$ . The increase in the density of the material when it is subjected to an external pressure $P$ from all sides is
A $P / ρ K$
B $K / ρ P$
C $ρ P / K$
D $ρ K / P$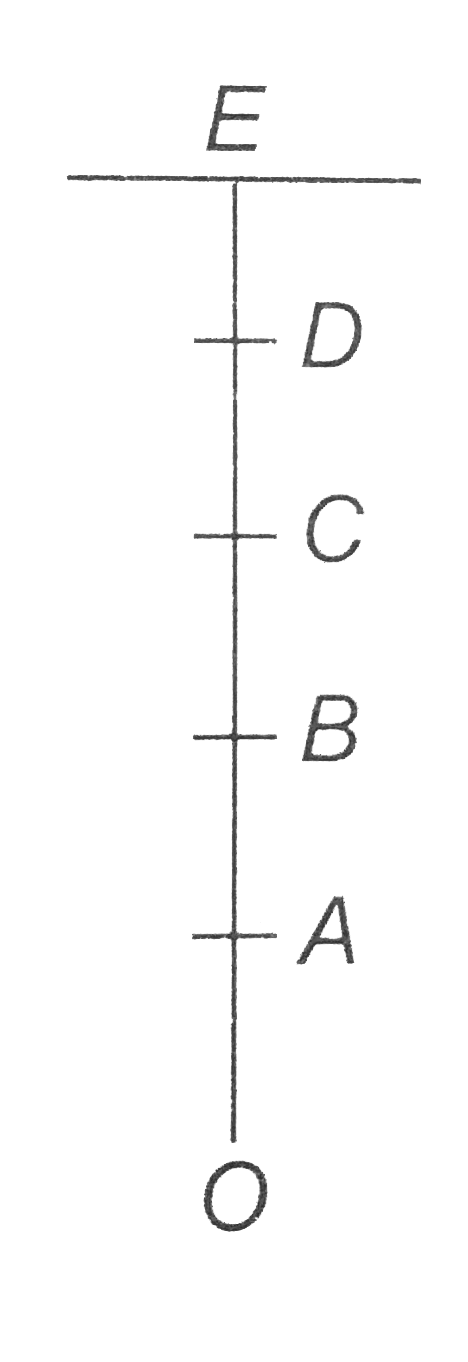
The length of wire $O E$ is divided into five equal parts $O A$ , $A B$ , $B C$ , $C D$ and $D E$ The wire is hanging from $E$ and its length is given by
$\frac{5}{4} \frac{σ}{ρ g}$ , where $σ$ is the breaking stress and $ρ$ is the density of the material of the wire. Find the point at which wire will break
A $A$
B $B$
C $C$
D $D$

If $ρ$ is the density of its material, then its rotational K.E. is given by