Two bodies having masses m1 and m2 and velocities v1 and v2 colide and form a composite system. If m1v1+m2v2=0(m1≠m2. The velocity of composite system will be
v1−v2
v1+v2
v1+v22
zero
The correct Answer is:D
To find the velocity of the composite system formed by two bodies with masses m1 and m2 and velocities v1 and v2, we can follow these steps:
Step 1: Understand the Conservation of Momentum
In a collision, the total momentum before the collision is equal to the total momentum after the collision. This is based on the principle of conservation of momentum.
Step 2: Write the Expression for Initial Momentum
The initial momentum of the system before the collision can be expressed as:
Pinitial=m1v1+m2v2
Step 3: Set Up the Equation for Final Momentum
After the collision, the two bodies stick together and move as a composite system with a combined mass of m1+m2 and a final velocity V. Therefore, the final momentum can be expressed as:
Pfinal=(m1+m2)V
Step 4: Apply the Conservation of Momentum
According to the conservation of momentum, we have:
Pinitial=Pfinal
Substituting the expressions we wrote:
m1v1+m2v2=(m1+m2)V
Step 5: Solve for the Final Velocity V
We can rearrange the equation to solve for V:
V=m1v1+m2v2m1+m2
Step 6: Use the Given Condition
From the problem, we know that:
m1v1+m2v2=0
This implies:
V=0m1+m2=0
Conclusion
Thus, the velocity of the composite system after the collision is:
V=0
In a collision, the total momentum before the collision is equal to the total momentum after the collision. This is based on the principle of conservation of momentum.
The initial momentum of the system before the collision can be expressed as:
Pinitial=m1v1+m2v2
After the collision, the two bodies stick together and move as a composite system with a combined mass of m1+m2 and a final velocity V. Therefore, the final momentum can be expressed as:
Pfinal=(m1+m2)V
According to the conservation of momentum, we have:
Pinitial=Pfinal
Substituting the expressions we wrote:
m1v1+m2v2=(m1+m2)V
We can rearrange the equation to solve for V:
V=m1v1+m2v2m1+m2
From the problem, we know that:
m1v1+m2v2=0
This implies:
V=0m1+m2=0
Thus, the velocity of the composite system after the collision is:
V=0
Topper's Solved these Questions
- CENTRE OF MASSBook:DC PANDEYChapter:CENTRE OF MASSExercise:Taking it together
Explore 73 Videos
- CENTRE OF MASSBook:DC PANDEYChapter:CENTRE OF MASSExercise:Assertion and reason
Explore 21 Videos
- CENTRE OF MASSBook:DC PANDEYChapter:CENTRE OF MASSExercise:Medical entrances gallery
Explore 27 Videos
- CALORIMETRY AND HEAT TRANSFERBook:DC PANDEYChapter:CALORIMETRY AND HEAT TRANSFERExercise:Medical entrance s gallery
Explore 38 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBook:DC PANDEYChapter:CENTRE OF MASS, IMPULSE AND MOMENTUMExercise:Comprehension type questions
Explore 15 Videos
Similar Questions
Two particles having masses m1andm2 are moving with velocities →V1and→V2 respectively. →V0 is velocity of centre of mass of the system.
(a) Prove that the kinetic energy of the system in a reference frame attached to the centre of mass of the system is KEcm=12μV2rel. Where μ=m1m2m1+m2 and Vrel is the relative speed of the two particles.
(b) Prove that the kinetic energy of the system in ground frame is given by
KE=KEcm+12(m1+m2)V20
(c) If the two particles collide head on find the minimum kinetic energy that the system has during collision.
View SolutionTwo masses, m1 and m2 , are moving with velocities v1 and v2. Find their total kinetic energy in the reference frame of centre of mass.
View Solution
Knowledge Check
Two bodies P and Q of masses m1 and m2 (m2>m1) are moving with velocity v1 and v2 force exerted by P on Q during the collision is
Agreater that the force exerted by Q on PBless than the force exerted by Q on PCsame as the force exerted by Q on PDsam as the force exerted by Q on P but opposite in directionTwo particles of masses m1 and m2 in projectile motion have velocities →v1 and →v2 , respectively , at time t=0. They collide at time t0. Their velocities become →v'1 and →v'2 at time 2t0 while still moving in air. The value of ∣∣∣(m1→v'1+m2→v'2)−(m1→v1+m2→v2)∣∣∣
AzeroB(m1+m2)gt0C2(m1+m2)gt0D12(m1+m2)gt0Two masses mA and mB moving with velocities vA and vB in opposite direction collide elastically after that the masses mA and mB move with velocity vB and vA respectively. The ratio (mA/mB) is
A1BvA−vBvA+vBC(mA+mB)/mADvA/vB
The velocities of two particles of masses m1 and m2 relative to an observer in an inertial frame are v1 and v2. Determine the velocity of the center of mass relative to the observer and the velocity of each particle relative to the center of mass.
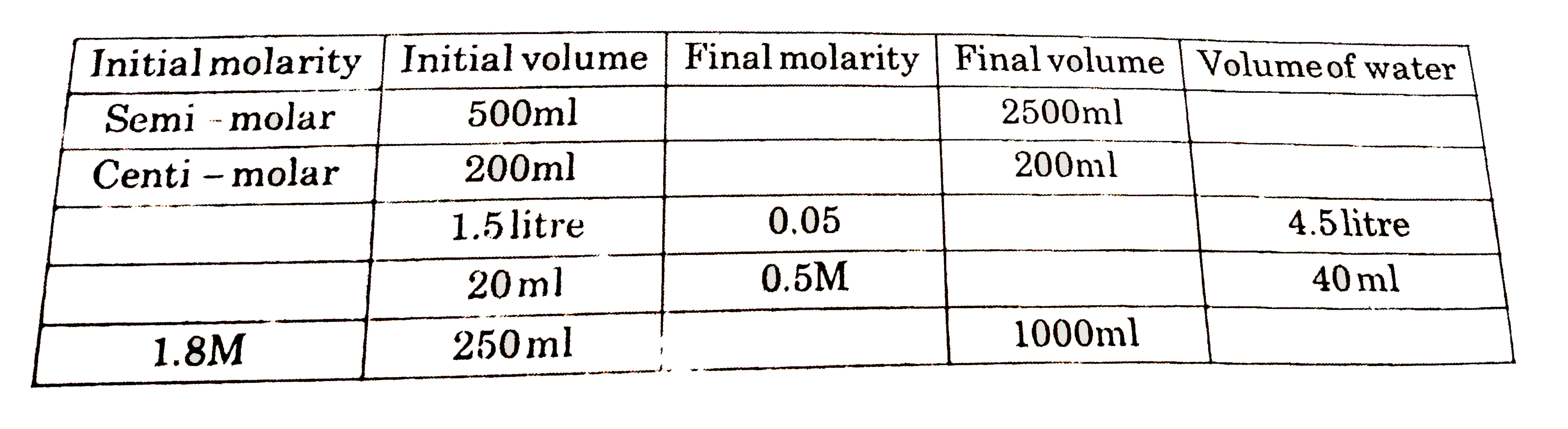
View SolutionUse of dilution formula (M1V1=M2V2)
View SolutionTwo balls with masses m1=3 and m2=5kg have initial velocities v1=v2=5m/s in the directions shown in figure. They collide at the origin.
a. find the velocioty of the CM 3s before the collision.
b. Find the position of the CM 2s after the collision.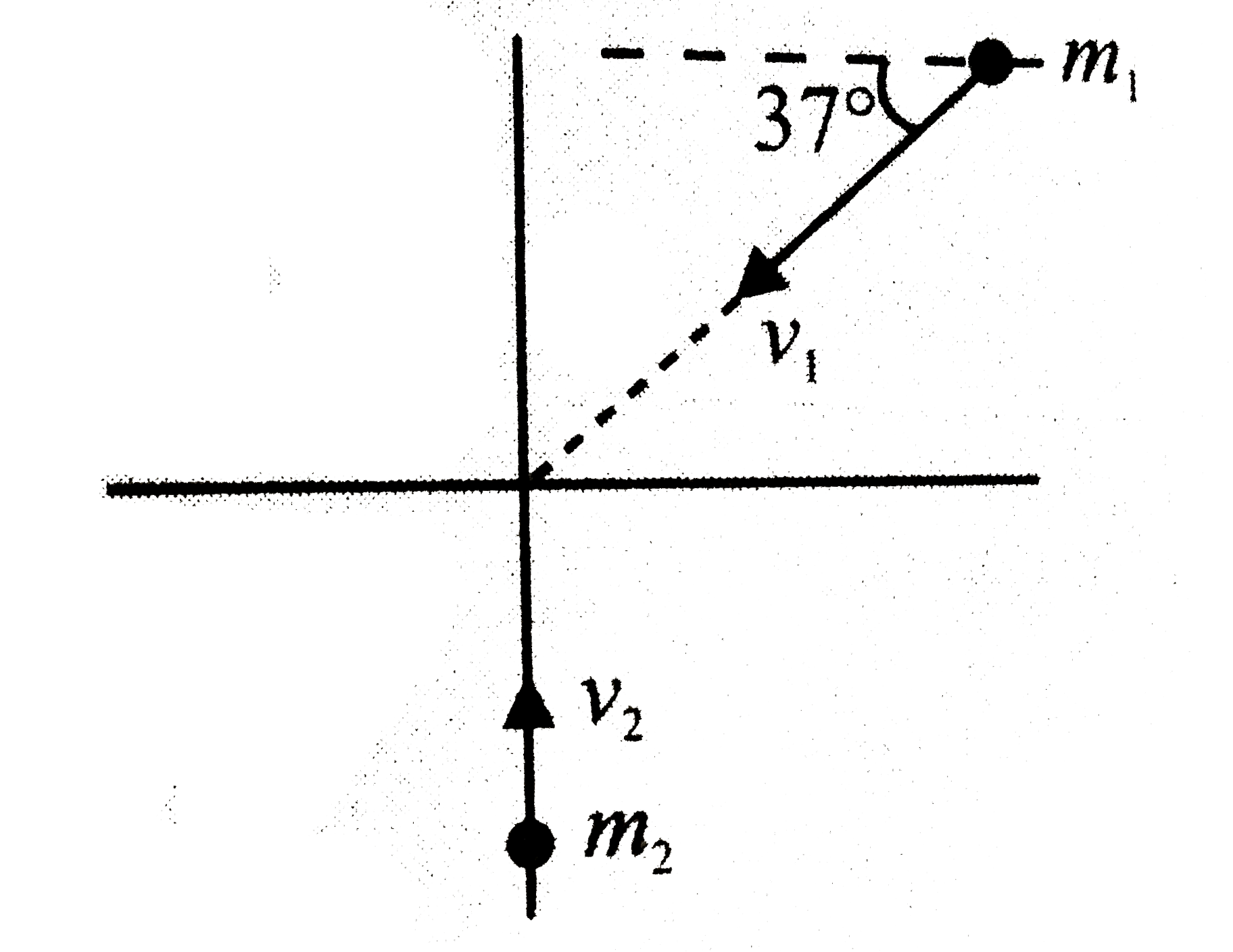
View SolutionTwo objects with masses m1andm2 are moving along the x-axis in the positive direction with speeds v1,andv2 , respectively, where v1 is less than v2 . The speed of the centre of mass of this system of two bodies is
View SolutionThe centre of mass of a body is a point at which the entire mass of the body is supposed to be concentrated. The position vector →r of c.m of the system of tow particles of masses m1 and m2 with position vectors →r1 and →r2 is given by
→r=m1→r1+m2→r2m1+m2
For an isolated system, where no external force is acting, −−→vcm= constant
Under no circumstances, the velocity of centre of mass of an isolated system can undergo a change
With the help of the comprehension given above, choose the most appropriate alternative for each of the following questions :
An electron and a proton move towards eachother with velocities v1 and v2 respectively. the velocity of their centre of mass is
View Solution
