All the particles of a body situated at distance $d$ from the origin. The distance of the center of mass of the body from the origin is

A

$= R$

B

$\leq R$

C

$> R$

D

$\geq R$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

For a single particle, distance of center of mass from orirign is R. For more than one particle distance $\leq R)$

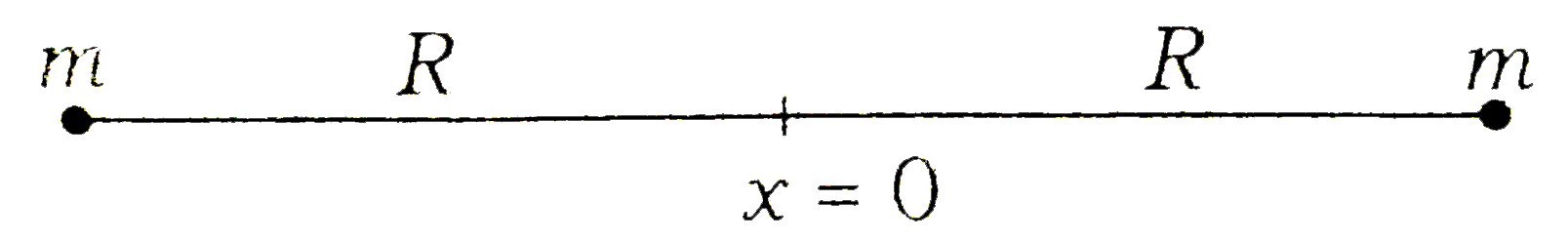
For example, for two particles of equal mass, kept as shown in figure, distance = 0.

|

Updated on:21/07/2023

Class 11 PHYSICS CENTRE OF MASS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
All the particles of a body are situated at a distance R from the origin. The distance of the centre of mass of the body from the origin is
A $= R$
B $\leq R$
C $> R$
D $\geq R$
Question 2 - Select One
A large number of particles are placed around the origin, each at a distance R from the origin. The distance of the center of mass of the system from the origin is
A $= R$
B $\leq R$
C $> R$
D $\geq R$
Question 3 - Select One
The distance of the $B (6, 6)$ from the origin is:
A $\sqrt{53}$ units
B $\sqrt{41}$ units
C $\sqrt{72}$ units
D $\sqrt{145}$ units

DC PANDEY-CENTRE OF MASS-Check points

Home
Ask Doubt
Profile