The front of a roller-coaster car is at the bottom of a hill and is 15 feet above the ground. If the front of the roller-coaster car rises at a constant rate of 8 feet per second, which of the following equations gives the height h, in feet, of the front of the roller-coaster car s seconds after it starts up the hill?
h = 8s + 15
h=15s+3358
h=8s+33515
h = 15s + 8
The correct Answer is:A
It’s given that the front of the roller-coaster car starts rising when it’s 15 feet above the ground. This initial height of 15 feet can be represented by a constant term, 15, in an equation. Each second, the front of the roller-coaster car rises 8 feet, which can be represented by 8s. Thus, the equation h = 8s + 15 gives the height, in feet, of the front of the roller-coaster car s seconds after it starts up the hill.
Choices B and C are incorrect and may result from conceptual errors in creating a linear equation. Choice D is incorrect and may result from switching the rate at which the roller-coaster car rises with its initial height.
Similar Questions
40% of all high school students hate roller coasters, the rest love them. 20% of those students who love roller coasters own chinchillas. What percentage of students love roller coasters but do not own a chinchilla?
View Solutionएक पहाड़ी की चोटी की वक्रता त्रिज्या 20 मी है। एक रोलर कोस्टर को इस तरह बनाया है की जब इसमें जा रहे यात्री पहाड़ी की चोटी के परितः घूमते है , तो उन्हें ' भारहीनता ' का आभास होता है। पहाड़ी की चोटी पर कार की चाल होगी
View Solutionचित्रानुसार वक्र पथ पर एक छोटा रोलर कॉस्टर बिन्दु A से u चाल के साथ गति प्रारम्भ करता है
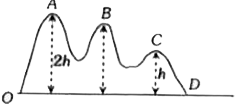
रोलर कॉस्टर तथा पथ के मध्य घर्षण नगण्य है तथा ये निरन्तर पथ के सम्पर्क में बना रहता है। पथ के बिन्दु D पर रोलर कॉस्टर की गति होगी|
View SolutionA small roller coaster starts at point A with a speed u on a curved track as shown in the figure The friction between the roller coaster and the track is negligible and it always remains in contact with the track. The speed of roller coaster at point D on the track will be
View SolutionWhen the roller coaster is on the ground its .............. (kinetic energy/potential energy) is zero.
View SolutionAt the highest point a roller coaster has
View SolutionIn a roller-coaster ride, the energy at the start is all ............ (potential energy/kinetic energy) as the cart is at the maximum height.
View SolutionExplain energy conversions in a roller-coaster ride.
View Solution
