Question

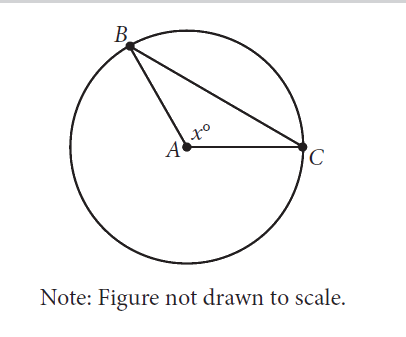
In the circle above, point A is the center and the length of arc $\stackrel{⌢}{BC}$ is $\frac{2}{5}$ of the circumference of the circle. What is the value of x ?

Answer

144

Explanation

In a circle, the ratio of the length of a given arc to the circle’s circumference is equal to the ratio of the measure of the arc, in degrees, to `360^@`. The ratio between the arc length and the circle’s circumference is given as `2/5` . It follows that `2/5` = `x/360` . Solving this proportion for x gives x = 144.