Question

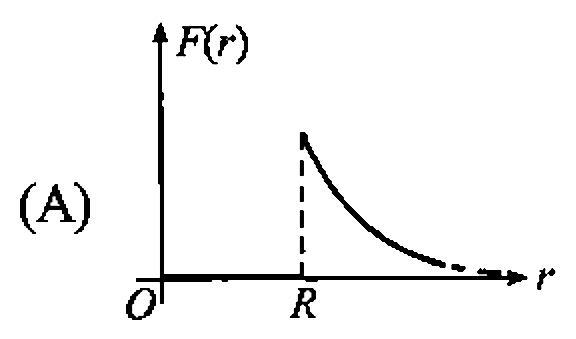
A particle of mass m is ocated at a distance r from the centre of shell of mass M and radius R. The force between the shell and mass $F\left(r\right)$. The plot of $F\left(r\right)$ ve r is :

Options

A.
B.
C.
D.

Answer

A

Explanation

To solve the problem, we need to analyze the gravitational force between a particle of mass $$m$$ located at a distance $$r$$ from the center of a spherical shell of mass $$M$$ and radius $$R$$. We will consider two cases: when the particle is inside the shell and when it is outside the shell.

Step 1: Analyze the Case When \( r < R \) (Inside the Shell)
- According to the shell theorem, the gravitational force inside a uniform spherical shell is zero. This means that if the particle of mass \( m \) is located anywhere inside the shell (i.e., \( r < R \)), the gravitational force \( F(r) \) acting on it due to the shell is:
\( F(r) = 0 \quad \text{for } r < R \)

Step 2: Analyze the Case When \( r \geq R \) (Outside the Shell)
- When the particle is outside the shell (i.e., \( r \geq R \)), the entire mass of the shell can be treated as if it were concentrated at its center. The gravitational force \( F(r) \) acting on the particle is given by Newton's law of gravitation:
\( F(r) = \frac{G M m}{r^2} \)
where \( G \) is the gravitational constant.

Step 3: Combine the Results
- We can summarize the results as follows:
- For \( r < R \): \( F(r) = 0 \)
- For \( r \geq R \): \( F(r) = \frac{G M m}{r^2} \)

Step 4: Plotting the Graph
- The graph of \( F(r) \) versus \( r \) will have two distinct regions:
- For \( r < R \), the force \( F(r) \) is zero, which means the graph will be a horizontal line along the \( F \)-axis at \( F = 0 \).
- For \( r \geq R \), the force \( F(r) \) decreases with \( r^2 \), which means the graph will show a hyperbolic decline as \( r \) increases.

Conclusion
- The plot of \( F(r) \) versus \( r \) will look like this:
- A horizontal line at \( F = 0 \) for \( r < R \)
- A curve that decreases as \( r \) increases for \( r \geq R \)

Final Answer
The correct option for the plot of \( F(r) \) versus \( r \) is option A.

---