Question

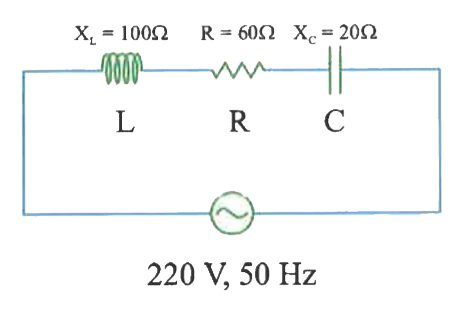
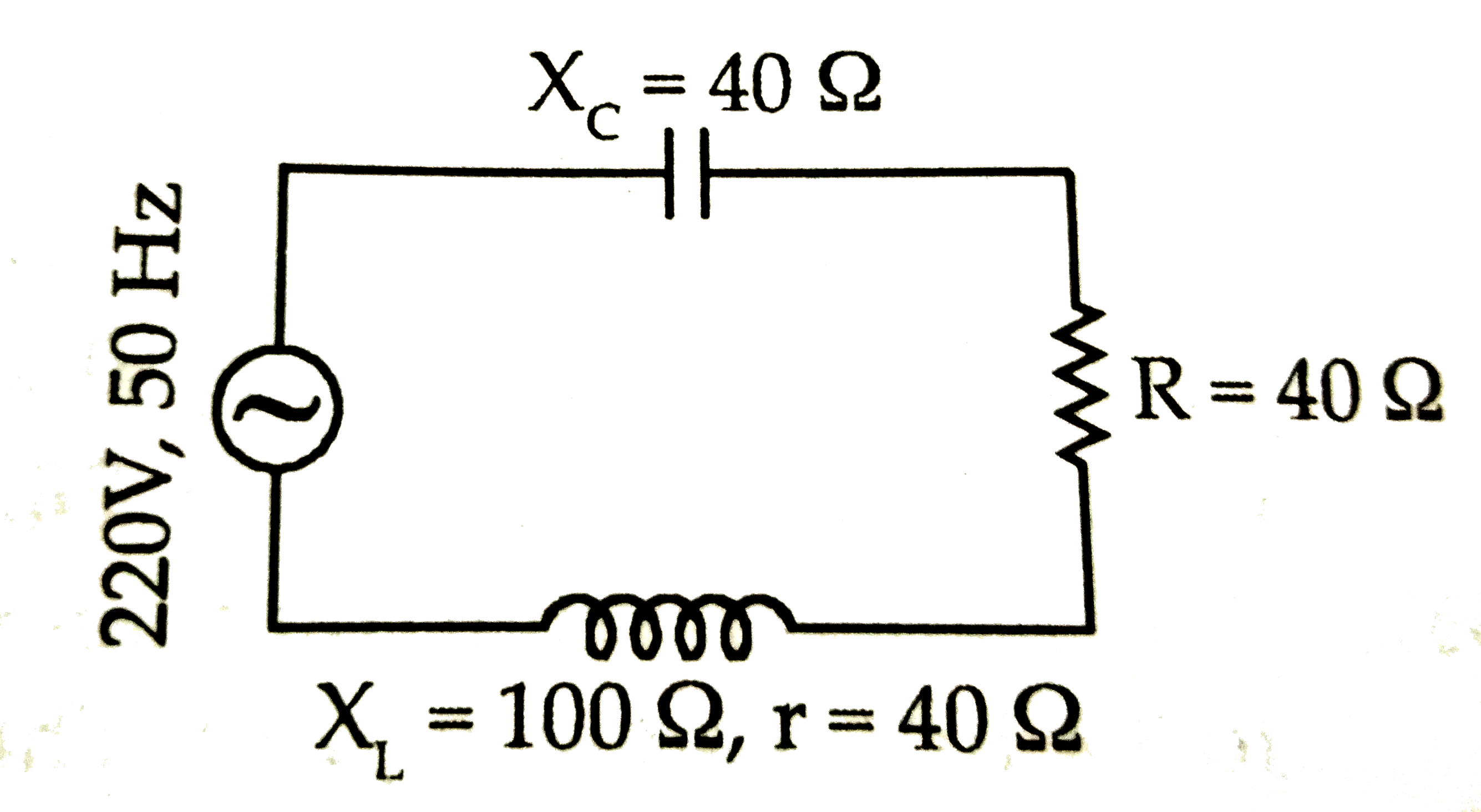
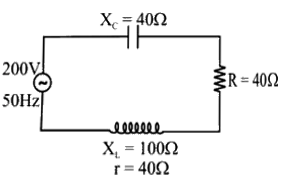
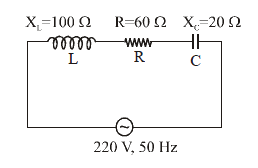In an $AC$ circuit, the reactannce is equal to the resistance. The power factor of the circuit will be

Options

A. 1
B. `1/2`
C. `1/(sqrt(2))`
D. zero

Answer

C

Explanation

To find the power factor of an AC circuit where the reactance (X) is equal to the resistance (R), we can follow these steps:

1. Understand the Power Factor:
The power factor (PF) of an AC circuit is defined as the cosine of the phase angle (φ) between the voltage and the current. Mathematically, it is given by:
\( \text{Power Factor} = \cos(\phi) \)

2. Relate Reactance and Resistance:
In the given circuit, we know that the reactance (X) is equal to the resistance (R). Therefore, we can express this relationship as:
\( X = R \)

3. Determine the Phase Angle:
The phase angle (φ) can be determined using the formula:
\( \tan(\phi) = \frac{X}{R} \)
Since \(X = R\), we can substitute to get:
\( \tan(\phi) = \frac{R}{R} = 1 \)

4. Calculate the Phase Angle:
The angle whose tangent is 1 is:
\( \phi = 45^\circ \)

5. Calculate the Power Factor:
Now, we can find the power factor using the cosine of the phase angle:
\( \text{Power Factor} = \cos(45^\circ) \)
The value of \(\cos(45^\circ)\) is:
\( \cos(45^\circ) = \frac{1}{\sqrt{2}} \)

Final Answer:
Thus, the power factor of the circuit is:
\( \text{Power Factor} = \frac{1}{\sqrt{2}} \approx 0.707 \)