A ball is thrown at angle θ and another ball is thrown at an angle (90∘−θ) with the horizontal direction from the same point with velocity 39.2ms−1. The second ball reaches 50 m higher than the first ball. Find their individual heights. Take g=9.8ms−2.
Step by step video & image solution for A ball is thrown at angle theta and another ball is thrown at an angle (90^(@)-theta) with the horizontal direction from the same point with velocity 39.2 ms^(-1). The second ball reaches 50 m higher than the first ball. Find their individual heights. Take g = 9.8 ms^(-2). by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROJECTILE MOTIONBOOK - SL ARORACHAPTER - PROJECTILE MOTIONEXERCISE - Problen Solution
17 Videos
- PROJECTILE MOTIONBOOK - SL ARORACHAPTER - PROJECTILE MOTIONEXERCISE - Problem For Self Practice
50 Videos
- PHYSICAL WORLD AND MEASUREMENTSBOOK - SL ARORACHAPTER - PHYSICAL WORLD AND MEASUREMENTSEXERCISE - Based on Combination
21 Videos
- PROPERTIES OF SOLIDSBOOK - SL ARORACHAPTER - PROPERTIES OF SOLIDSEXERCISE - All Questions
97 Videos
Similar Questions
A ball is thrown at an angle θ and another ball is thrown at an angle (90∘−tehta)withthehorizontaldirectionomthesamep∮,eachwithvelocity 40 ms^(-1).Thesecondballreaches 40 mhigherthanthefirstball.F∈dtheir∈÷eithts,Take g= 10 m//s.
View SolutionA ball is thown at angle θ and another ball is thrown at angle (90∘−θ) with the horizontal direction from the same point each with speeds of 40 m/s. The second ball reaches 50 m higher than the first ball. Find the individual heights. g=10m/s2
View Solution
Knowledge Check
A ball is thrown at an angle θ with the horizontal and the range is maximum. The value of tanθ is:-
A1B√3C1√3D2On an inclined plane of inclination 30∘, a ball is thrown at angle of 60∘ with the horizontal from the foot of the incline with a velocity of 10√3ms−1. Then the ball will hit the inclined plane in
A1sB2sC2√3sD4√3sA ball is thrown up with a certain velocity at angle θ to the horizontal. The kinetic energy varies with height h of the particle as:
A B
B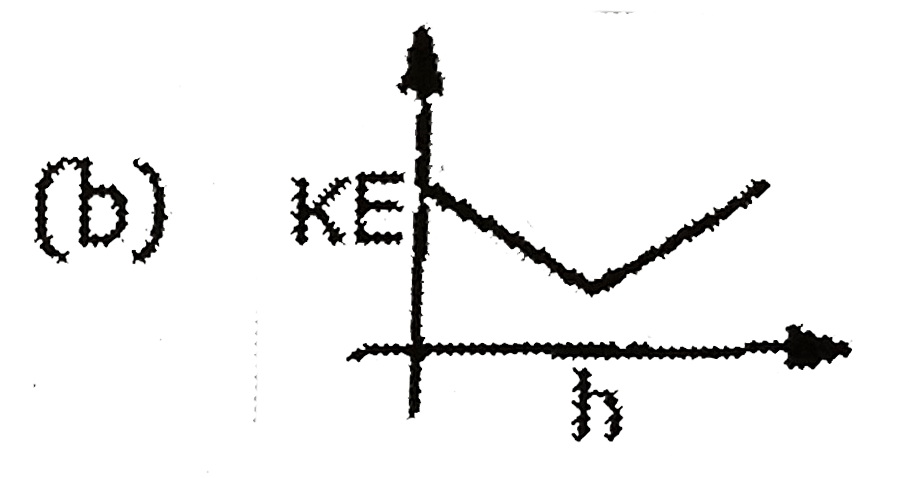 C
C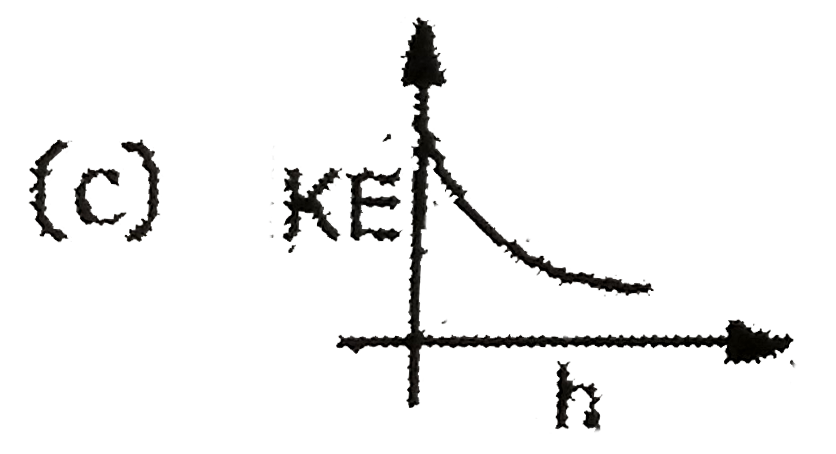 D
D
One body is thrown at an angke θ with the horizontal and another similar body is thrown at an angle θ with the vertical direction from the same point with same velocity40ms−1. The second body reaches 50 metrea higher than the first body. Deteramine their individual heights. Take g=10ms−2.
View SolutionTwo balls A and B are thrown from the same point with similar velocity of 19.6ms , at angle θ and 90∘−θ with the horizontal , respectively . Ball B reaches 10 m higher than ball A. Find the individual maximum higher reached by ball A and B .
View SolutionA ball of mass M is thrown vertically upwards. Another ball of mass 2M is thrown at an angle θ with the vertical. Both of them stay in air for the same period of time. The heights attained by the two are in the ratio
View SolutionA ball of mass M is thrown vertically upwards. Another ball of mass 2M is thrown at an angle θ with the vertical. Both of them stay in air for the same period of time. The heights attained by the two are in the ratio
View SolutionA ball is thrown up with a certain velocity at an angle θ to the horizontal. The graph between kinetic energy and horizontal displacement is given by:
View Solution
