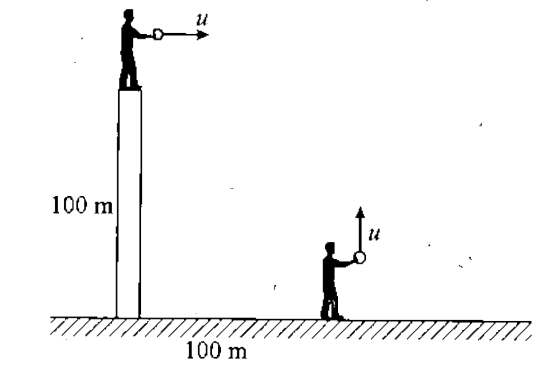
A shot of mass m is fired horizontally from the top of a tower of height 100m, with a velocity μ=50 m/s as shown in figure −4.154 At a distance 100m from the foot the tower a child throws a ball or same m in vertial direction with the same velocity μ=50 m/s. He throws the ball such that the ball will colides with the shot in its path and merged with it makeing an object of mass 2m. Find the distance form the child, where this object lands on ground.
A shot of mass m is fired horizontally from the top of a tower of height 100m, with a velocity mu=50 m/s as shown in figure -4.154 At a distance 100m from the foot the tower a child throws a ball or same m in vertial direction with the same velocity mu=50 m/s. He throws the ball such that the ball will colides with the shot in its path and merged with it makeing an object of mass 2m. Find the distance form the child, where this object lands on ground.

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:[123.6mfrom the child]

Updated on:21/07/2023
|
Answer

Step by step video, text & image solution for A shot of mass m is fired horizontally from the top of a tower of height 100m, with a velocity mu=50 m/s as shown in figure -4.154 At a distance 100m from the foot the tower a child throws a ball or same m in vertial direction with the same velocity mu=50 m/s. He throws the ball such that the ball will colides with the shot in its path and merged with it makeing an object of mass 2m. Find the distance form the child, where this object lands on ground. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023
Class 11PHYSICSCENTRE OF MASS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • A particle of mass 50 g is projected horizontally from the top of a tower of height 50 m with a velocity 20m/s. If g=10m/s2, then find the :-

    AVelocity at the instant t=2 s
    BPosition at the instant t=3 s
    CVelocity just before hitting the ground
    DChange in velocity in 2 s

  • A cannon ball is projected horizontally from the top of a tower 980 meters high, with a velocity of 50m/sec. The time of flight is

    A2 sec
    B√2 sec
    C1sec
    D1/2 sec

  • The angle of elevation of the top of a tower of height 100√3m from a point at a distance of 100 m from the foot of the tower on a horizontal plane is

    A60∘
    B90∘
    C120∘
    D180∘