A plank with a body of mass m places on it starts moving straight up according to the law y=a(1−cosωtt), whee y is the displacement from the initial position, ω=11s−1. Find :
(a) the time dependence of the force that the body exerts on the plank if a=4.0cm, plot this dependence,
(b) the minimum amplitude of oscillation of the plank at which the body starts falling behind the plank,
(c) the amplitude of oscillation of the plank at which the body springs up to a height h=50cm relative to the initial position (at the moment t=0).
The correct Answer is:gΩ2(Ω.√2hg−1)
Step by step video, text & image solution for A plank with a body of mass m places on it starts moving straight up according to the law y=a(1-cos omegat t ), whee y is the displacement from the initial position, omega =11 s^(-1). Find : (a) the time dependence of the force that the body exerts on the plank if a=4.0cm, plot this dependence, (b) the minimum amplitude of oscillation of the plank at which the body starts falling behind the plank, (c) the amplitude of oscillation of the plank at which the body springs up to a height h=50cm relative to the initial position (at the moment t=0). by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SIMPLE HARMONIC MOTIONBOOK - NN GHOSHCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Eexercies
39 Videos
- SIMPLE AND COMPOUND PENDULUMBOOK - NN GHOSHCHAPTER - SIMPLE AND COMPOUND PENDULUMEXERCISE - Exercise
24 Videos
- SPECIFIC HEAT CAPACITY OF GASESBOOK - NN GHOSHCHAPTER - SPECIFIC HEAT CAPACITY OF GASESEXERCISE - All Questions
17 Videos
Similar Questions
A plank with a body of mass m placed on it starts moving straight up according to the law y=a(1−cosωt), where y is the displacement from the initial position, ω=11rad/s. Find
(a) The time independence of the force that the body exerts on the plank.
(b) The minimum amplitude of oscillations of the plank at which the body starts falling behind the plank.
View SolutionA plank with a bar placed on it performs horizontal harmonic oscillations with amplitude a=10cm. Find the coefficient of friction between the bar and the plank if the former starts sliding along the plank when the amplitude of oscillation of the plank becomes less than T=1.0s.
View Solution
Knowledge Check
A man of mass m is standing on a plank of equal mass m resting on a smooth horizontal surface. The man starts moving on the plank with speed u relative to the plank. The speed of the man relative to the ground is
 A2uBu2CzeroDu4
A2uBu2CzeroDu4A block is kept on a rough horizontal plank. The coefficient of friction between the block and the plank is 12. The plank is undergoing SHM of angular frequency 10 rad/s. The maximum amplitude of plank in which the block does not slip over the plank is (g= 10 m/s2)
A4cmB5cmC10 cmD16 cmA man of mass M stands at one end of a plank of length L Which lies at rest on a frictionless surface . The man walks to the other end of the plank. If the mass of the plank is 3M, the distance that the man moves relative to the ground is
AL4B3L4C2L3DL3
A body of mass 1kg is moving according to the law x(t)=(5t+4t2+6t3)m . The force acting on the body at time t=2s is
View SolutionA plank of mass m1 with a bar of mass m2 placed on it lies on a smooth horizontal plane. A horizontal force growing with time t as F′=at (a is constant) is applied to the bar. Find how the accelerations of the plank w1 and of the bar w2 depend on t, if the coefficient of friction between the plank and the bar is equal to k. Draw the approximate plots of these dependences.
View SolutionA wooden plank of mass 20 kg is resting on a smooth horizontal floor. A man of mass 60 kg starts moving from one end of the plank to the other end. The length of the plank is 10 m. Find the displacement of the plank over the floor when the man reaches the other end of the plank.
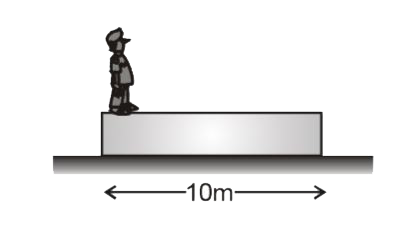
View SolutionA wooden plank of mass 20kg is resting on a smooth horizontal floor. A man of mass 60kg starts moving from one end of the plank to the other end. The length of the plank is 10m. Find the displacement of the plank over the floor when the man reaches the other end of the plank.

View SolutionA particle of mass m is projected at an angle of 60∘ with a velocity of 20m/s relative to the ground from a plank of same mass m which is placed on smooth surface Initially Plank was at rest The minimum length of the plank for which the ball will fall on the plank itself is (g=10m/s2)

View Solution
