Determine the luminosity of a surface whose luminance depends on direction as L=L0cosθ, where θ is the angle between the radiation direction and the normal to the surface.
The correct Answer is:B32πL0
Step by step video, text & image solution for Determine the luminosity of a surface whose luminance depends on direction as L = L_(0) cos theta, where theta is the angle between the radiation direction and the normal to the surface. by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A point source is suspended at a hight h=1.0m over the centre of a round table of radius R=1.0m. The luminous intensity I of the source depends on direction so that illuminance at all points of the table is the same. Find the function I(θ), where θ is the angle between the radiation direction and the vertical, as well as the luminous flux reaching the table if I(0)=I0=100cd.
View SolutionShow that the electric field at the surface of a charged conductor is given by →E=σε0ˆn , where σ is the surface charge density and ˆn is a unit vector normal to the surface in the outward direction .
View Solution
Knowledge Check
A parallel beam of monochromatic light is incident on the surface of water having refractive index 4/3. The direction of the incidence beam bisects the angle between the normal to the water surface and the water surface. The angles of incidence and refraction are
Ai=30∘,r=20∘Bi=45∘,r=20∘Ci=45∘,r=32∘Di=60∘,r=40∘Assertion : If θ is the angle between the planes of transmission of two analysers then the intensity of emergent light is proportional to cos∘θ.
Reason : In the propagation of electromagnetic waves, the angle between the direction of propogation and plane of polarisation is π2.AAssertion is True, Reason is True, Reason is a correct explanation for AssertionBAssertion is True, Reason is True, Reason is not a correct explanation for AssertionCAssertion is True, Reasonis FalseDAssertion is False, Reason is TrueIf l1,m1,n1 and l2,m2,n2 are the directional cosines of two vectors and θ is the angle between them, then their value of cosθ is
Al1l2+m1m2+n1n2Bl1m1+m1n1+n1l1Cl2m2+m2n2+n2l2Dm1l2+l2m2+n1m2
A point source is suspended at a height h over the centre of a round. Table The lumious intensity. P of the source depends on the direction θ with the normal function Pθ in terms of θ, assuming thatP(0)=P0
View SolutionProve that the equation of the normal to x23+y23=a23 is ycosθ−xsinθ=acos2θ, where θ is the angle which the normal makes with the axis of x.
View SolutionA semicircular wire is uniformly charged with linear charge density dependent on the angle θ from y-direction as λ=λ0|sinθ|, where λ0 is a constant. The electric field intensity at the centre of the arc is
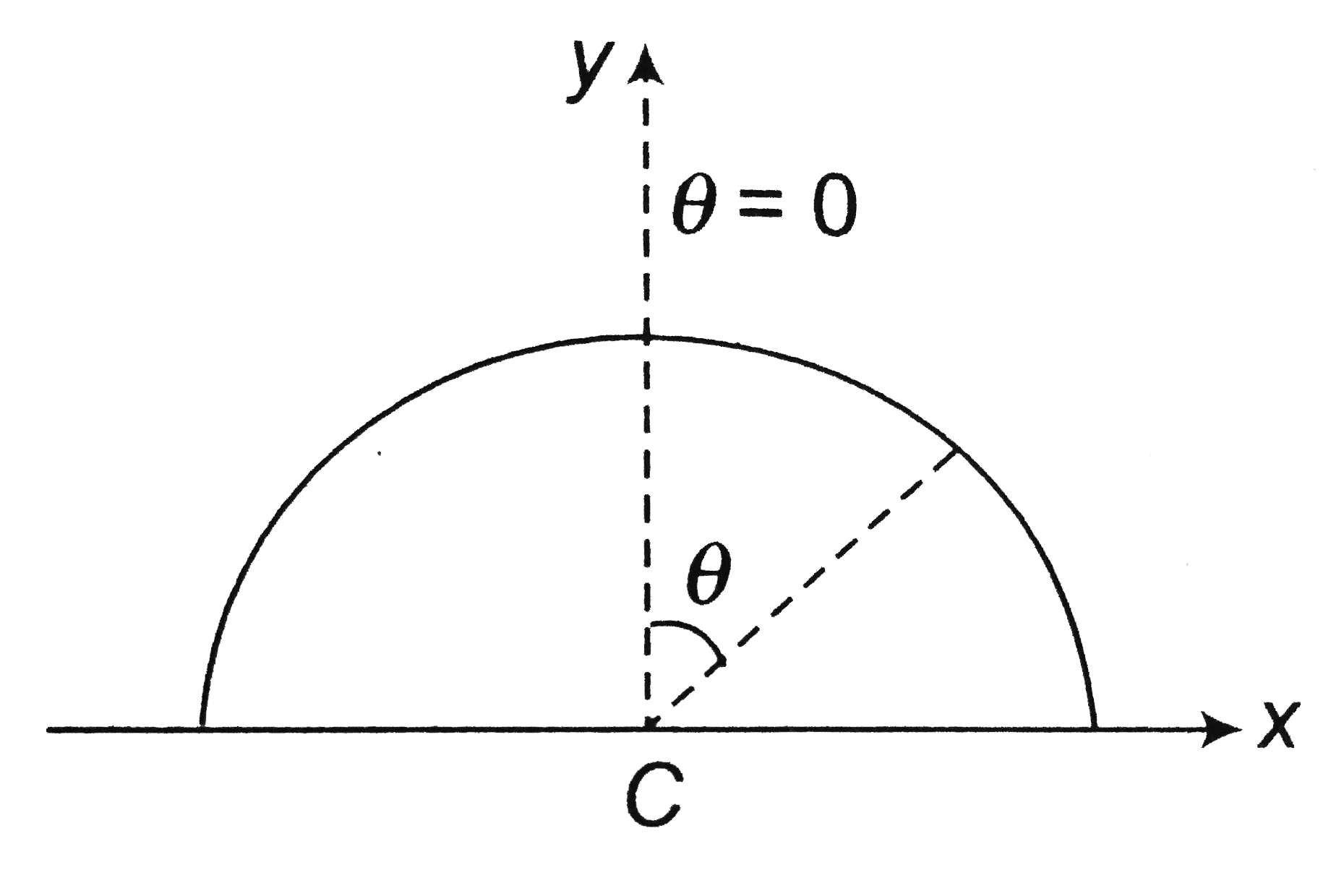
View SolutionA beam of monochromatic light of wavelength λ falls normally on a diffraction grating of line spacing d. If θ is the angle between the second-order diffracted beam and the direction of the incident light, what is the value of sinθ ?
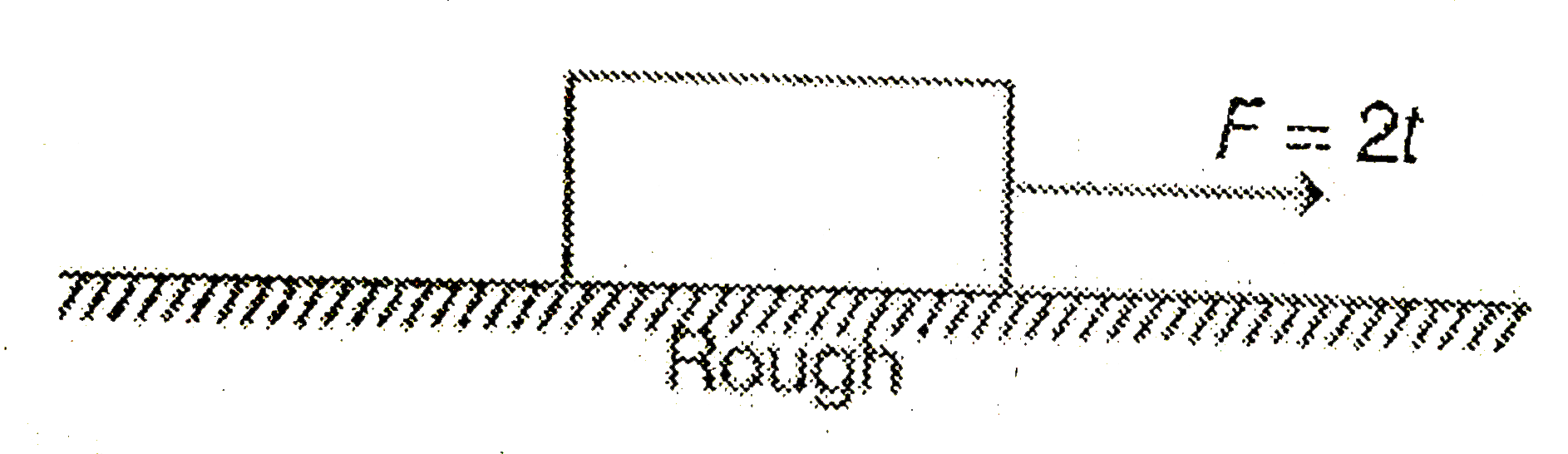
View SolutionA time varying force is applied on a block placed over a rough surface as shown in figure. Let θ be the angle between net contact force on the block and the normal reaction, then with time, θ will
View Solution
