A body cools in a surrounding which is at constant temperature of θ0. Assume that it obeys Newton's law of colling. Its temperature θ is plotted against time t. Tangents are drawn to the curve at the points P(θ=θt) and Q(θ=θ2). These tangents meet the time axis at angles of ϕ2 and ϕ1, as shown
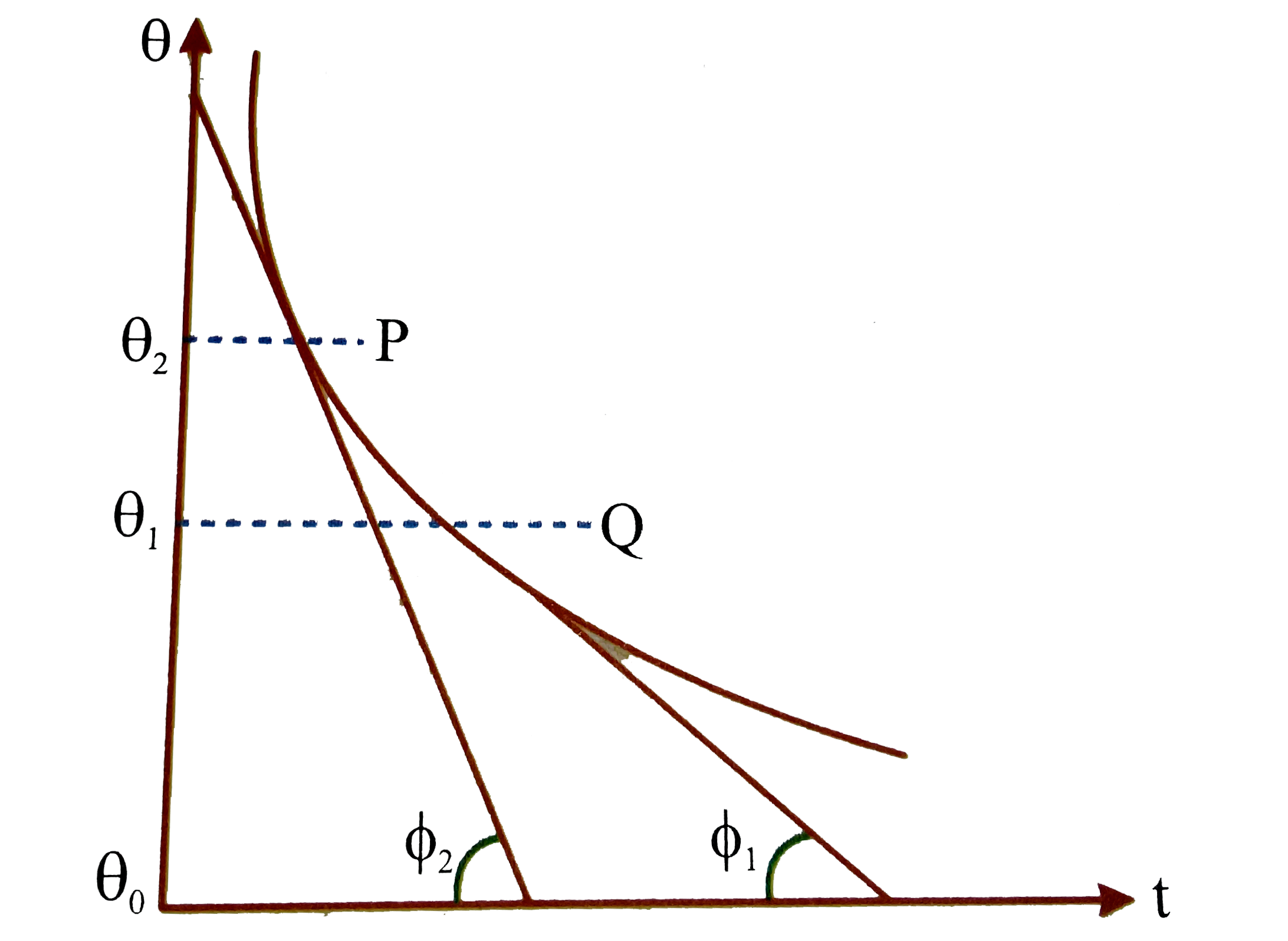
tanϕtanϕ=θ1−θ0θ2−θ0
tanϕtanϕ=θ2−θ0θ1−θ0
tanϕtanϕ=θ1θ2
tanϕtanϕ=θ2θ1
Step by step video & image solution for A body cools in a surrounding which is at constant temperature of theta_(0). Assume that it obeys Newton's law of colling. Its temperature theta is plotted against time t. Tangents are drawn to the curve at the points P(theta=theta_(t)) and Q(theta=theta_(2)). These tangents meet the time axis at angles of phi_(2) and phi_(1), as shown by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Two tangents are drawn from the point (−2,−1) to the parabola y2=4x .If θ is the angle between these tangents,then the value of tanθ is
View SolutionA body with an initial temperature θ1 is allowed to cool in a surrounding which is at a constant temperature of θ0(θ<θ1) Assume that Newton's law of cooling is obeyed Let k= constant The temperature of the body after time t is best experssed by .
View Solution
Knowledge Check
A body cools in a surrounding which is at a constant temperature of θ0 Assume that it obeys Newton's law of cooling Its temperature θ is plotted against time t Tangents are drawn to the curve at the points P(θ=θ1) and Q(θ=θ2) These tangents meet the time axis at angle of ϕ2 andϕ1 as shown
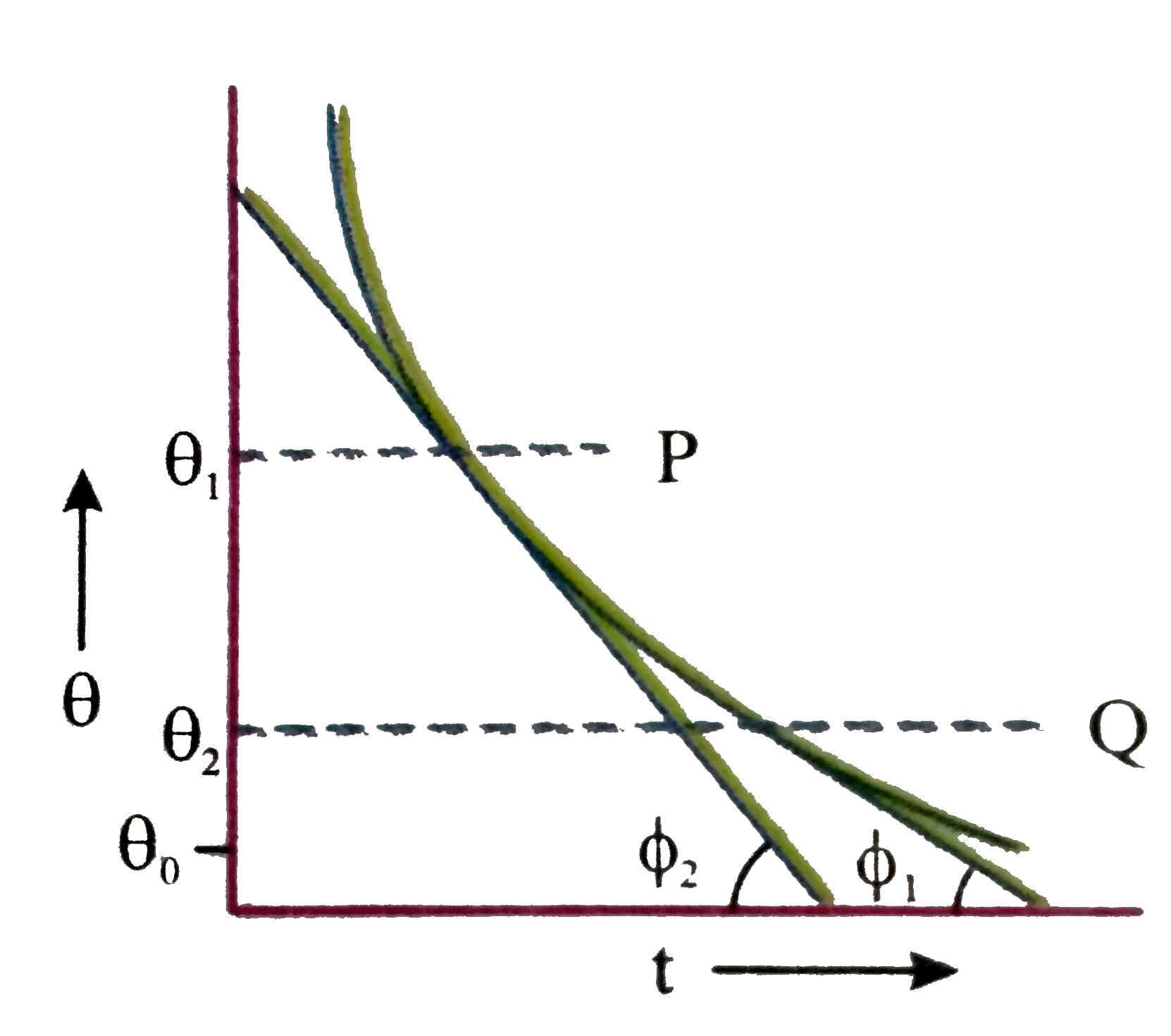 .Atanϕ2tanϕ1=θ1−θ0θ2−θ0Btanϕ2tanϕ1=θ1−θ0θ2−θ0Ctanϕ2tanϕ1=θ1−θ0θ2−θ0Dtanϕ2tanϕ1=θ1−θ0θ2−θ0
.Atanϕ2tanϕ1=θ1−θ0θ2−θ0Btanϕ2tanϕ1=θ1−θ0θ2−θ0Ctanϕ2tanϕ1=θ1−θ0θ2−θ0Dtanϕ2tanϕ1=θ1−θ0θ2−θ0A body obeying Newton's law of cooling cools in a surrounding which is at a constant temperature Its temperature θ is plotted against time There are two points on the curve with temperatures θ2 and θ1(θ2>θ1) such that tangents on these points make angles of 2ϕ and half of its with time axis respectively Find the temperature of the surrounding .
Aθ1cos2ϕ−θ2(1+cos2ϕ)Bθ1(1+cos2ϕ)−θ2cos2ϕC(θ2−θ1)cos2ϕD2θ1+θ2(1+tan2ϕ)(1−tan2ϕ)Tangents are drawn to ellipse x2a2+y2b2=1 at points P(θ1) and Q(θ2) then the point of intersection of these tangents is
A⎛⎜⎝acosθ1+θ22cosθ1−θ22,b sinθ1+θ22cosθ1−θ22⎞⎟⎠B⎛⎜⎝acosθ1−θ22cosθ1+θ22,b sinθ1−θ22cosθ1+θ22⎞⎟⎠C⎛⎜⎝a sinθ1+θ22sinθ1−θ22,b cosθ1+θ22sinθ1−θ22⎞⎟⎠Dnone
Two tangents are drawn from the point (−2,–1) to the parabola y2=4x. If θ is the angle between these tangents then tan θ =
View SolutionIn Newton's law of cooling experiment, we plot a graph of (dθdt) against the temperature difference (θ−θ0). The graph is
View SolutionThe angle made by the tangent to the curve x=a(θ+sinθcosθ),y=a(1+sinθ)2 wutg X-axis is
View SolutionThe equation of tangent to the curve x=a(θ+sinθ),y=a(1+cosθ) at θ=π2 is
View SolutionThe tangents to the curve x=a(θ−sinθ),y=a(1+cosθ) at the points θ=(2k+1)π,k∈Z are parallel to :
View Solution
