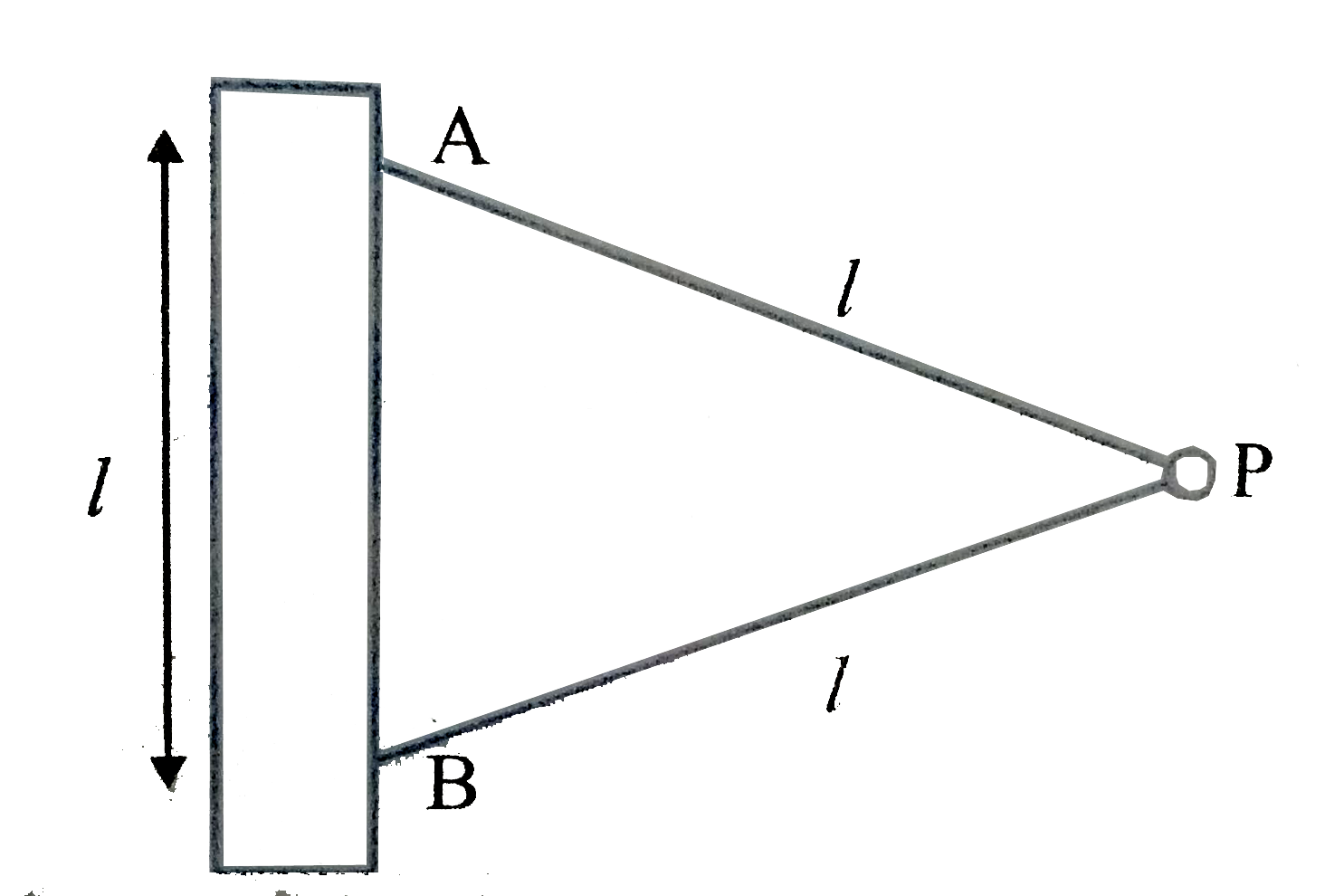
The particle P of mass m is attached to two light, rigid rods AP and BP of length l each. A and B are hings on a fixed vertical axis. The system APB can rotate freely about this axis. The angle ABP = the angle BAP=θ. The tensions in AP and BP are T1 and T2 respectively.
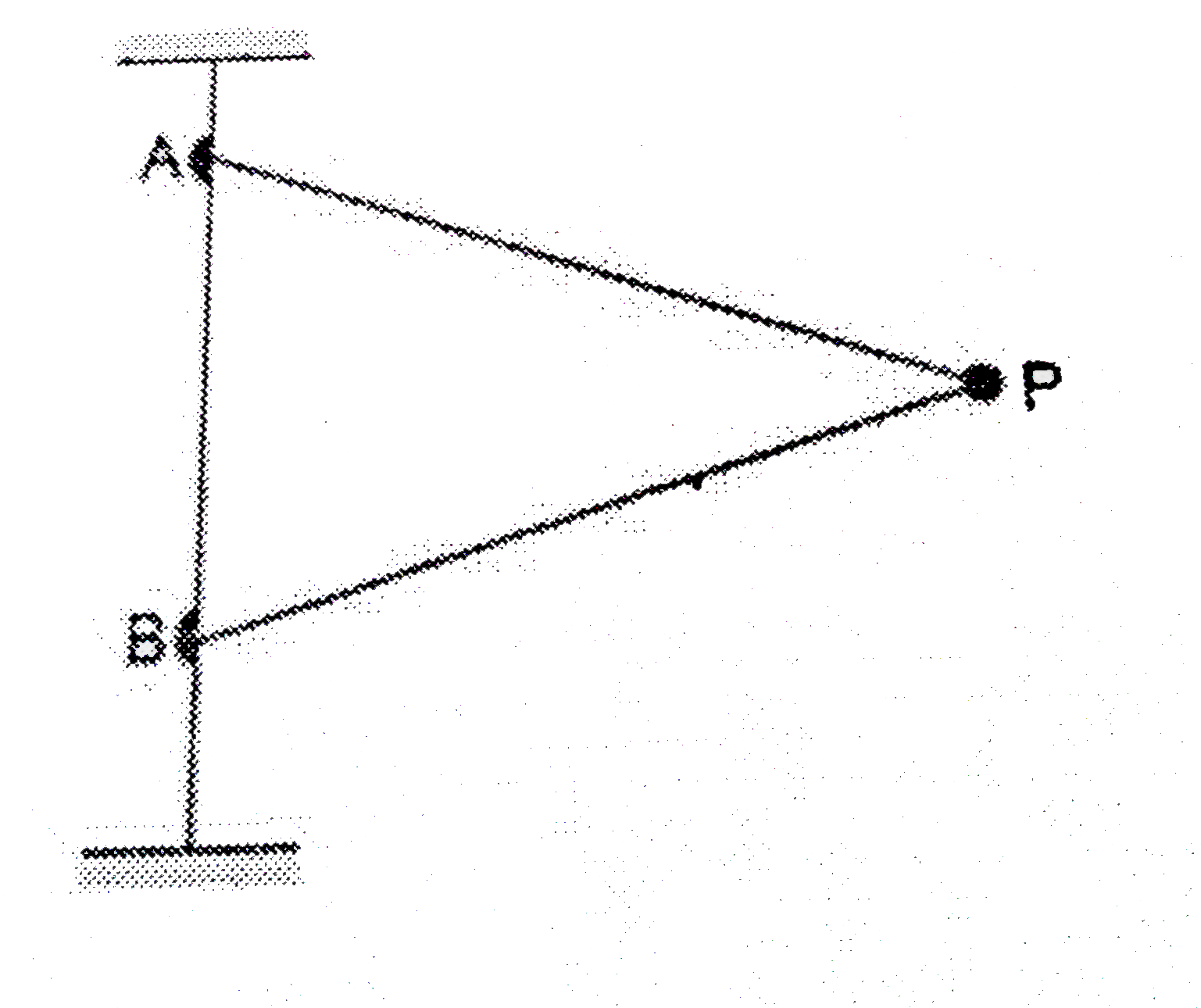
When the system is at rest, which of the following is not correct?
At P, the direction of T1 is from P to A.
At P, the direction of T2 is from P to B.
The rods AP and BP together exert a net force and net torque on AB.
T1=T2.
The correct Answer is:D
Step by step video, text & image solution for The particle P of mass m is attached to two light, rigid rods AP and BP of length l each. A and B are hings on a fixed vertical axis. The system APB can rotate freely about this axis. The angle ABP = the angle BAP = theta. The tensions in AP and BP are T_(1) and T_(2) respectively. When the system is at rest, which of the following is not correct? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
If A and B are (1, 4) and (5, 2) respectively, find the coordinates of P when AP/BP=3/4 .
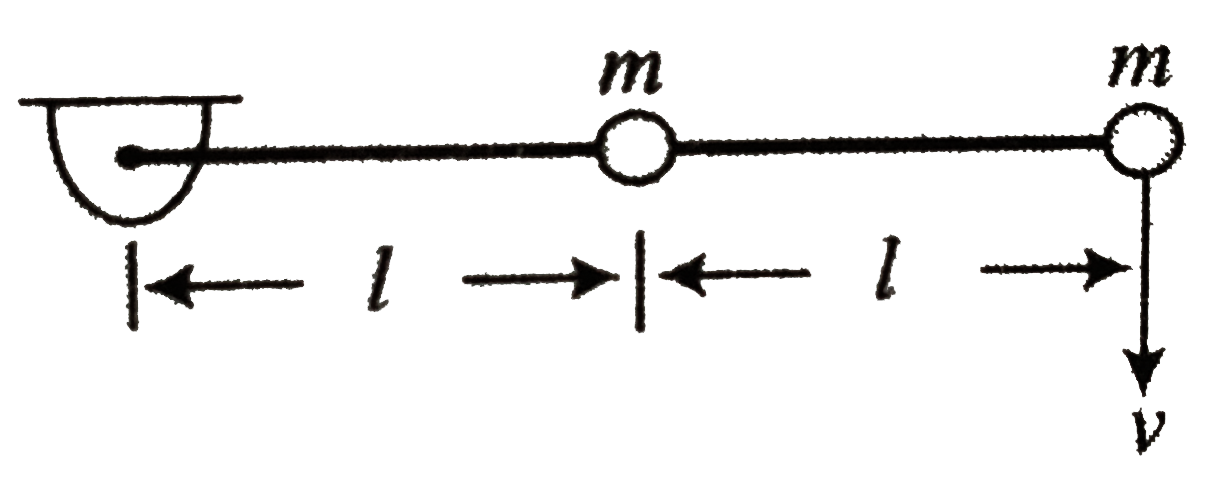
View SolutionTwo beads each of mass m are welded at the ends of two light rigid rods each of length l. if the pivots are smooth, find the ratio of translational and rotational kinetic energy of system.
View Solution
Knowledge Check
The particle P of mass m is attached to two light, rigid rods AP and BP of length l each. A and B are hings on a fixed vertical axis. The system APB can rotate freely about this axis. The angle ABP = the angle BAP=θ. The tensions in AP and BP are T1 and T2 respectively.
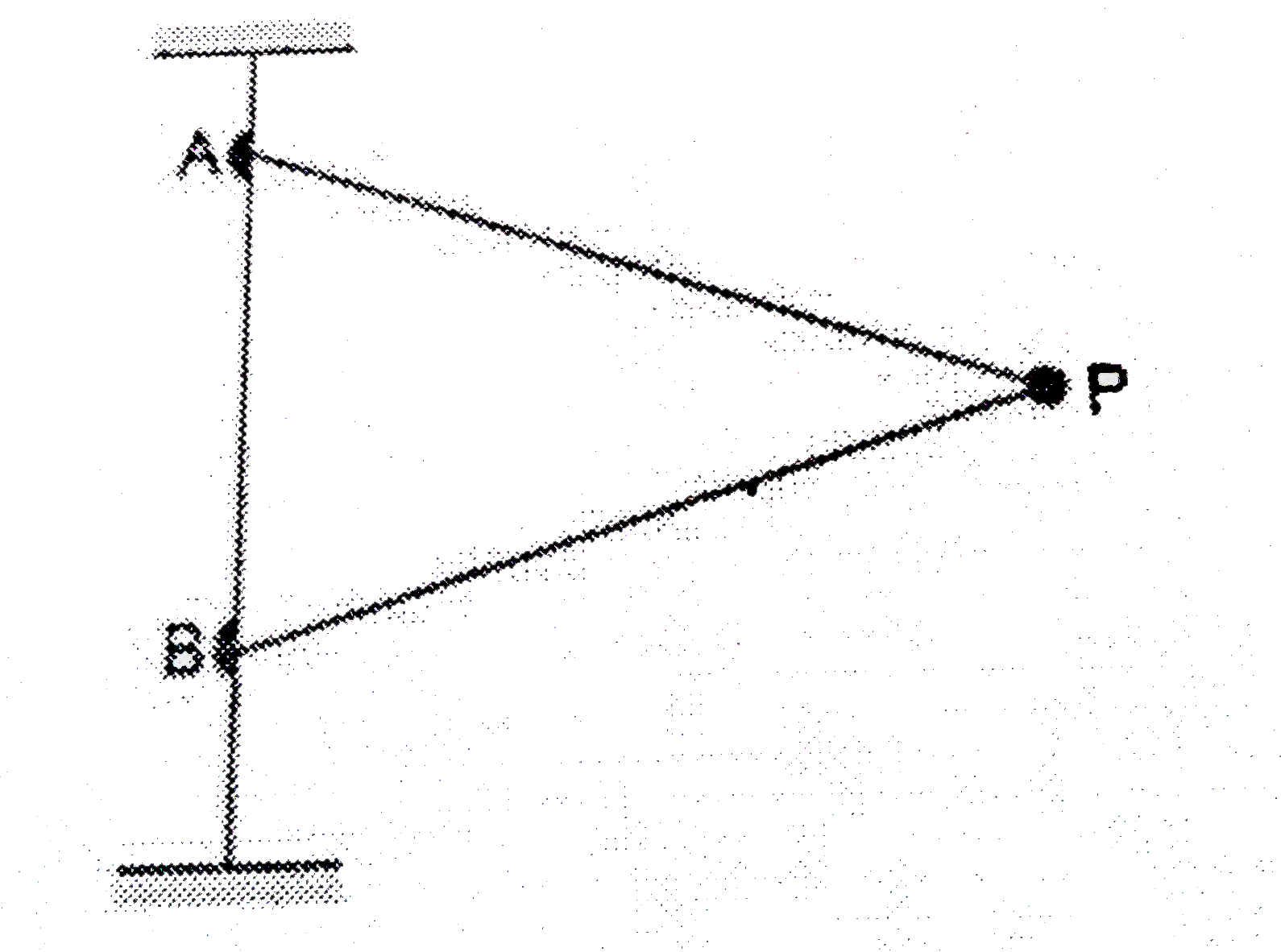
When the system is made to rotate about AB with angular velocity ω, which of the following is not correct?AT1 will always be greater than T2.BT1−T2=mω2t for small values of ω.CT2 will become zero for ω2=g/lcosθ.DThe direction of T2 will always be from P to B.The particle P of mass m is attached to two light, rigid rods AP and BP of length l each. A and B are hings on a fixed vertical axis. The system APB can rotate freely about this axis. The angle ABP = the angle BAP=θ. The tensions in AP and BP are T1 and T2 respectively.
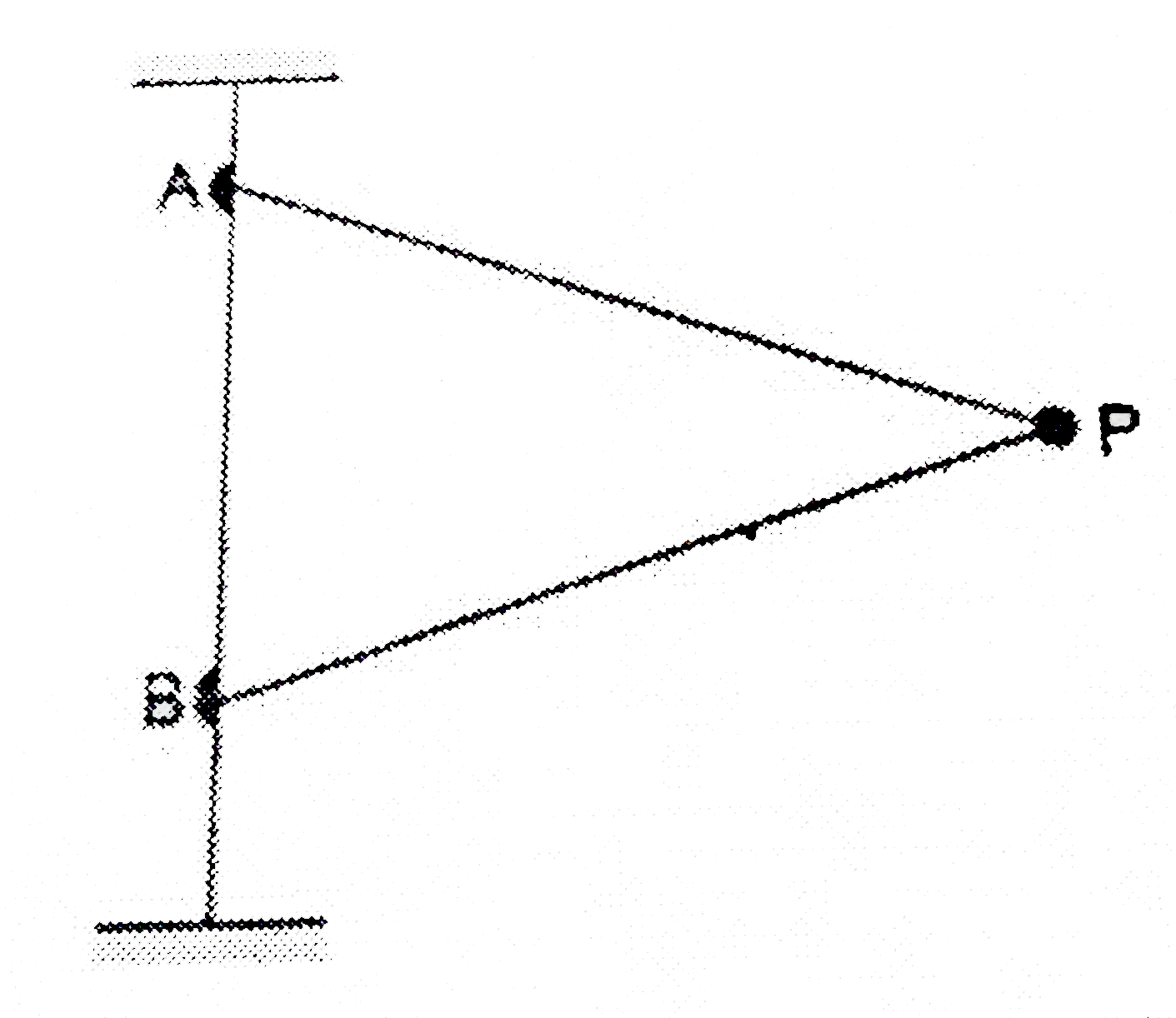
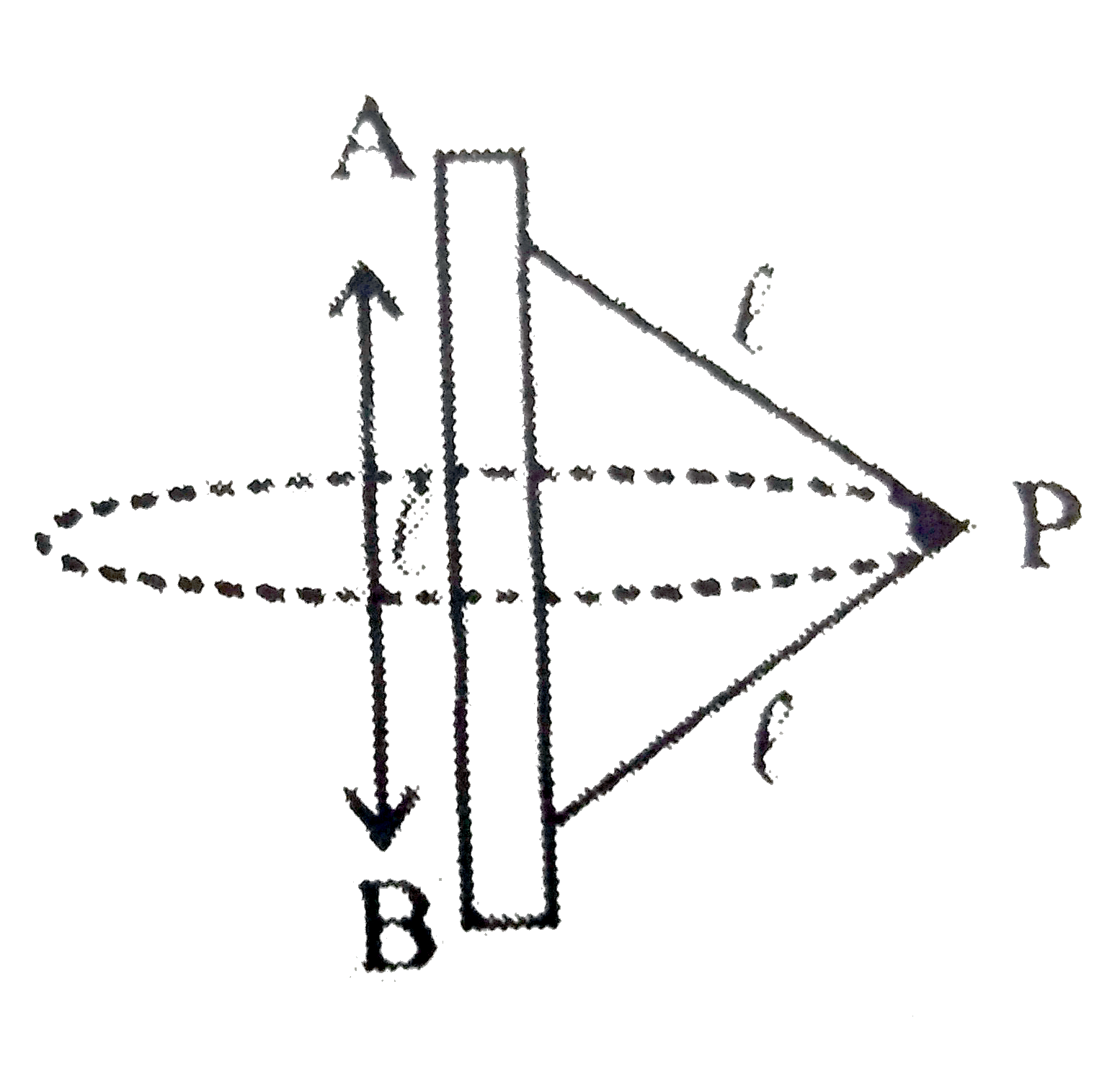
The system is now rotated by 90∘ so that must be imparted to P, normal to the plane of the figure, such that it moves in a complete circular path in a vertical plane with AB as the axis?A2√glsinθB2√glcosθC(5/2)√glsinθDNone of theseA particle P of mass m is attached to a vertical axis by two strings AP and BP of legth l each. The separation AB=l, rotates around the axis with an angular velocity ω. The tension in the two string are T1 and T2. Then
 AT1=T2BT1+T2=mω2lCT1−T2=2mgDBP will remains taut only if ω≥√2g/l
AT1=T2BT1+T2=mω2lCT1−T2=2mgDBP will remains taut only if ω≥√2g/l
A particle of mass m is attached to an end of a light rigid rod of length a. The other end of the rod is fixed, so that the rod can rotate freely in vertical plane about its fixed end. The mass m is given a horizontal velocity u at the lowest point. (a) Prove that when the radius to the mass makes an angle θ with the upward vertical the horizontal component of the acceleration of the mass (measured in direction of u) is [g(2+3cosθ)−u2/a]sinθ (b) If 4ag ltu2 lt5ag, show that there are four points at which horizontal component of acceleration is zero. locate the points.
View SolutionA particle P of mass m is attached to a vertical axis by two strings AP and BP of length l each. The separation AB=l. P rotates around the axis with an angular velocity ω. The tensions in the two strings are T1andT2
View SolutionA particle of mass m is fixed to one end of a light rigid rod of length l and rotated in a vertical circular path about its other end. The minimum speed of the particle at its highest point must be
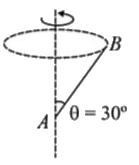
View SolutionA rod AB of mass m and length l is rotating about a vertical axis as shown. It's moment of inertia about this axis is :
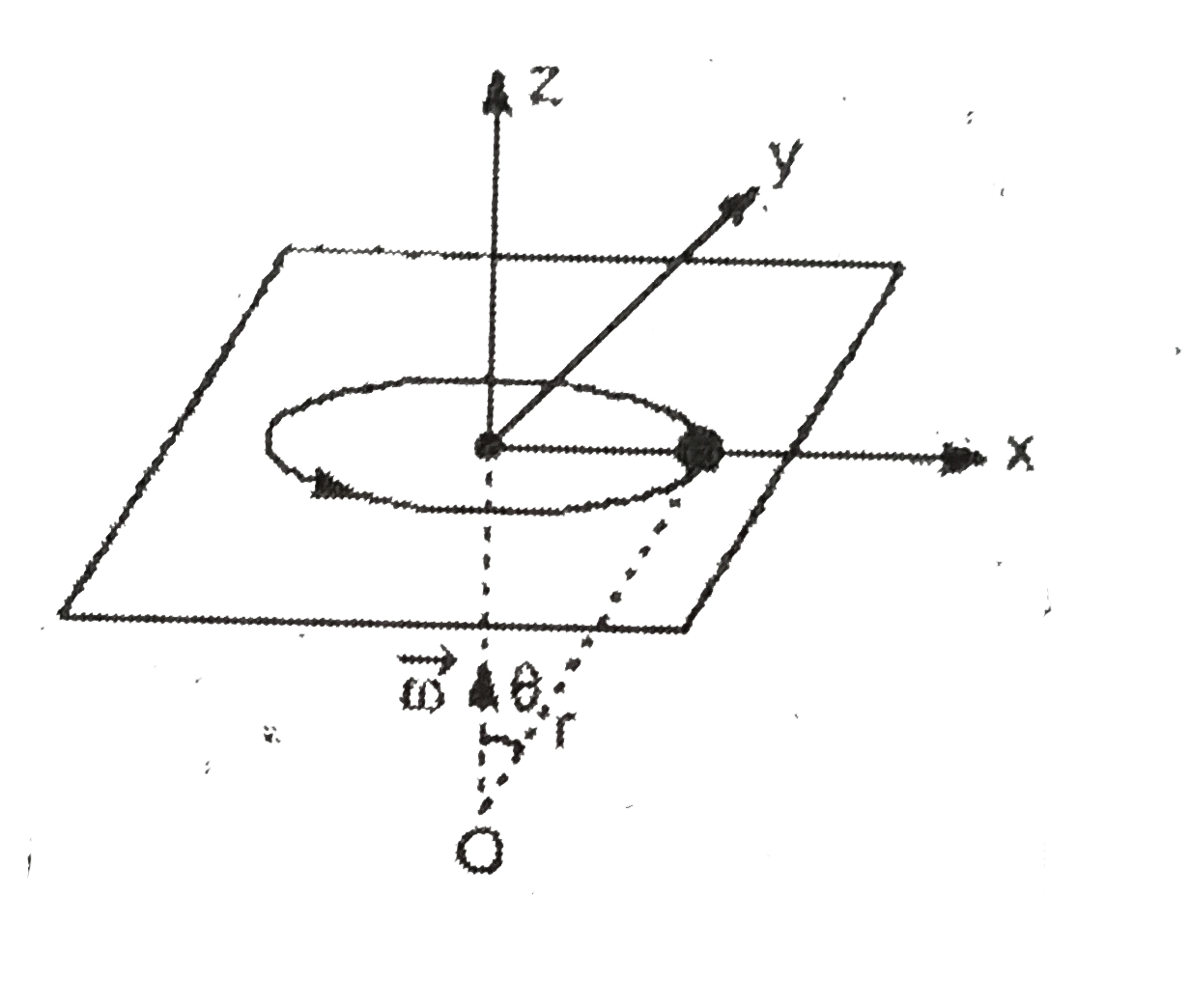
View SolutionA mass m is attached to a rigid rod of negligible mass as shown in figure. The system is pivoted at point O and rotates about the indicated z-axis with angular velocity →ω, maintaining a fixed angle θ with the axis.
View Solution
