In Young's experiment, find the distance between two slits that results in the third minimum for 420nm violet light at an angle 30∘
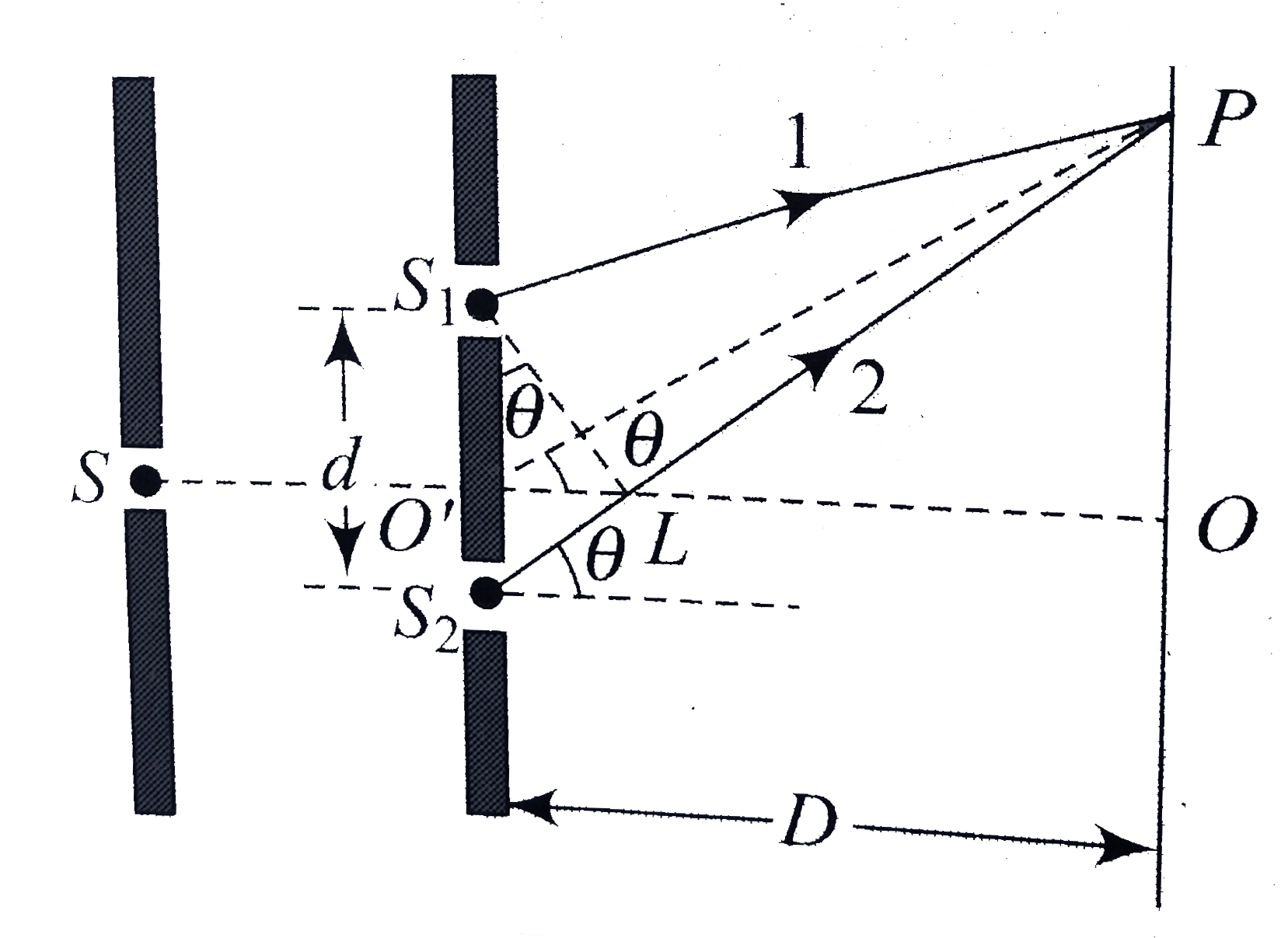 .
.
Step by step video, text & image solution for In Young's experiment, find the distance between two slits that results in the third minimum for 420 nm violet light at an angle 30^(@) . by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- WAVE OPTICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - WAVE OPTICSEXERCISE - Practice Exercise 6.1
1 Videos
- WAVE OPTICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - WAVE OPTICSEXERCISE - Practice Exercise
25 Videos
- THERMODYNAMICS LAWS & SPECIFIC HEATS OF GASES BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - THERMODYNAMICS LAWS & SPECIFIC HEATS OF GASES EXERCISE - Practice Exercise 3.4
11 Videos
- WAVES BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - WAVES EXERCISE - Unsolved Numerical Problems for Preparation of NSE, INPhO & IPhO
135 Videos
Similar Questions
If in Young's experiment the distance between the two slits is doubled and the distance of the screen and slite is halved the fringewidth
View SolutionIn Young's experiment, the dustance between two slit is 08 mm and the distance of the screen from the slits is 80cm if the fringe width is 0.6mm , thent eh wavelenghts of light usedis
View Solution
Knowledge Check
In Young's double slit experiment, the distance between two slits is made three times then the fringe width will becomes
A9 timesB1/9 timesC3 timesD1/3 timesIn Young’s double slit experiment, the distance between the two slits is made half, then the fringe width will become
AHalfBDoubleCOne fourthDUnchangedIn Young's double slit experiment, if the distance between two slits is equal to the wavelength of used light. Then the maximum number of bright firnges obtained on the screen will be
AInfiniteB3C4D5
In double slit experiment , the distance between two slits is 0.6mm and these are illuminated with light of wavelength 4800Å. The angular width of dark fringe on the screen at a distance 120 cm from slits will be
View SolutionIn Young's double-slit experiment the distance between the slits is 1 mm and that between slit and screen is 1m. If 10th bright fringe is 5 mm away from the central bright fringe, then wavelength of light used will be
View SolutionIn Young's double slit experiment, a third slit is made in between the double sits. Then
View SolutionIn Young's experiment the distance between two slits is d3 and the distance between the screen and the slits is 3D. The number of fringes in 13 metre on the screen, formed by monochromatic light of wavelength 3λ, will be:
View SolutionIn Young's experiment the distance between the slits is reduced to half and the distance between the slit and screen is doubled then the fringe width
View Solution
