The average of the test scores of a group of x students is 76 and the average of the test scores of a group of y students is 90. When the scores of the two groups of students are combined, the average test score is 85. What is the value of of xy?
47
59
23
74
The correct Answer is:B
To solve the problem step by step, we will follow the reasoning provided in the video transcript.
Step 1: Define the sums of scores
Let the sum of the test scores of the group of x students be A and the sum of the test scores of the group of y students be B.
Step 2: Set up equations for the averages
From the information given:
- The average score of x students is 76, so we can write:
A=76x(Equation 1)
- The average score of y students is 90, so we can write:
B=90y(Equation 2)
Step 3: Set up the equation for the combined average
When the scores of both groups are combined, the average score is 85. Therefore, we can express this as:
A+Bx+y=85
Step 4: Substitute A and B into the combined average equation
Substituting the values of A and B from Equations 1 and 2 into the combined average equation gives:
76x+90yx+y=85
Step 5: Cross-multiply to eliminate the fraction
Cross-multiplying results in:
76x+90y=85(x+y)
Step 6: Expand the right-hand side
Expanding the right-hand side gives:
76x+90y=85x+85y
Step 7: Rearrange the equation
Rearranging the equation to isolate terms involving x and y:
76x+90y−85x−85y=0
This simplifies to:
−9x+5y=0
or
9x=5y
Step 8: Solve for the ratio xy
To find the ratio xy, we can rearrange the equation:
xy=59
Final Answer
Thus, the value of xy is:
xy=59
---
Let the sum of the test scores of the group of x students be A and the sum of the test scores of the group of y students be B.
From the information given:
- The average score of x students is 76, so we can write:
A=76x(Equation 1)
- The average score of y students is 90, so we can write:
B=90y(Equation 2)
When the scores of both groups are combined, the average score is 85. Therefore, we can express this as:
A+Bx+y=85
Substituting the values of A and B from Equations 1 and 2 into the combined average equation gives:
76x+90yx+y=85
Cross-multiplying results in:
76x+90y=85(x+y)
Expanding the right-hand side gives:
76x+90y=85x+85y
Rearranging the equation to isolate terms involving x and y:
76x+90y−85x−85y=0
This simplifies to:
−9x+5y=0
or
9x=5y
To find the ratio xy, we can rearrange the equation:
xy=59
Thus, the value of xy is:
xy=59
Topper's Solved these Questions
- PROBLEM SOLVING AND DATA ANALYSISBOOK - ENGLISH SATCHAPTER - PROBLEM SOLVING AND DATA ANALYSISEXERCISE - Grib-In
40 Videos
- PROBLEM SOLVING AND DATA ANALYSISBOOK - ENGLISH SATCHAPTER - PROBLEM SOLVING AND DATA ANALYSISEXERCISE - Grib-In
40 Videos
- PROBLEM SOLVING AND DATA ANALYSISBOOK - ENGLISH SATCHAPTER - PROBLEM SOLVING AND DATA ANALYSISEXERCISE - EXERICSE
80 Videos
- PROBLEMS IN CONTEXT BOOK - ENGLISH SATCHAPTER - PROBLEMS IN CONTEXT EXERCISE - PRACTICE TEST
10 Videos
Similar Questions
Calculate the mean of the scores of 20 students in a mathematics test
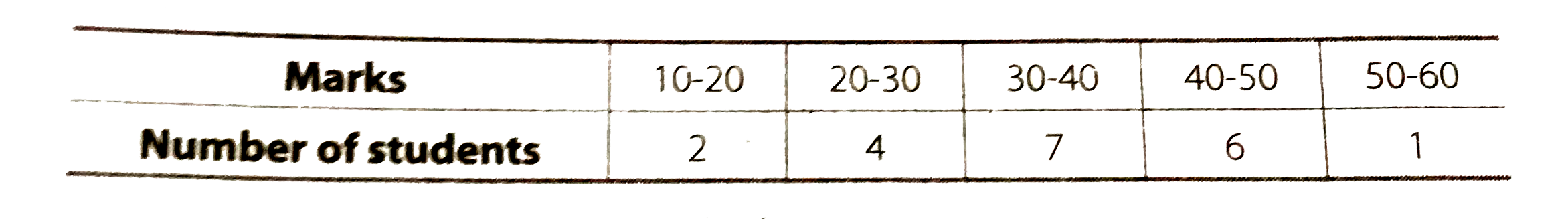
View SolutionA group of students takes a test and the average score is 72. One more student takes the test and receives a score of 88 increasing the average score of the group to 76. How many students were in the initial group ?
View Solution
Knowledge Check
The highest and lowest test scores of five students in Mr . Canyon's science class are listed below . Which students had the greatest range of scores ?
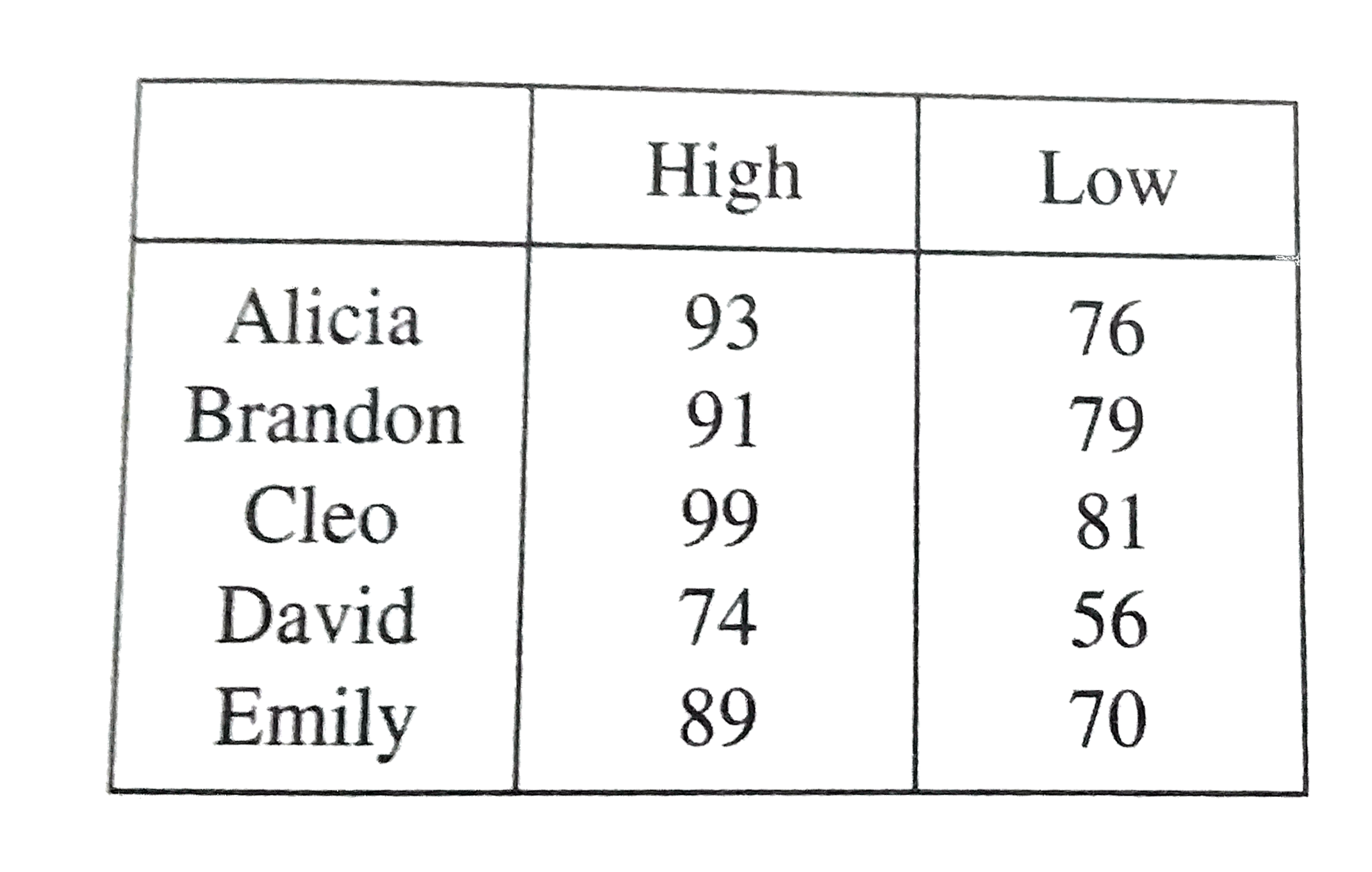 AAliciaBBrandonCCleoDEmily
AAliciaBBrandonCCleoDEmilyA student's mean score on 5 tests was 84. The student's mean score on the first 4 of these tests was 87. What was the student's score on the fifth of these tests ?
A68B72C75D81In a class of 10 boys and 15 girls, the average score on a biology test is 90. If the average score for the girls is x, what is the average score for the boys in terms of x ?
A200−23xB225−32xC250−2xD250−3x
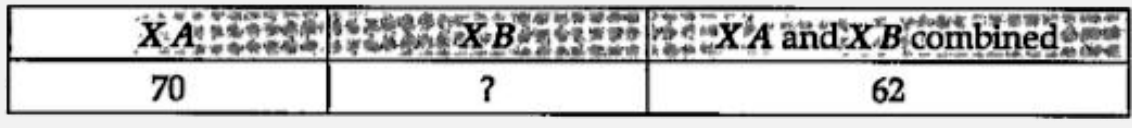
The number of students in section X A and X B are 30 and 35 respectively. The mean scores of students in the mathematices test are as follows:
View SolutionThe average scored by the students of a class in English is 64. The average of marks scored by boys and the girls are respectively 68 and 58. Then find the ratio of the number of boys to the number of girls.
View SolutionIn a class of 85% students pass in a surprise test. What per cent of students did not pass?
View SolutionThe average of 8, 13, x, and y is 6. The average of 15, 9, x and x is 8. What is the value of y?
View SolutionOn a certain exam, the medium grade for a group of 25 students is 67. If the highest grade on the exam is 90, which of the following could be the number of students that scored 67 on the exam?
I. 5
II. 20
III. 24
View Solution
