The stopping potential for acertain photosensitive metal is Vq when the frequency of incident radiation is V0 When the frequency of the incident radiations is doubled, what will be the stopping potential ?
V0
2V0
4V0
None of the above
To solve the problem, we need to understand the relationship between the stopping potential, the frequency of the incident radiation, and the work function of the metal.
1. Understanding Stopping Potential: The stopping potential V0 is defined as the potential difference needed to stop the most energetic photoelectrons emitted from the metal surface when illuminated by light of frequency ν0. The energy of the emitted electrons can be expressed as:
eV0=hν0−ϕ
where e is the charge of the electron, h is Planck's constant, ν0 is the frequency of the incident radiation, and ϕ is the work function of the metal.
2. Doubling the Frequency: When the frequency of the incident radiation is doubled, the new frequency becomes ν=2ν0. We can express the energy of the emitted electrons at this new frequency as:
eV=h(2ν0)−ϕ
3. Setting Up the Equation: The new stopping potential V can be expressed as:
eV=2hν0−ϕ
4. Relating the Two Stopping Potentials: Now we can relate the new stopping potential V to the original stopping potential V0:
eV=2hν0−ϕ
From the first equation, we know:
eV0=hν0−ϕ
Rearranging gives:
ϕ=hν0−eV0
5. Substituting Work Function: Now substitute ϕ back into the equation for V:
eV=2hν0−(hν0−eV0)
Simplifying this gives:
eV=2hν0−hν0+eV0
eV=hν0+eV0
6. Final Expression for Stopping Potential: Dividing through by e gives:
V=hν0e+V0
7. Conclusion: Therefore, the new stopping potential when the frequency is doubled is:
V=V0+hν0e
This indicates that the stopping potential increases but does not provide a specific numerical answer without knowing the work function or the initial stopping potential.
eV0=hν0−ϕ
where e is the charge of the electron, h is Planck's constant, ν0 is the frequency of the incident radiation, and ϕ is the work function of the metal.
eV=h(2ν0)−ϕ
eV=2hν0−ϕ
eV=2hν0−ϕ
From the first equation, we know:
eV0=hν0−ϕ
Rearranging gives:
ϕ=hν0−eV0
eV=2hν0−(hν0−eV0)
Simplifying this gives:
eV=2hν0−hν0+eV0
eV=hν0+eV0
V=hν0e+V0
V=V0+hν0e
This indicates that the stopping potential increases but does not provide a specific numerical answer without knowing the work function or the initial stopping potential.
Topper's Solved these Questions
- PHOTOELECTRIC EFFECT AND MATTER WAVESBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - PHOTOELECTRIC EFFECT AND MATTER WAVESEXERCISE - Conceptual MCQ
27 Videos
- PHOTOELECTRIC EFFECT AND MATTER WAVESBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - PHOTOELECTRIC EFFECT AND MATTER WAVESEXERCISE - Numerical MCQs
60 Videos
- PHOTOELECTRIC EFFECT AND MATTER WAVESBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - PHOTOELECTRIC EFFECT AND MATTER WAVESEXERCISE - Unsolved numerical problems
42 Videos
- NUCLEAR PHYSICS AND RADIOACTIVITYBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - NUCLEAR PHYSICS AND RADIOACTIVITYEXERCISE - Concptual MCQs
48 Videos
- THERMODYNAMICS LAWS & SPECIFIC HEATS OF GASES BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - THERMODYNAMICS LAWS & SPECIFIC HEATS OF GASES EXERCISE - Practice Exercise 3.4
11 Videos
Similar Questions
Knowledge Check
Which of the following is the graph between the frequency (v) of the incident radiations and the stopping potential ?
A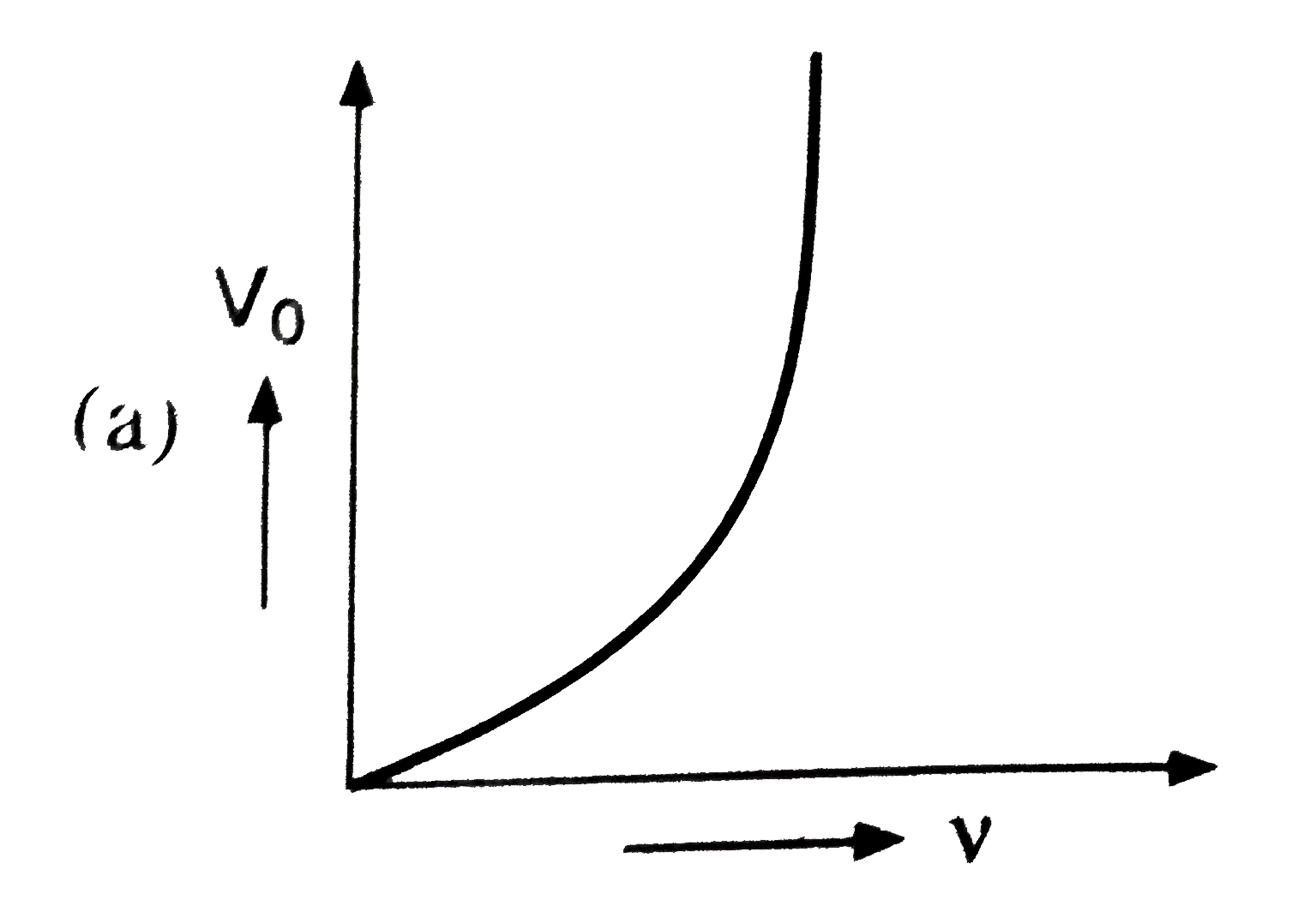 B
B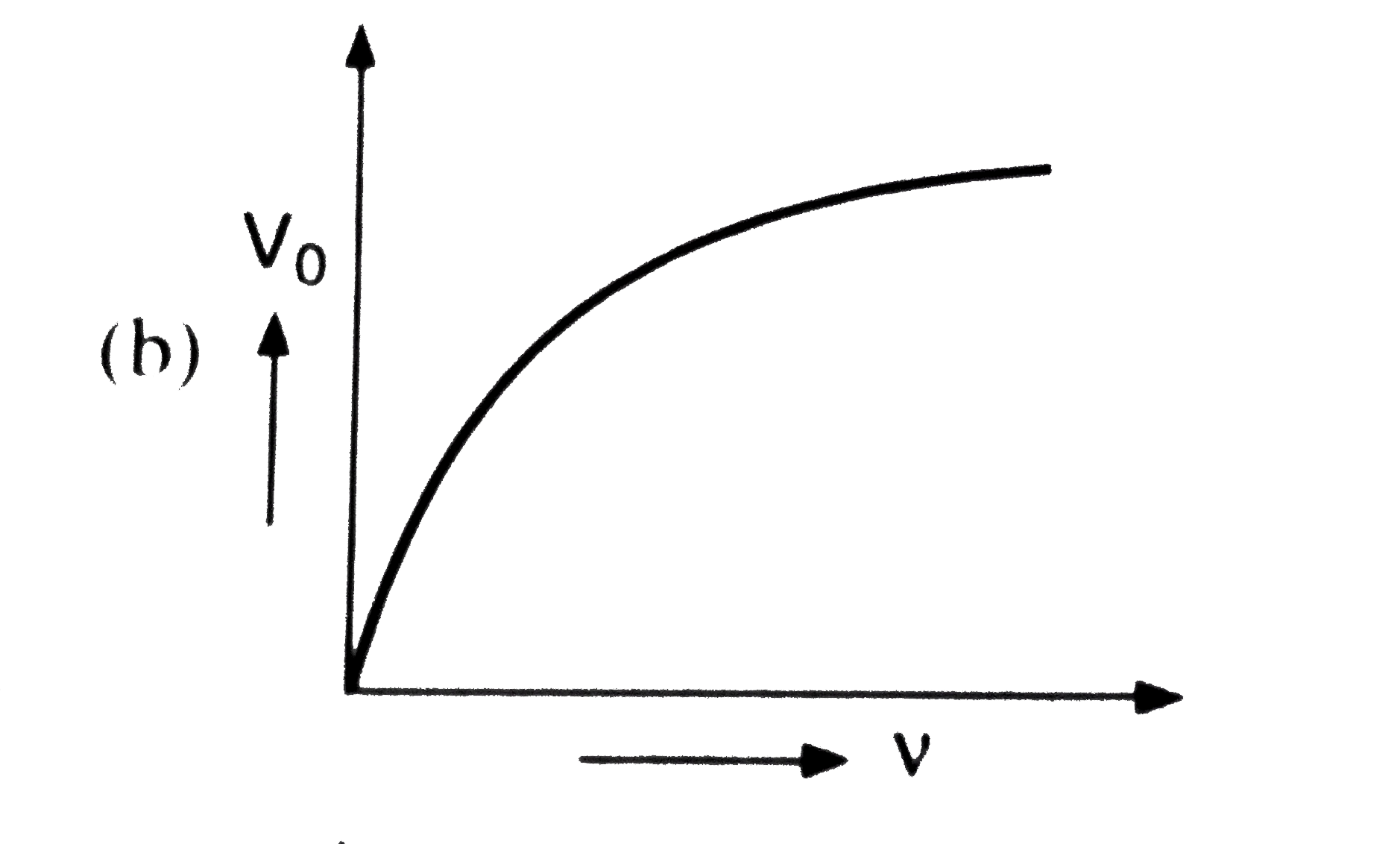 C
C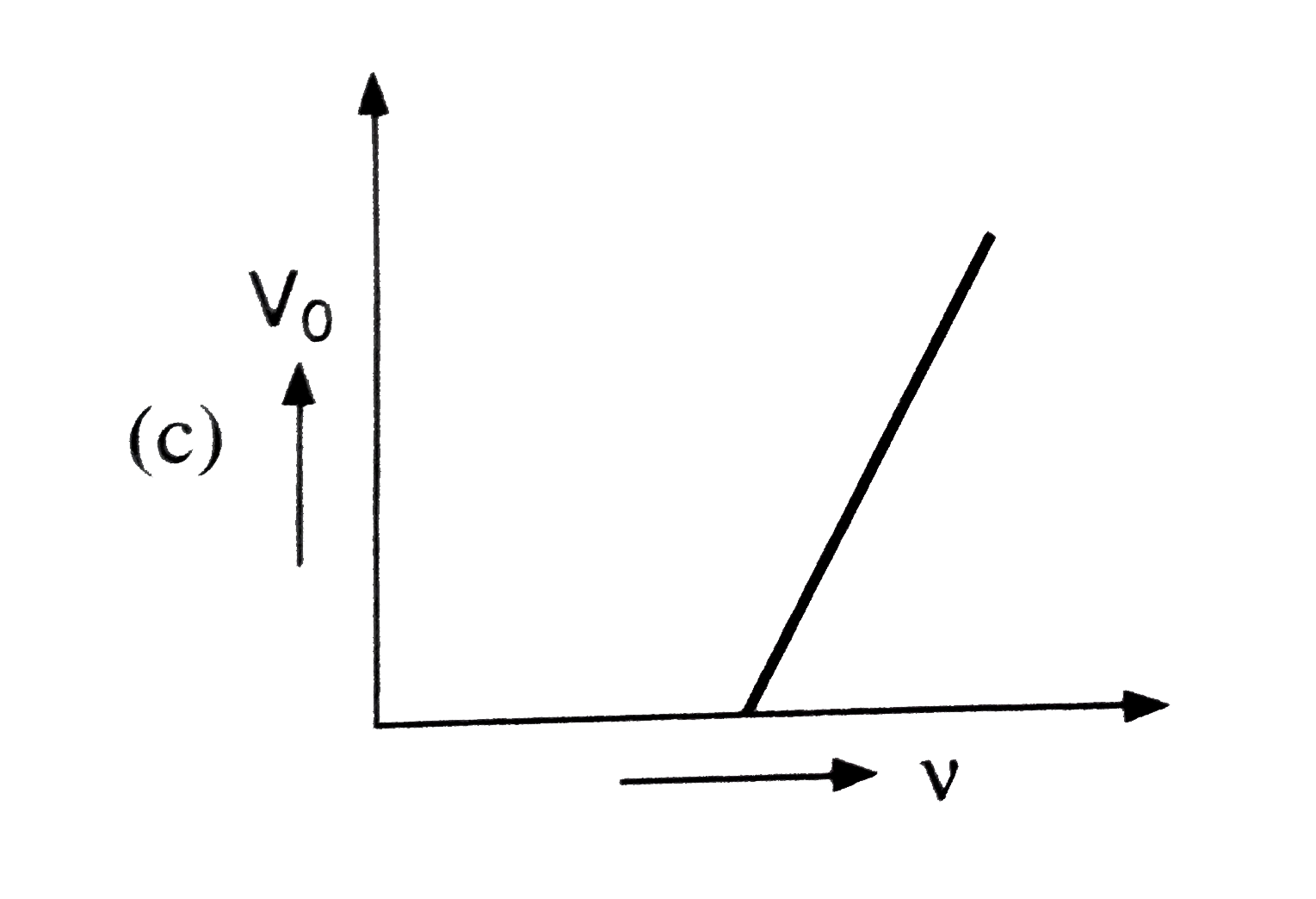 D
D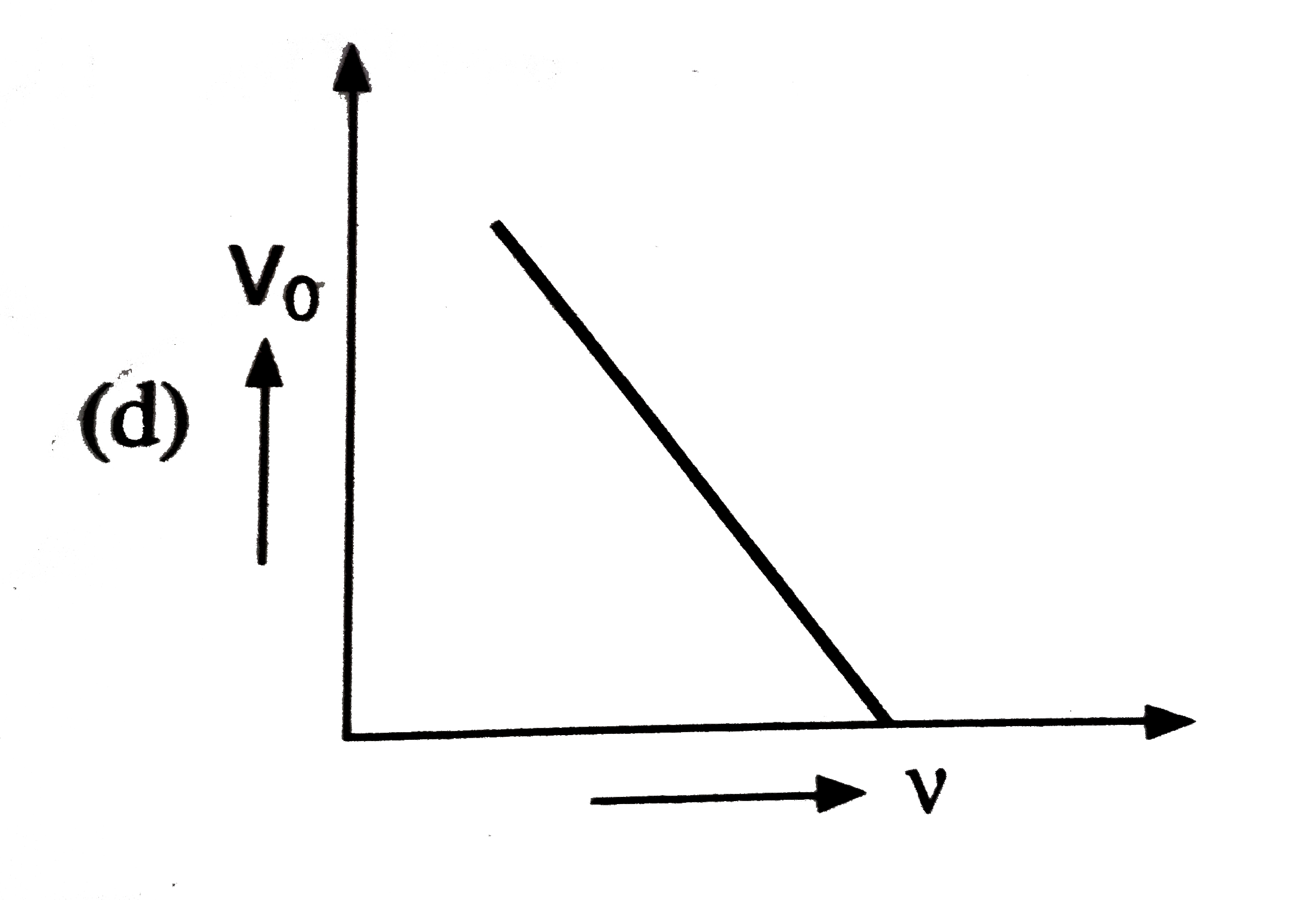
When the frequency of light incident an a metallic plate is doubled , the KE of the emitted photoelectrons will be :
AdoubledBhalvedCmore than doubledDincreases but less than doubled.When the frequency of light incident an a metallic plate is doubled , the KE of the emitted photoelectrons will be :
AdoubledBhalvedCmore than doubleDincreases but less than double.
Does the 'stopping potential' in photoelectric emission depend upon
(i) the intensity of the incident radiation in a photocell. ?
(ii) The frequency of the incident radiation ?
View SolutionState how in a photo-cell, the work function of the metal influence the kinetic energy of the emitted electrons.
(a) If the intensity of the incident radiation is doubled, what change occur in (i) the stopping potential and (ii) in photoelectric current?
(b) If the frequency of the incident radiation is doubled, what changes occur in the (i) stopping potential and (ii) photoelectric current.
View SolutionWhen light off frequency v1 is incident on a photosensitive surface , the stopping potential is V1 . If the frequency of incident radiation becomes v1/2 the stopping potential changes to V2 Find out the expression for the threshold frequency for the surface in terms of V1andV2
If the frequency of incident radiation is doubled , will the maximum kinetic energy of the photoelectrons also be doubled ? Given reason .
View SolutionKinetic energy of surface photoelectrons is x when frequency of incident radiation is v .If frequency of incident radiation is made 2v, the kinetic energy of surface photoelectron will be
View SolutionThe threshold frequency for a certain photosensitive metal is v0. When it is illuminated by light of frequency v=2v0, the stopping potential for photoelectric current is V0. What will be the stopping potential when the same metal is illuminated by light of frequency v=3v0?
View Solution
