The air tight and smooth pistons of a cylindrical vessel are connected with a string as shown. Initially pressure and temperature of the gas are P0 and T0. The atmospheric pressure is also P0. At a later time, tension in the string is 38P0A where A is the cross-sectional area of the cylinder. At this time, the temperature of the gas has become
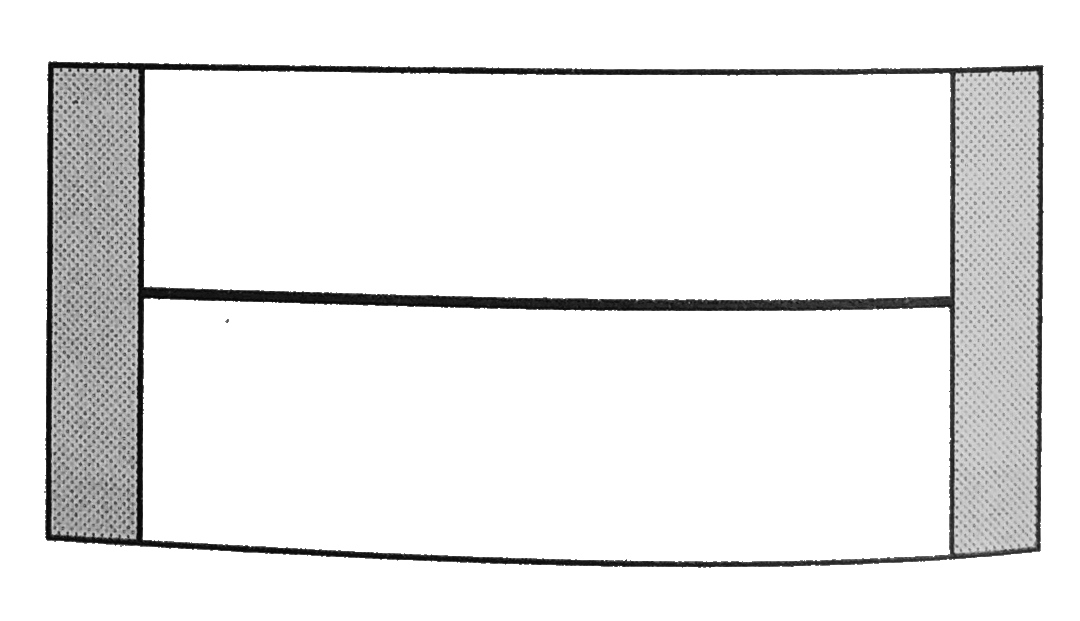
38T0
34T0
118T0
138T0
The correct Answer is:C
Step by step video, text & image solution for The air tight and smooth pistons of a cylindrical vessel are connected with a string as shown. Initially pressure and temperature of the gas are P_(0) and T_(0). The atmospheric pressure is also P_(0). At a later time, tension in the string is (3)/(8) P_(0)A where A is the cross-sectional area of the cylinder. At this time, the temperature of the gas has become by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- KINETIC THEORY OF GASESBook:CENGAGE PHYSICSChapter:KINETIC THEORY OF GASESExercise:Comprehension Type
Explore 5 Videos
- KINETIC THEORY OF GASESBook:CENGAGE PHYSICSChapter:KINETIC THEORY OF GASESExercise:Fill in the blanks
Explore 8 Videos
- KINEMATICS-2Book:CENGAGE PHYSICSChapter:KINEMATICS-2Exercise:Exercise Integer
Explore 9 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBook:CENGAGE PHYSICSChapter:KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSExercise:Interger
Explore 11 Videos
Similar Questions
An amount n (in moles) of a monatomic gas at initial temperature T0 is enclosed in a cylinderical vessel fitted with a ligth piston. The surrounding air has a temperature Ts(>T0) . And the atmospheric pressure is Pa . Heat may be conducted between the surrounding and the gas through the bottom of the cylinder. The bottom has a surface area A, thickness x and thermal conductivity K. Assuming all change to be slow, find the distance moved by the piston in time t.
View Solutionshows a cylindrical tube of cross-sectional area A fitted with two frictionless pistons. The pistons are connected to each other by a metallic wire. Initially, the temperature of the gas is T0and its pressure is p0which equals the atmospheric pressure, (a)What is the tention in the wire?(b)What will be the tention if the temperature is increased to 2T_(0)?

View Solution
Knowledge Check
The air tight and smooth piston of a cylindrical vessel are connected with a string as shown. Initially pressure and temperature of the gas are P0 and T0. The atmospheric pressure is also P0. At a later time, tension in the string is 38P0A where A is cthe cross-sectional are of the cylinder. at this time, the temperature of the gas has become.
 A38T0B34T0C118T0D138T0
A38T0B34T0C118T0D138T0In an adiabatic change, the pressure and temperature of a monoatomic gas are related with relation as P∝TC, Where C is equal to:
A35B53C25D52A metal sphere connected by a string is dipped in a liquid of density ρ as shown in figure. The pressure at the bottom of the vessel will be, (p0=atmospheric pressure )
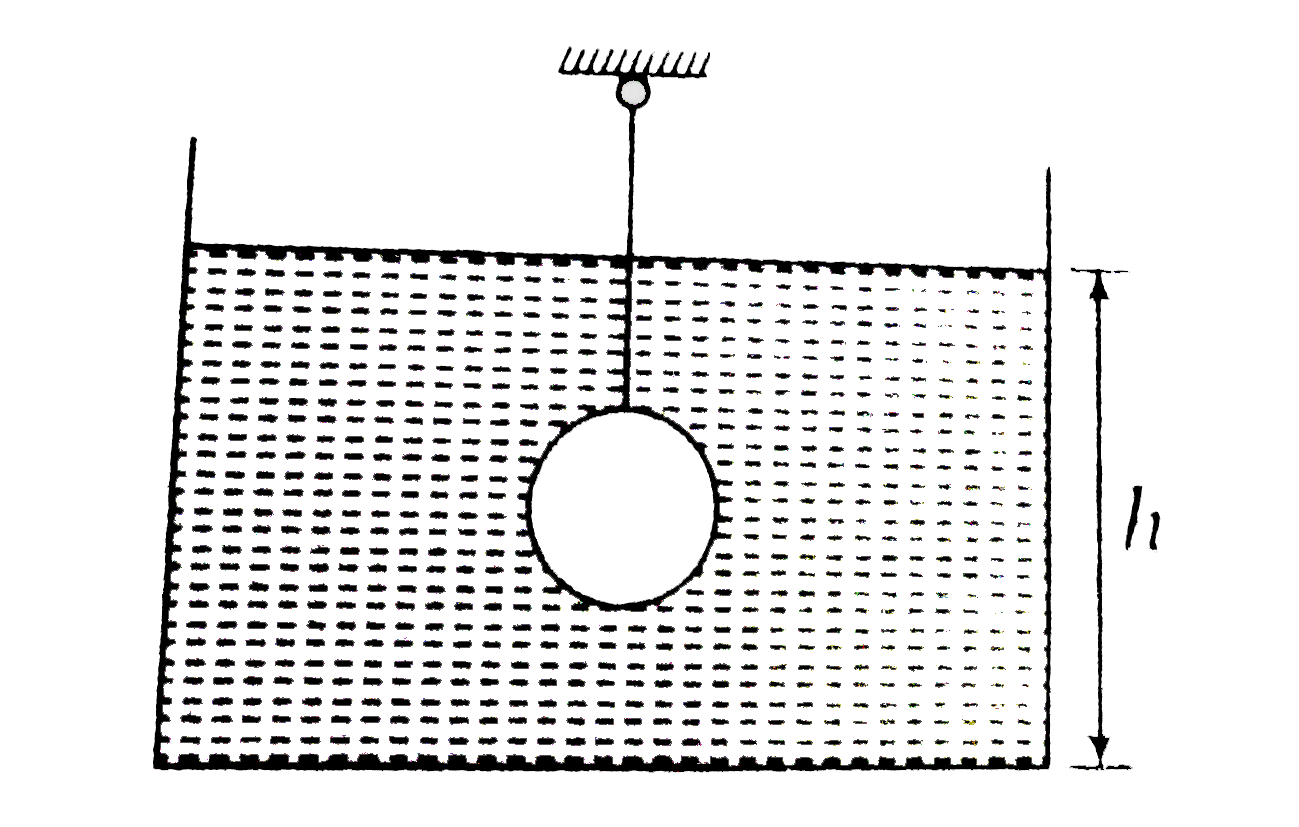 Ap=p0=ρghBp>p0+ρghCp<p0+ρghDp0
Ap=p0=ρghBp>p0+ρghCp<p0+ρghDp0
A cylinderical vessel contaning liquid is closed by a smooth piston of mass m. the area of cross-section of the piston is A. if the atmospheric pressure is P0, Find the pressure of the liquid just below the piston.
View SolutionAn adiabatic cylinder of cross section A is fitted with a mass less conducting piston of thickness d and thermal conductivity K. Initially, a monatomic gas at temperature T0 and pressure P0 occupies a volume V0 in the cylinder. The atmospheric pressure is P0 and the atmospheric temperature is T1(>T0). Find
(a) the temperature of the gas as a function of time
(b) the height raised by the piston as a function of time.
Neglect friction and heat capacities of the container and the piston.
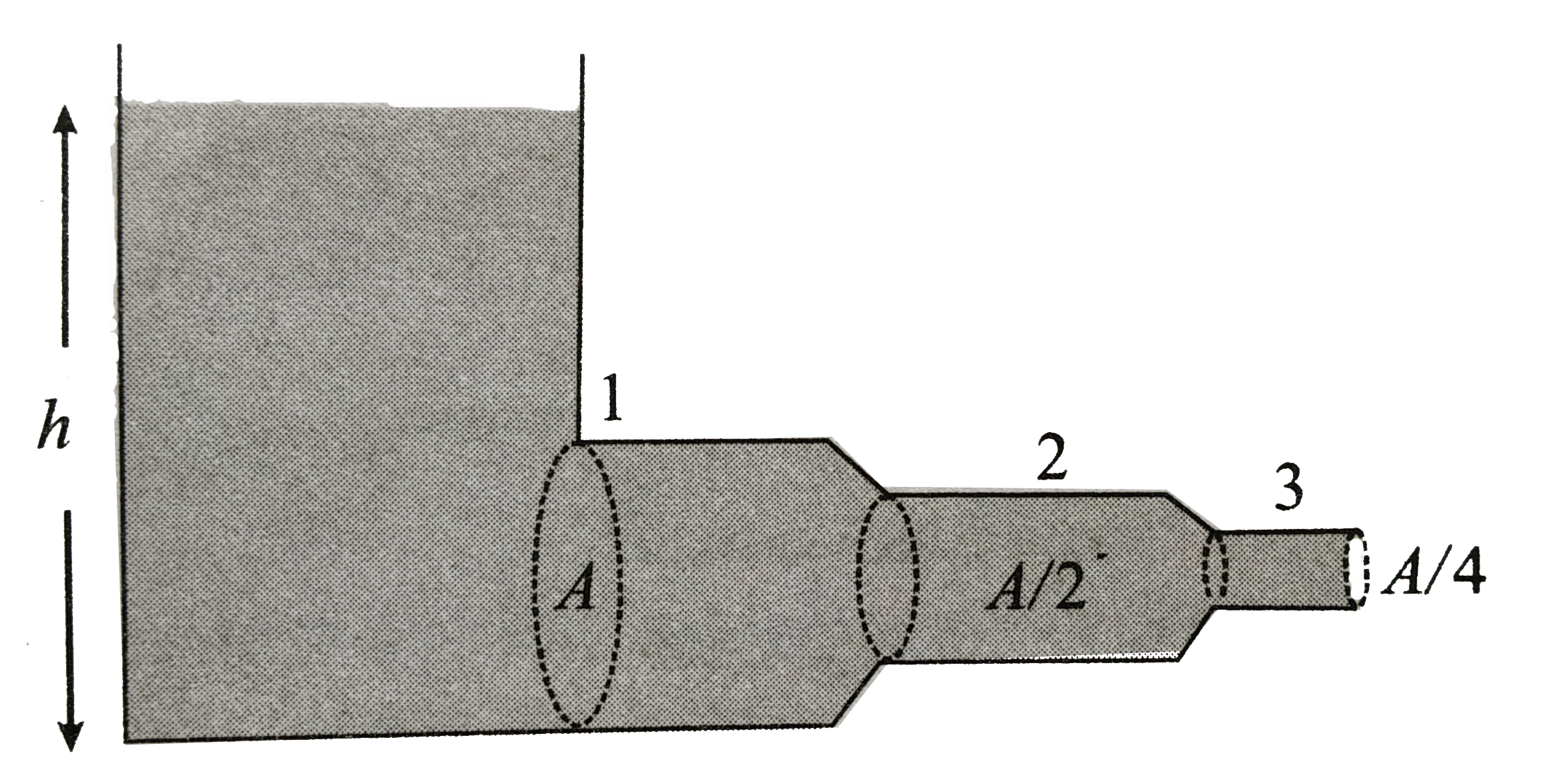
View SolutionIn the figure shown the velocity and pressure of the liquid at the cross section (2) are given by (If P0 is the atmospheric pressure).
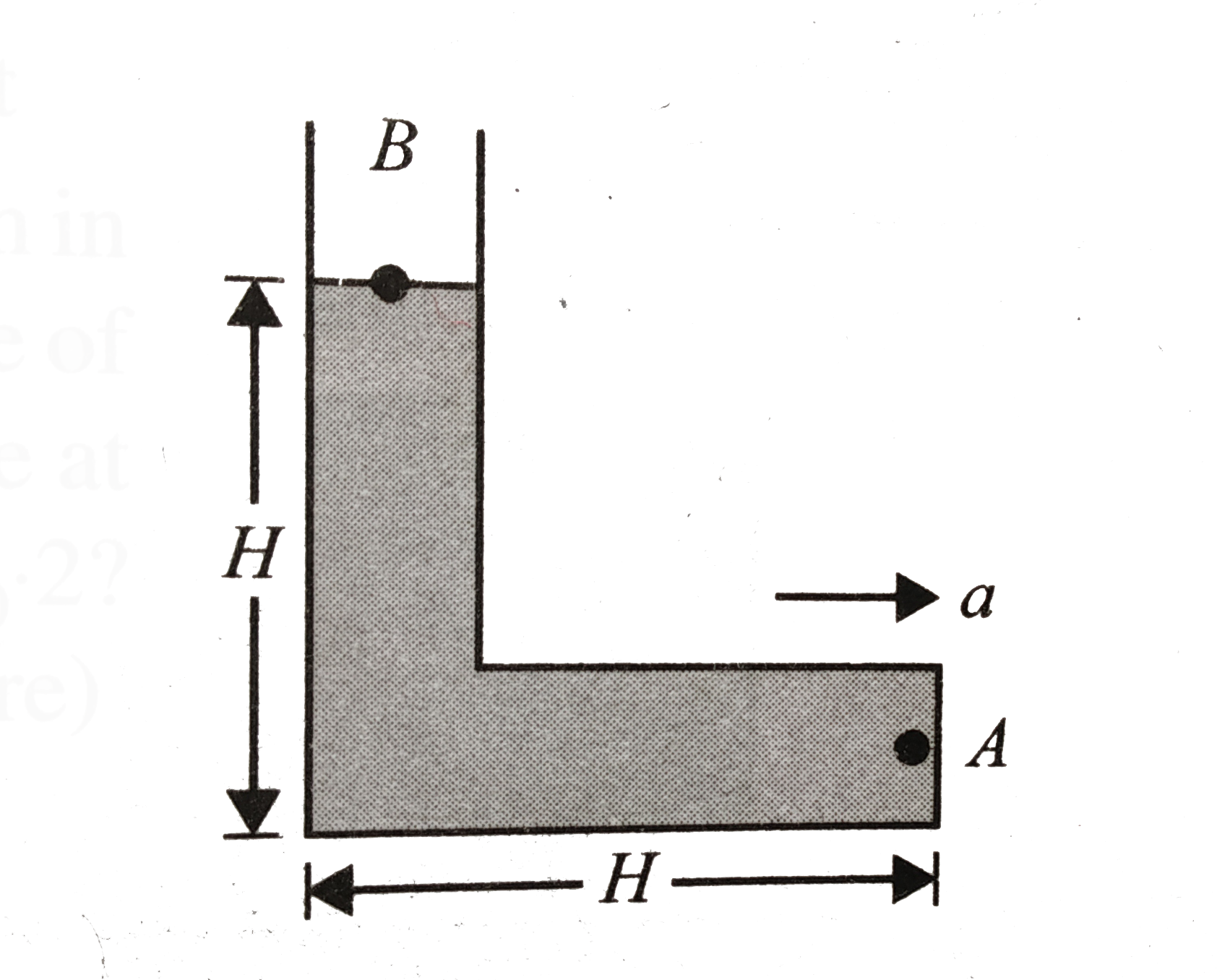
View SolutionFor the L-shaped vessel shown in the figure, determine the value of acceleration a so that pressure at point A becomes equal to p02? (p0 is the atmospheric pressure)
View SolutionTwo cylinders fitted with pistons and placed as shown, connected with string through a small tube of negligible volume, are filled with gas at pressure P0 and temperature T0. The radius of smaller cylinder is half of the other. If the temperature is increased to 2T0, find the pressure if the piston of bigger cylinder moves towards left by 1 metre ?

View Solution
