Two point-like charges a and b whose strengths are equal in absolute value are positioned at a certain distance from each other. Assuming the field strength is positive in the direction coinciding with the positive direction of the r axis, determine the signs of the charges for each distribution of the field strength between the charges shown in Figures (a),(b),(c),and(d).
Step by step video & image solution for Two point-like charges a and b whose strengths are equal in absolute value are positioned at a certain distance from each other. Assuming the field strength is positive in the direction coinciding with the positive direction of the r axis, determine the signs of the charges for each distribution of the field strength between the charges shown in Figures (a), (b), (c), and (d). by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Two point-like charge a and b whose strengths are equal in absolute value are positioned at a certain distance from each other. Assumeing the field strenght is positive in he direction of the r axis. Determine the sign of the charge for each distribution of the field strenght between charge for each distribution of the field strenght between charges shwon in fig.

View SolutionTwo equal like charges are positioned at a certain distance from each other. How do the electric field strength and the potential vary along the axis that passes through the midpoint of the distance between the charges at right angles to the line connecting the charges?
View Solution
Knowledge Check
Two point charges a and b whose magnitude are same, positioned at a certain distance along the positive x-axis from each other a is at origin. Graph is drawn between electrical field strength and distance x from a. E is taken positive if it is along the line joining from a to b From the graph it can be decided that
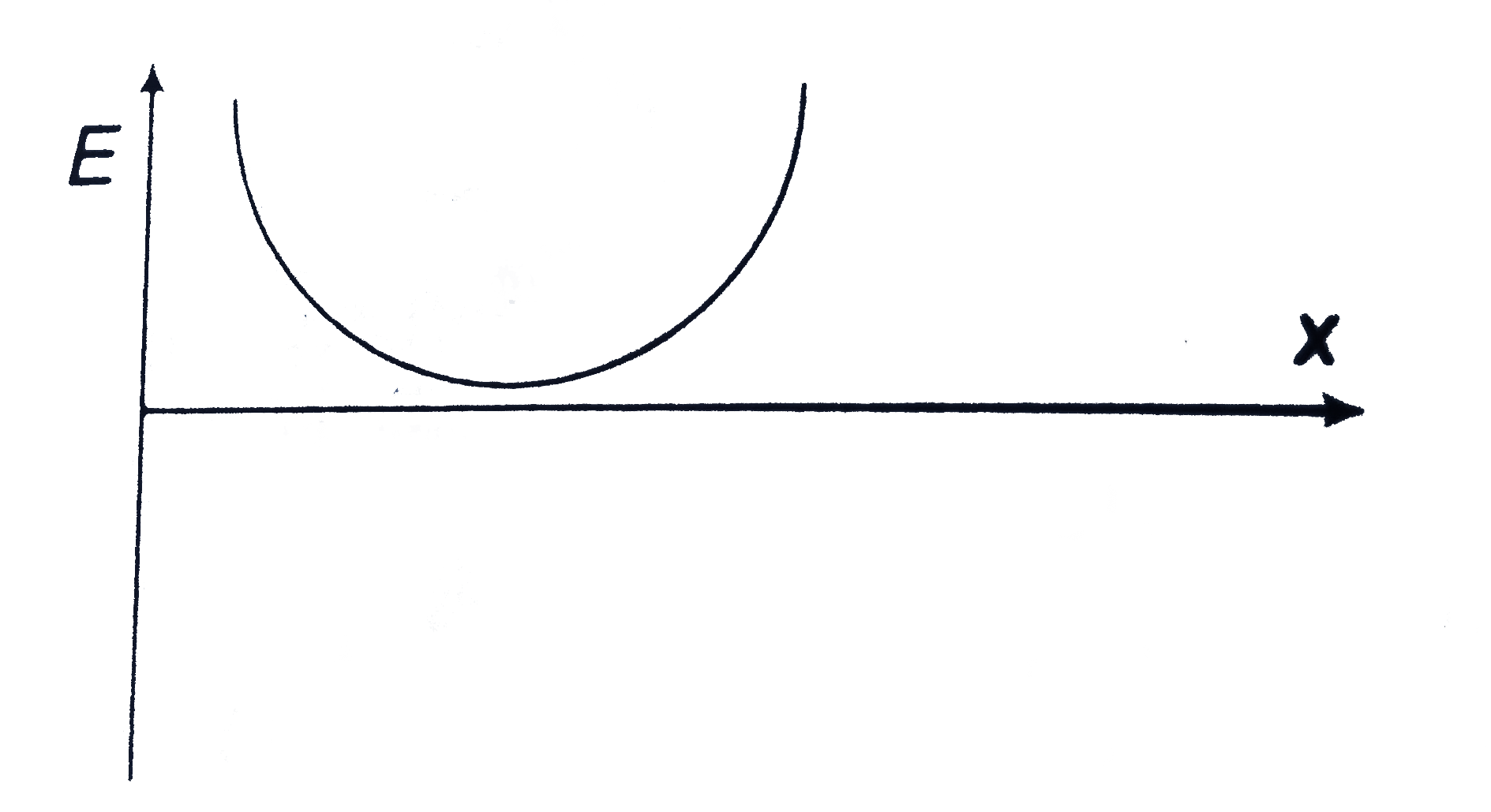 Aa is positive b is negativeBa and b both are positiveCa and b both are negativeDa is negative, b is positive
Aa is positive b is negativeBa and b both are positiveCa and b both are negativeDa is negative, b is positiveThe magnetic field strength B at the point between the capacitor plates is indicated in figure. B in terms of the rate of charge of the electric field strength, i.e., dE/dt between the plates is equal to
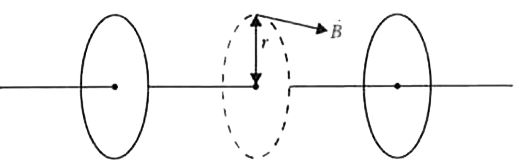 Aμ02πrdEdtBε0μ0r2dEdtCZeroDμ02rdEdt
Aμ02πrdEdtBε0μ0r2dEdtCZeroDμ02rdEdt
Two point charge Qa and Qb are positional at point A and B. The field strenght to the right of charge Qb on the line that passes therough the two charges varies according to a law represented schematically in fig. (without employing a definite scale). The field strength is assumed to be positive if its direction coincedes with the positive direction of the x-axis. The distance between the charges is l=21cm.
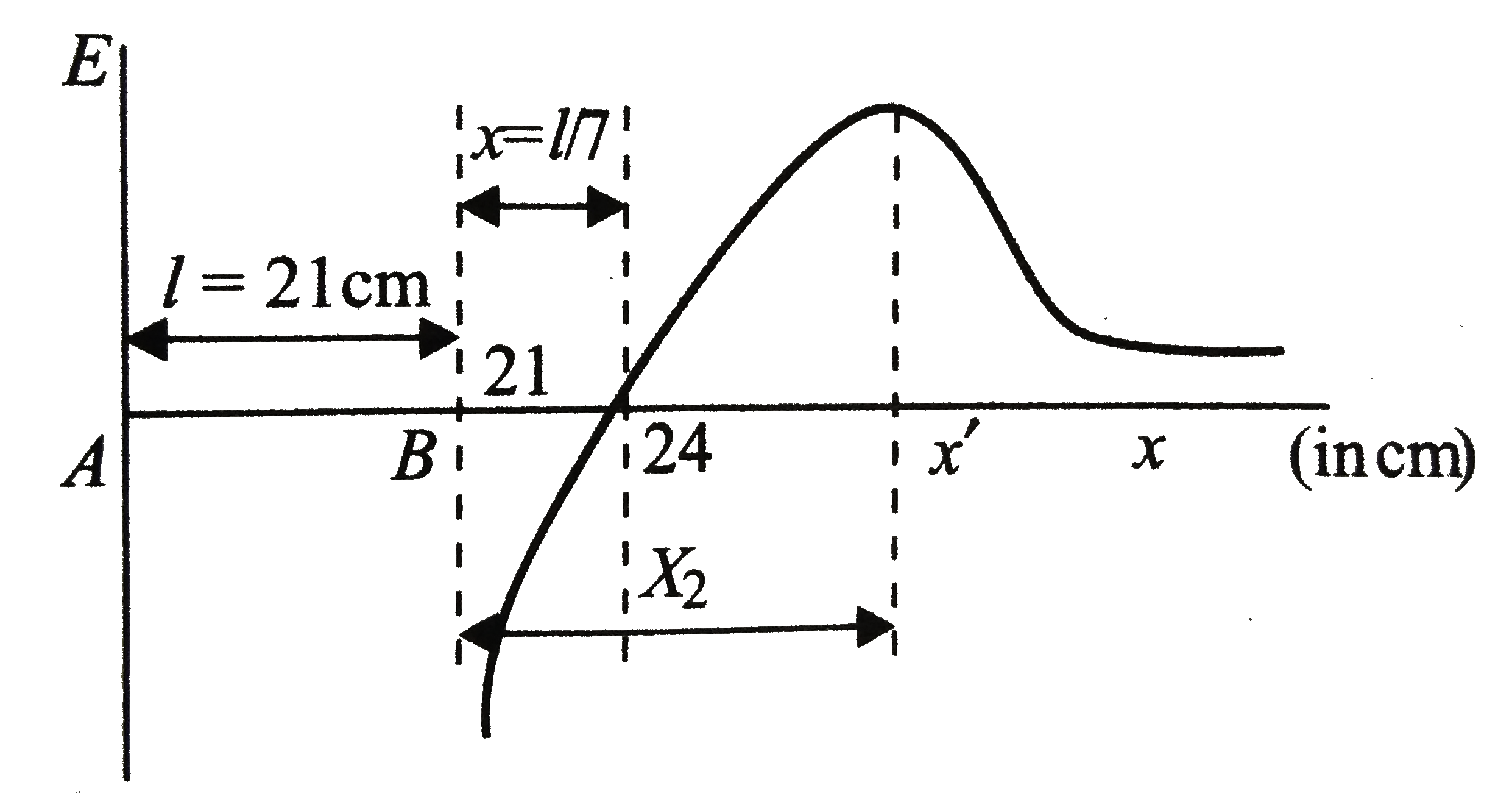
(a) Find the sign of the charges.
(b) Find the ration between the absolute value of charge Qa and Qb.
(c ) Find the coordinate x of the point where the field strenght is maximum.
View SolutionTwo point charges Q1 and Q2 are positioned at points I and 2. The field intensity to the right of the charge Q2 on the line that passes through the two charges varies according to a law that is represented in the figure. The field intensity is assumed to be positive if its direction coincides with the positive direction on the x-axis. The distance between the charges is l.
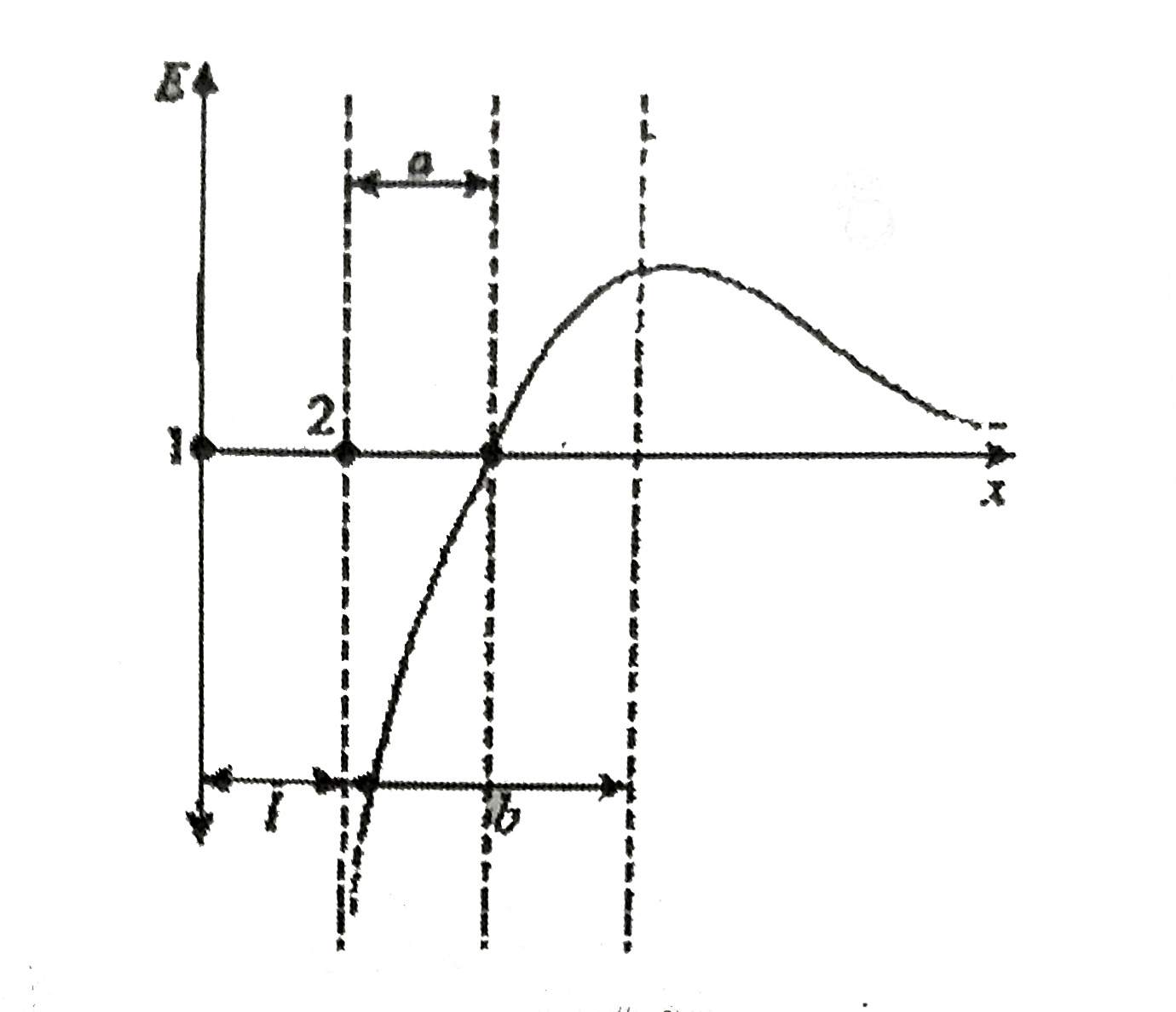
(a) Find the sign of each charge
(b) Find the ratio of the absolute values of the charges ∣∣∣Q1Q2∣∣∣
(c) Find the value of b where the field intensity is maximum
[(a) Q2 is negative and Q1 is positive (b) (l+aa)2, (c) l(l+aa)2/3−1⎤⎥ ⎥ ⎥⎦
View SolutionTwo point charges, Q1andQ2 are positioned at a certain distance from each other. Tho curves in the figure represent the distri bution of the potential along the straight line connecting the two charges. At which points (1,2,andor3) is the electric field strength zero? What are tho signes of the charges Q1andQ2 and which of the two is greater in magnitude?
View SolutionVarious potential distrihutions between two .point charges are shown in Figures (a)−(d) (the charges are equal in absolute value). Determine the signs of the charges for each case.
View SolutionTwo equal positive charges, each of 2μC interact with a third positive charge of 3μC situated as shown in Fig. Calculate the magnitude and direction of the force on the 3μC charge.

View SolutionTwo point charges q and −q are separated by the distance l, both being located at a distance l/2 from the infinite conducting plane. Find :
(a) the modulus of the electric force acting on each charge,
(b) the magnitude of the electric field strength vector at the midpoint between these charges.
View Solution
