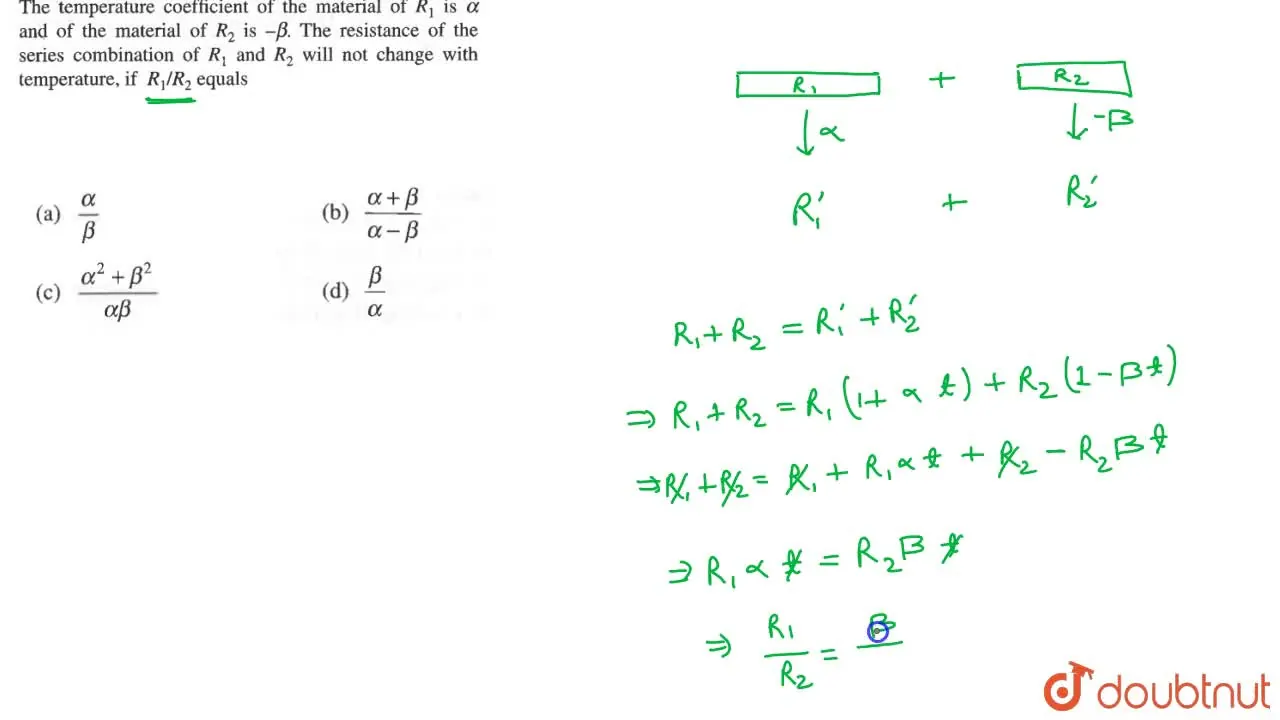
Two resistance R1 and R2 are made of different material. The temperature coefficient of the material of R1 is α and of the material of R2 is −β. Then resistance of the series combination of R1 and R2 will not change with temperature, if R1/R2 will not change with temperature if R1/R2 equals
αβ
α+βα−β
α2+β2αβ
βα
The correct Answer is:D
Step by step video, text & image solution for Two resistance R_(1) and R_(2) are made of different material. The temperature coefficient of the material of R_(1) is alpha and of the material of R_(2) is -beta. Then resistance of the series combination of R_(1) and R_(2) will not change with temperature, if R_(1)//R_(2) will not change with temperature if R_(1)//R_(2) equals by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC CURRENT & CIRCUITSEXERCISE - Combination of Resistance 114videos
ELECTRIC CURRENT & CIRCUITS
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC CURRENT & CIRCUITSEXERCISE - Combination of Resistance 214videos
ELECTRIC CURRENT & CIRCUITS
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - QUESTION BANK40videos
ELECTRIC CHARGES AND FIELDS
- BOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC CURRENT AND CIRCUITEXERCISE - Interger8videos
ELECTRIC CURRENT AND CIRCUIT
Similar Questions
Two resistances R1 and R2 are made of different materials. The temperature coefficient of the material of R1 is α and of the material of R2 is −β. The resistance of the series combination of R1 and R2 will not change with temperature, if R1/R2 equals.
View SolutionTwo spheres of radii R1 and R2 are made of the same material and are at the same temperature. The ratio of their thermal capacities is:
View SolutionThe temperature coefficient of resistance for two material A and B are 0.0031∘C and 0.0068∘C−1 respectively .Two resistance R1andR2 made from material A and B respectively . Have resistance of 200Ω and 100Ω at 0∘C. Show as a diagram the colour cube of a carbon resistance that would have a resistance equal to the series combination of r1andR2 at a temperature of 100∘C (Neglect the ring corresponding to the tolerance of the carbon resistor)
View SolutionTwo resistors of resistance R1 and R2 having R1>R2 are connected in parallel. For equivalent resistance R, the correct statement is
View SolutionTwo spherical black bodies of radii R1 and R2 and with surface temperature T1 and T2 respectively radiate the same power. R1/R2 must be equal to
View SolutionTow resistance R1=50±2 ohm and R2=60 pm connected in series , the equivalent resistance of the series combination in
View SolutionIf resistors of resistance R1 and R2 are connected in parallel,then resultant resistance is "
View SolutionTwo wires of resistance R1 and R2 have temperature coeficient of resistance α1 and α2 respectively. These are joined in series. The effeictive temperature coefficient of resistance is
View SolutionTwo reactions r1 and r2 proceed at 25∘C at the same rate. The temperature coefficient of the second reaction is 2.5 times of temperature coefficient of the first reaction. The ratio of rates of these reactions at 96∘C will be (r2/r1), approx
View SolutionTwo resistors R1 and R2 are in series.Their coefficients are alpha1 and alpha2 .Find the effective value of alpha ?
View Solution