Equal charges Q are placed at the four corners A, B, C, D of a square of length a. The magnitude of the force on the charge at B will be
3Q24πε0a2
4Q24πε0a2
(1+1√22)Q24πε0a2
(2+1√2)Q24πε0a2
The correct Answer is:C
To solve the problem of finding the magnitude of the force on the charge at corner B of a square with equal charges at each corner, we can follow these steps:
1. Identify the Charges and Their Positions:
- Let the charges at corners A, B, C, and D be Q.
- The square has a side length a.
2. Calculate the Forces Due to Each Charge on Charge B:
- Force due to Charge A (F_A):
- The distance between charges A and B is a.
- The force FA can be calculated using Coulomb's law:
FA=kQ2a2
- This force acts along the line connecting A and B (to the right).
- Force due to Charge C (F_C):
- The distance between charges B and C is also a.
- The force FC is given by:
FC=kQ2a2
- This force acts along the line connecting B and C (upward).
- Force due to Charge D (F_D):
- The distance between charges B and D is √2a (the diagonal of the square).
- The force FD is given by:
FD=kQ2(√2a)2=kQ22a2
- This force acts at a 45∘ angle to the horizontal (downward and to the right).
3. Resolve the Forces:
- The forces FA and FC act along the x-axis and y-axis, respectively.
- The force FD can be resolved into its x and y components:
- FDx=FDcos(45∘)=kQ22a2⋅1√2=kQ22√2a2
- FDy=FDsin(45∘)=kQ22a2⋅1√2=kQ22√2a2
4. Calculate the Net Force in the X and Y Directions:
- Net Force in the X-Direction (F_x):
Fx=FA+FDx=kQ2a2+kQ22√2a2
- Net Force in the Y-Direction (F_y):
Fy=FC−FDy=kQ2a2−kQ22√2a2
5. Calculate the Magnitude of the Resultant Force:
- The magnitude of the resultant force Fnet can be found using the Pythagorean theorem:
Fnet=√F2x+F2y
6. Substituting the Values:
- Substitute the expressions for Fx and Fy into the equation for Fnet and simplify.
Final Result:
The magnitude of the force on the charge at B can be expressed as:
Fnet=
⎷(kQ2a2+kQ22√2a2)2+(kQ2a2−kQ22√2a2)2
- Let the charges at corners A, B, C, and D be Q.
- The square has a side length a.
- Force due to Charge A (F_A):
- The distance between charges A and B is a.
- The force FA can be calculated using Coulomb's law:
FA=kQ2a2
- This force acts along the line connecting A and B (to the right).
- The distance between charges B and C is also a.
- The force FC is given by:
FC=kQ2a2
- This force acts along the line connecting B and C (upward).
- The distance between charges B and D is √2a (the diagonal of the square).
- The force FD is given by:
FD=kQ2(√2a)2=kQ22a2
- This force acts at a 45∘ angle to the horizontal (downward and to the right).
- The forces FA and FC act along the x-axis and y-axis, respectively.
- The force FD can be resolved into its x and y components:
- FDx=FDcos(45∘)=kQ22a2⋅1√2=kQ22√2a2
- FDy=FDsin(45∘)=kQ22a2⋅1√2=kQ22√2a2
- Net Force in the X-Direction (F_x):
Fx=FA+FDx=kQ2a2+kQ22√2a2
- Net Force in the Y-Direction (F_y):
Fy=FC−FDy=kQ2a2−kQ22√2a2
- The magnitude of the resultant force Fnet can be found using the Pythagorean theorem:
Fnet=√F2x+F2y
- Substitute the expressions for Fx and Fy into the equation for Fnet and simplify.
The magnitude of the force on the charge at B can be expressed as:
Fnet=
⎷(kQ2a2+kQ22√2a2)2+(kQ2a2−kQ22√2a2)2
Topper's Solved these Questions
- COULOMB LAW AND ELECTRIC FIELDBook:CENGAGE PHYSICSChapter:COULOMB LAW AND ELECTRIC FIELDExercise:Multiple Correct Answer Type
Explore 1 Video
- COULOMB LAW AND ELECTRIC FIELDBook:CENGAGE PHYSICSChapter:COULOMB LAW AND ELECTRIC FIELDExercise:Single Answer Correct Type
Explore 22 Videos
- COULOMB LAW AND ELECTRIC FIELDBook:CENGAGE PHYSICSChapter:COULOMB LAW AND ELECTRIC FIELDExercise:Subjective Type
Explore 13 Videos
- COMMUNICATION SYSTEMBook:CENGAGE PHYSICSChapter:COMMUNICATION SYSTEMExercise:QUESTION BANK
Explore 19 Videos
- Current ElectricityBook:CENGAGE PHYSICSChapter:Current ElectricityExercise:QUESTION BANK
Explore 40 Videos
Similar Questions
A charge Q is placed at the corner of a cube of edge length L. The electric flux linked to one of the faces not touching the charge Q is
View SolutionFour identical charges q are placed at the corners of a square of side a. Find the potential energy of one of the charges due to the remaining charges.
 .
.
View Solution
Knowledge Check
Equal charges q are placed at the three corners B, C, D of a square ABCD of side a. The potential at A is
A14πε0qaB3q4πε0Cq4πε0a[2+1√2]Dq4πε0a[1+√2]Equal charges q are placed at the three corners B, C, D of a square ABCD of side 'a'. The ratio of potential at A to centre O is
A2√2+13√2B2+√26C2√2+16D2√2+13q,2q, 3q and 4q charges are placed at the four corners A,B,C and D of a square. The field at the centre P of the square has the direction parallel to
 AABBCBCACDBD
AABBCBCACDBD
Four identical point charges q are placed at four corners of a square of side a. Find the potential energy of the charge system.
 .
.
View SolutionCharges Q−q,Q,−q are placed at the corners A,B,C,D of a square respectively. If the resultant force on the charge Q is zero due to other charges, what is the relation between Q and q?
View SolutionFour equal charges Q are placed at the four corners of a square of each side is 'a'. Work done in removing a charge −Q from its centre to infinity is
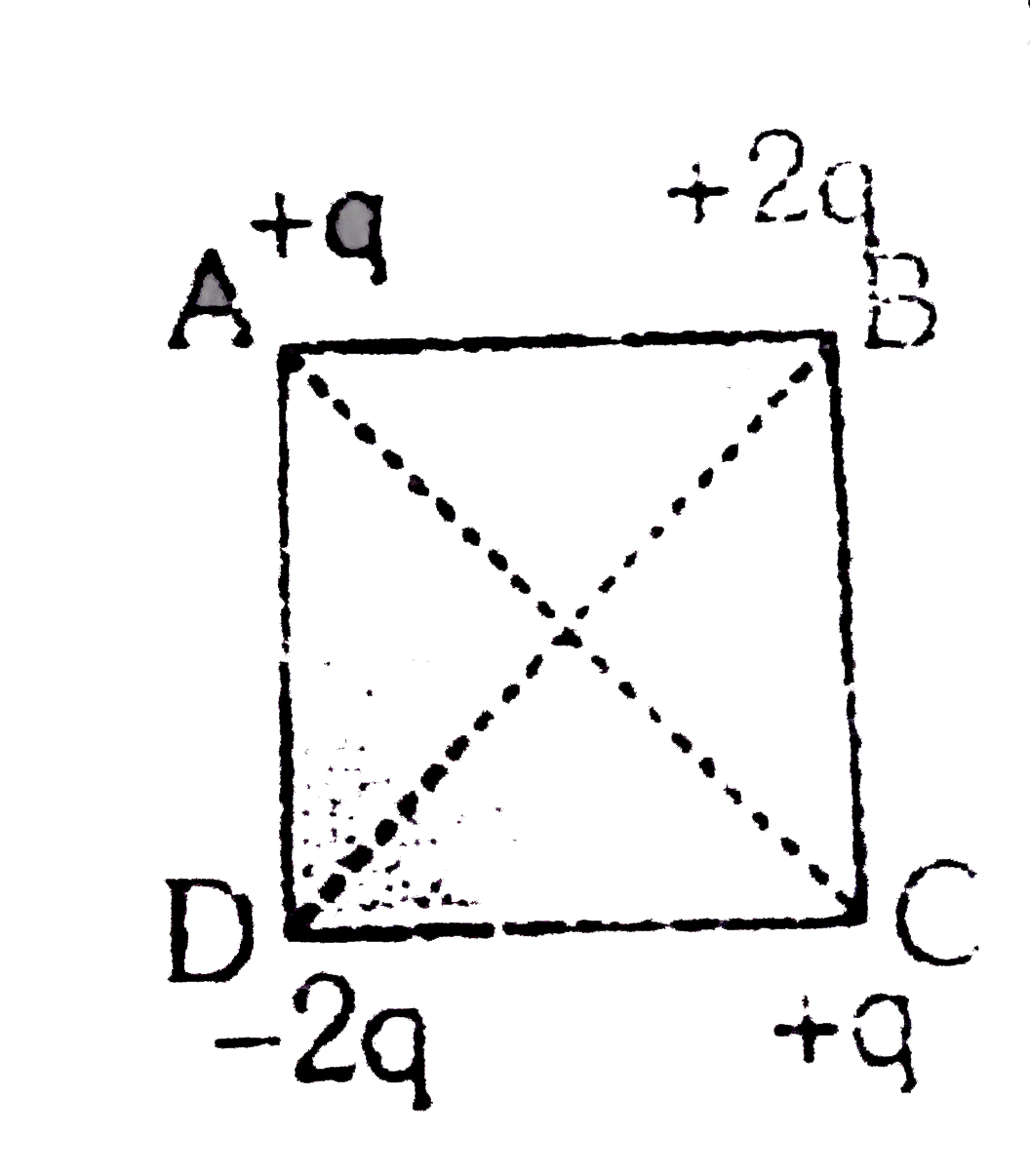
View SolutionFour charges are arranged at the corners of a square ABCD as shown in the figure. The force on the charge kept at the centre O will be:
View SolutionThree point charges each of value +q are placed on three vertices of a square of side a metre. What is the magnitude of the force on a point charge of value −q coulomb placed at the centre of the square?
View Solution