Charges 4Q, q and Q and placed along x-axis at positions x=0,x=t/2 and x=1, respectively. Find the value of q so that force on charge Q is zero
Q
Q/2
−Q/2
−Q
The correct Answer is:D
To solve the problem of finding the value of charge q such that the net force on charge Q is zero, we can follow these steps:
Step 1: Identify the positions of the charges
We have three charges:
- Charge 4Q is located at x=0
- Charge q is located at x=12
- Charge Q is located at x=1
Step 2: Determine the forces acting on charge Q
The forces acting on charge Q are due to the other two charges:
1. The force F1 due to charge 4Q (located at x=0).
2. The force F2 due to charge q (located at x=12).
Step 3: Calculate the force F1 on charge Q
The force F1 exerted by charge 4Q on charge Q is given by Coulomb's law:
F1=14πϵ0⋅(4Q)(Q)(1−0)2=4Q24πϵ0
This force is repulsive since both charges are positive.
Step 4: Calculate the force F2 on charge Q
The force F2 exerted by charge q on charge Q is also given by Coulomb's law:
F2=14πϵ0⋅(q)(Q)(1−12)2=qQ4πϵ0⋅(12)2=4qQ4πϵ0
This force is attractive if q is negative (since Q is positive).
Step 5: Set the net force on charge Q to zero
For the net force on charge Q to be zero, we need:
F1−F2=0
Substituting the expressions for F1 and F2:
4Q24πϵ0−4qQ4πϵ0=0
This simplifies to:
4Q2=4qQ
Dividing both sides by 4Q (assuming Q≠0):
Q=q
Step 6: Determine the sign of q
Since the force F1 is repulsive and F2 must be attractive to balance it, q must be negative:
q=−Q
Conclusion
Thus, the value of charge q that makes the net force on charge Q equal to zero is:
q=−Q
We have three charges:
- Charge 4Q is located at x=0
- Charge q is located at x=12
- Charge Q is located at x=1
The forces acting on charge Q are due to the other two charges:
1. The force F1 due to charge 4Q (located at x=0).
2. The force F2 due to charge q (located at x=12).
The force F1 exerted by charge 4Q on charge Q is given by Coulomb's law:
F1=14πϵ0⋅(4Q)(Q)(1−0)2=4Q24πϵ0
This force is repulsive since both charges are positive.
The force F2 exerted by charge q on charge Q is also given by Coulomb's law:
F2=14πϵ0⋅(q)(Q)(1−12)2=qQ4πϵ0⋅(12)2=4qQ4πϵ0
This force is attractive if q is negative (since Q is positive).
For the net force on charge Q to be zero, we need:
F1−F2=0
Substituting the expressions for F1 and F2:
4Q24πϵ0−4qQ4πϵ0=0
This simplifies to:
4Q2=4qQ
Dividing both sides by 4Q (assuming Q≠0):
Q=q
Since the force F1 is repulsive and F2 must be attractive to balance it, q must be negative:
q=−Q
Thus, the value of charge q that makes the net force on charge Q equal to zero is:
q=−Q
Topper's Solved these Questions
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICSCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Multiple Correct Answers Type
2 Videos
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICSCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Comprehension Type
2 Videos
- COULOMB LAW AND ELECTRIC FIELDBOOK - CENGAGE PHYSICSCHAPTER - COULOMB LAW AND ELECTRIC FIELDEXERCISE - Integer
8 Videos
- COMMUNICATION SYSTEMBOOK - CENGAGE PHYSICSCHAPTER - COMMUNICATION SYSTEMEXERCISE - QUESTION BANK
19 Videos
- Current ElectricityBOOK - CENGAGE PHYSICSCHAPTER - Current ElectricityEXERCISE - QUESTION BANK
40 Videos
Similar Questions
Point charges q1 and q2 lie o the x-axis at points x=−a and x=+a respectively.
a. How must q1 and q2 be related for the net electrostatic force on point charge +Q, placed at x=+a2, to be zero?
b. With the same point charge +Q now placed ate x=+3a/2
View Solution(a) Two point charges Q and 16Q are fixed at separation d. Where should a third point charge be placed between them so that it experiences no force I.e. in equilibrium ?
(b) In the previous problem , if we replace Q by −Q , where should the third charge be placed on the joining the charges so that it is equilibrium ?
(c ) Three point charges Q,q and Q are placed at x=0,x=d/2 and x=d. Find q in terms of Q so that all charges are in equilibrium.
(d) Two point charges 9Q and 25Q are placed at separation d. Where should a third charge q be placed between them so that all charges are in equilibrium ? Also find the value of q and its nature.
View Solution
Knowledge Check
Point charges +4q,−q and +4q are kept on the x-axis at points x=0,x=a and X=2a respectively, then
AOnly q is in stable equilibriumBNone of the charges are in equilibriumCAll the charges are in unstable equilibriumDAll the charges are in stable equlibriumPoint charges +4q,−q are kept on the x-axis at points x=0,x=a and X=2a respectively, then
AOnly q is in stable equilibriumBNone of the charges are in equilibriumCAll the charges are in unstable equilibriumDAll the charges are in stable equilibriumThree charges 4q,Q and q are in a straight line in the position of 0.l/2 and l respectively. The resultant force on q will be zero, if Q=
A−qB−2qC−q2D4q
An infinite number of charges, each equal to q, are placed along the x -axis at x=1,x=2,x=4,x=8, etc. The electric field at the point x=0 due to the set of charges is qnπɛ0. Find n.
View SolutionIn the configuration as shown +q charge is placed at origin and Q and Q are placed at distance a and a0 respectively from origin. If net electrostatic force on charge (+q) is along negative y-axis and θ=θ2/2, then (Q/Q) is equal to:
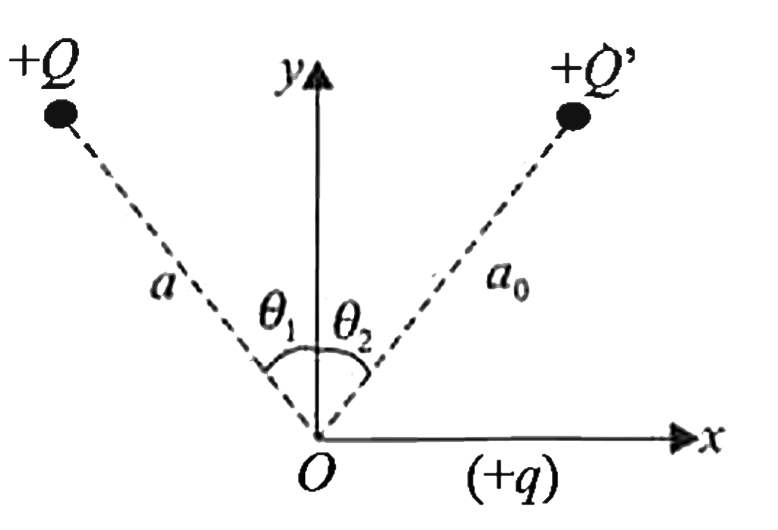
View SolutionCharges Q−q,Q,−q are placed at the corners A,B,C,D of a square respectively. If the resultant force on the charge Q is zero due to other charges, what is the relation between Q and q?
View SolutionIn the figure, two point charges +qand−q are placed on the x-axis at (+a,0)and(−a,0) respectively. A tiny dipole of dipolem moment (p) is kept at the origin along the y-axis. The torque on the dipole equals

View SolutionA two point charges 4q and -q are fixed on the x - axis x=−d2andx=d2 , respectively. If a third point charge 'q' is taken from the origin to x = d along the semicircle as shown in the figure, the energy of the charge will
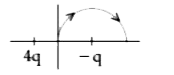
View Solution