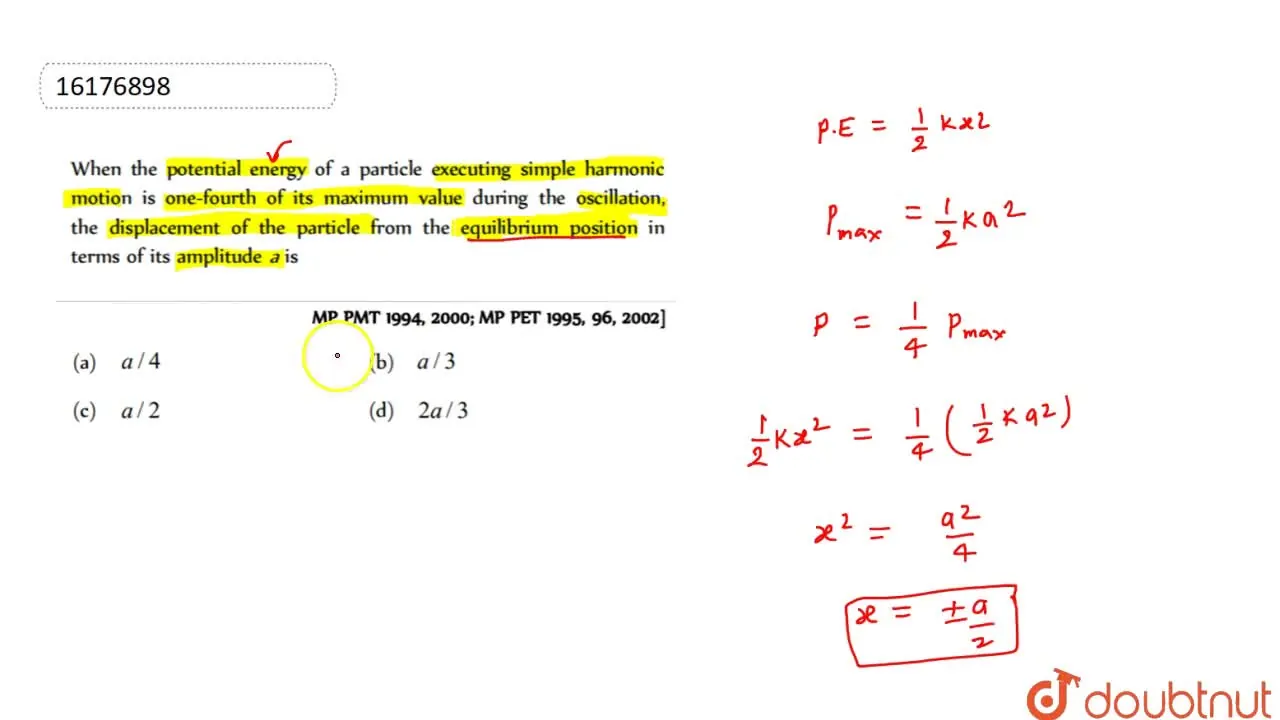
When the potential energy of a particle executing simple harmonic motion is one-fourth of its maximum value during the oscillation, the displacement of the particle from the equilibrium position in terms of its amplitude a is
a/4
a/3
a/2
2a/3
The correct Answer is:C
Step by step video, text & image solution for When the potential energy of a particle executing simple harmonic motion is one-fourth of its maximum value during the oscillation, the displacement of the particle from the equilibrium position in terms of its amplitude a is by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Related Playlists
- BOOK - ERRORLESS CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Time period and frequency18videos
SIMPLE HARMONIC MOTION
- BOOK - ERRORLESS CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - simple pendulum61videos
SIMPLE HARMONIC MOTION
- BOOK - ERRORLESS CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Acceleration of simple Harmonic motion21videos
SIMPLE HARMONIC MOTION
- BOOK - ERRORLESS CHAPTER - ROTATIONAL MOTIONEXERCISE - Practice Problems (Problems based on motion of connected mass)10videos
ROTATIONAL MOTION
- BOOK - ERRORLESS CHAPTER - SURFACE TENSIONEXERCISE - Exercise214videos
SURFACE TENSION
Similar Questions
The total energy of a particle executing simple harmonic motion is
View SolutionThe total energy of a particle having a displacement x, executing simple harmonic motion is
View SolutionThe potential energy of a particle, executing a simple harmonic motion, at a dis"tan"ce x from the equilibrium position is proportional to
View SolutionA particle is executing simple harmonic motion with an amplitude A and time period T. The displacement of the particles after 2T period from its initial position is
View SolutionThe kinetic energy and potential energy of a particle executing simple harmonic motion will be equal, when displacement (amplitude =a) is
View SolutionA particle is executing simple harmonic motion .Its total energy is proportional to its -
View SolutionThe total energy of a particle, executing simple harmonic motion is.
where x is the displacement from the mean position, hence total energy is independent of x.View SolutionWhen the displacement of a particle executing simple harmonic motion is half its amplitude, the ratio of its kinetic energy to potential energy is
View SolutionThe total mechanical energy of a particle executing simple harmonic motion is E when the displacement is half the amplitude of its kinetic energy will be
View SolutionA particle executes simple harmonic motion. The amplitude of vibration of particle is 2 cm. The displacement of particle in one time period is
View Solution