Question

 Write the formula for the refractive index of the prism.

Explanation

Observe the ray diagram in the figure.
[d is the exterior angle of `DeltaOMN`. Exterior angle is equal to the sum of interior opposite angles.]
From `DeltaOMN`, we get
`d=i_(1) - r_(1) + i_(2) -r_(2)`
`therefore d=(i_(1)+i_(2))-(r_(1)+r_(2))" "-(1)`
From `DeltaPMN`, we have
`A+(90-r_(1))-(90-r_(2))=180^(@)`
`A+cancel180-(r_(1)+r_(2))=cancel(180)`
`A+cancel(180)-(r_(1)+r_(2))=cancel(180)`
`A=r_(1)+r_(2)" "-(2)`
From (1) and (2) , `d=i_(1)+i_(2)-A`
From (1) and (2) , `d=i_(1) +i_(2)-A`
`implies A+d=i_(1)+i_(2)" "-(3)`

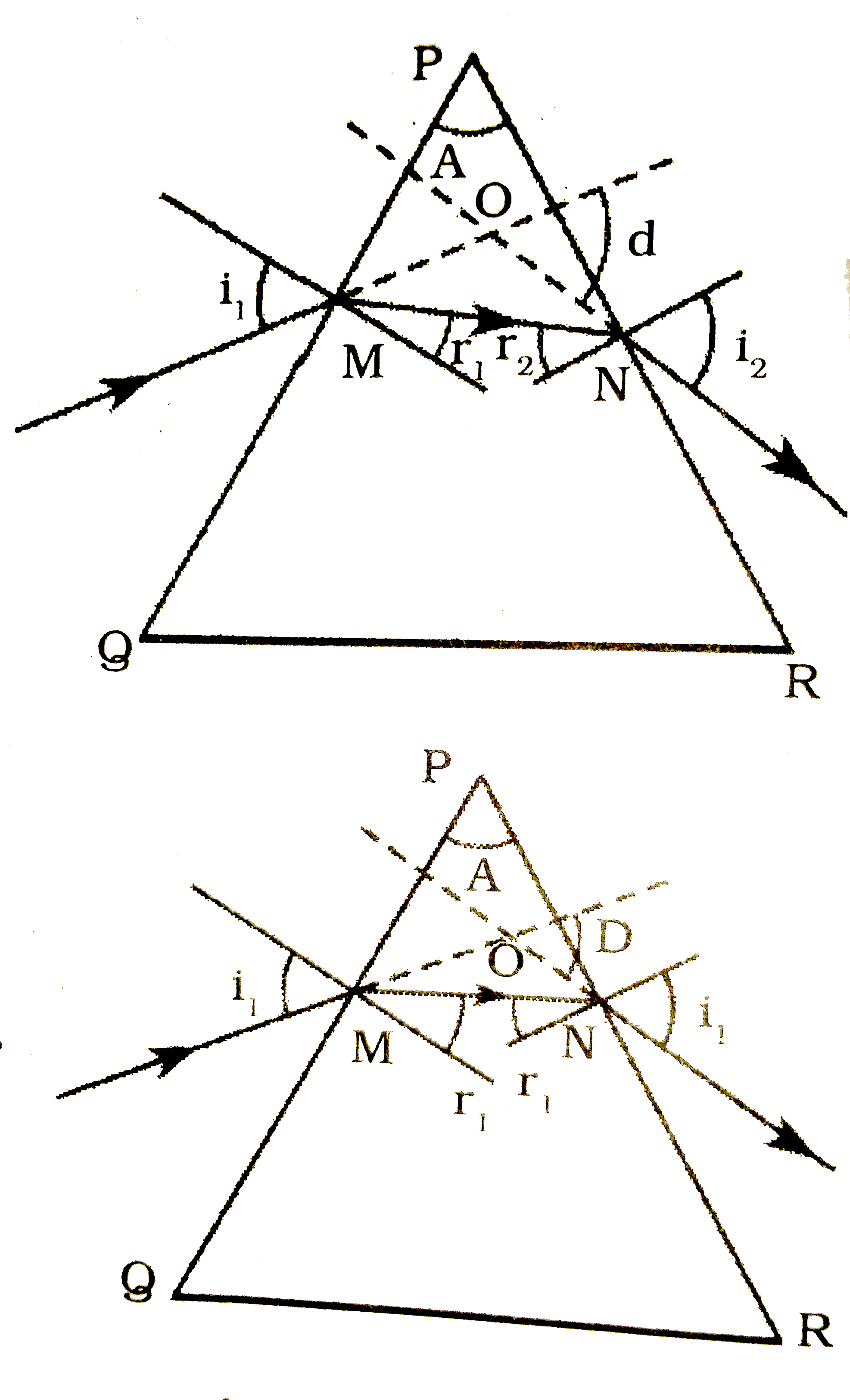
From Snell's law we know that `n_(1)` sin 1=`n_(2)` sin r.
Using Snell's law at M, with refractive index of air, `n_(1)=1, i=i_(1), n_(2) = n, r= r_(1)`
`implies sin i_(1)=n sin i_(2) " "-(4)`
Similarly , at N with `n_(1)=n, i=r_(2), n_(2)=1, r=i_(2)`
`implies n sin r_(2) = sin i_(2)" "-(5)`
We know that at the angle of minimum deviation (D), `i_(1)=i_(2) implies MN "||" QR`
When `i_(1)=i_(2)`, angle of deviation (D) decomes angle of minimum deviation (D).
`therefore (3) implies A + D =2i_(1) implies i_(1)=(A+D)/(2)`
When `i_(2)` , then it is clear that `r_(1)`=r_(2)`
`thererfore (2) implies A=2r_(1) implies r_(1)=(A)/(2)`
Substitute `i_(1) and r_(1)` in (4) , we get sin `((A+D)/(2))` = n sin `A/2`
`therefore n=(sin((A+D)/(2)))/(sin((A)/(2)))`
Where 'n' is the refractive index of the material of the prism .