Let f(x)=x1+x2andg(x)=e−x1+[x] (where [.] denote greatest integer function), then
A
f(x) is monotonically decreasing in [1,3.8]
B
f(x) is monotonically increasing i [1,3.8]
C
the greatest value of f(x) is 13×14.44
D
the least value of f(x) is 2
Video Solution
Text Solution
The correct Answer is:D
y=⎧⎪
⎪
⎪
⎪⎨⎪
⎪
⎪
⎪⎩x2+11≤x<2x2+122≤x<3x2+133≤x≤3.8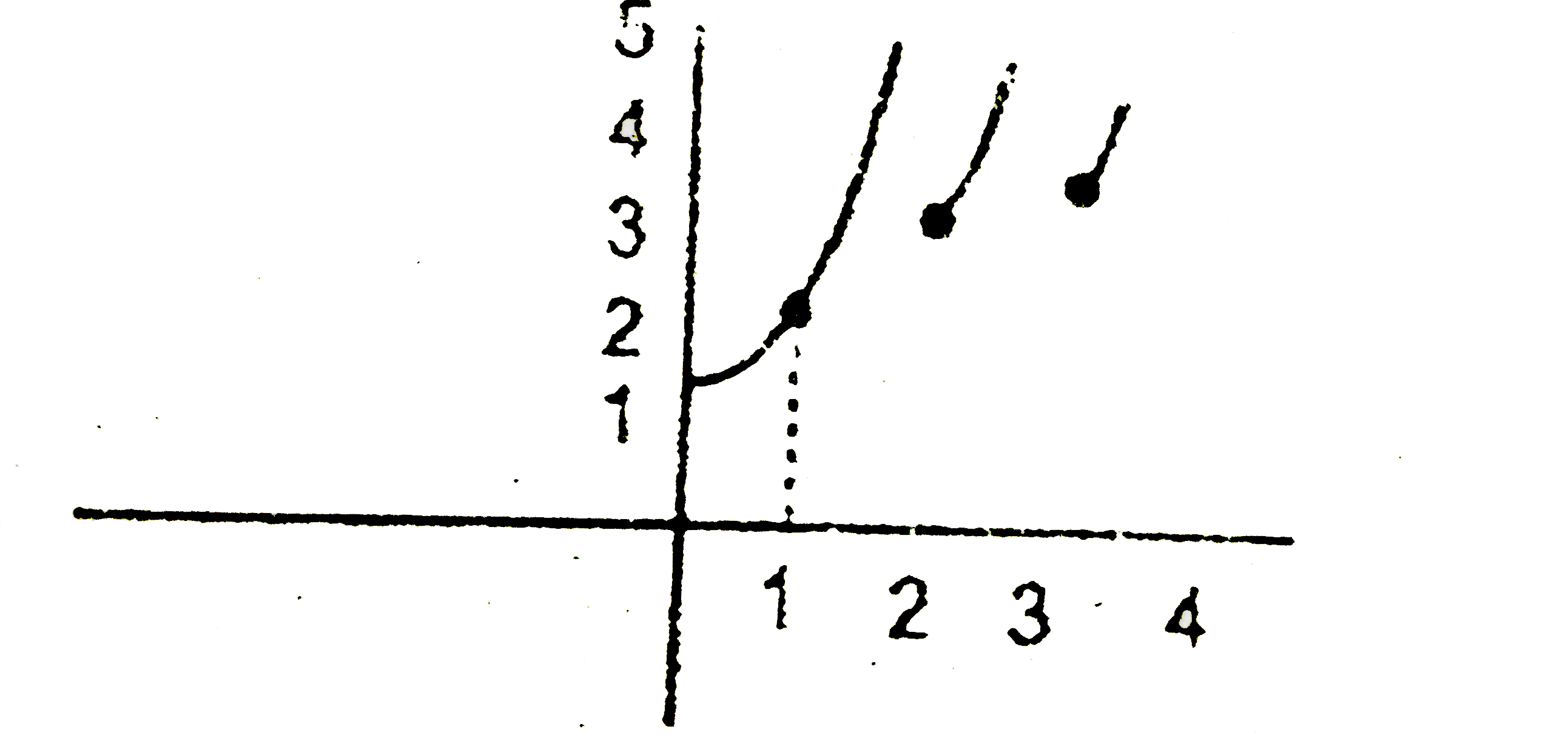 
least value of f(x) is 2
|
Updated on:21/07/2023
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Let f(x)=[|x|] where [.] denotes the greatest integer function, then f'(−1) is
A0B1Cnon-existentDnone of these - Question 2 - Select One
If f(x)=log[x−1](|x|)x,where [.] denotes the greatest integer function,then
AD(f)=[3,∞),R(f)={0,1}BD(f)=[3,∞),R(f)=[3,∞),R(f)={0}CD(f)=(2,∞),R(f)={0,1}DD(f)=(3,∞),R(f)={0} - Question 3 - Select One
If f(x)=[x]|x|,x≠0 where [.] denotes the greatest integer function, then f'(1) is
A−1B1Cnon-existentD∞
If f(x)=x−[x]1−[x]+x, where [.] denotes the greatest integer function, then f(x)∈:
View Solutionf(x)=1+[cosx]x, in 0≤x≤x2 (where [.] denotes greatest integer function)
View Solutionf(x)=1+[cosx]x in 0≤x≤π2 (where [.] denotes greatest integer function)
View SolutionLet Limx→1[x]x=l and limx→1x[x]=m where [ .] denotes the greatest integer function, then
View SolutionLet f(x)=|x|andg(x)=[x], (where [.] denotes the greatest integer function) Then, (fog)'(−1) is
View Solution