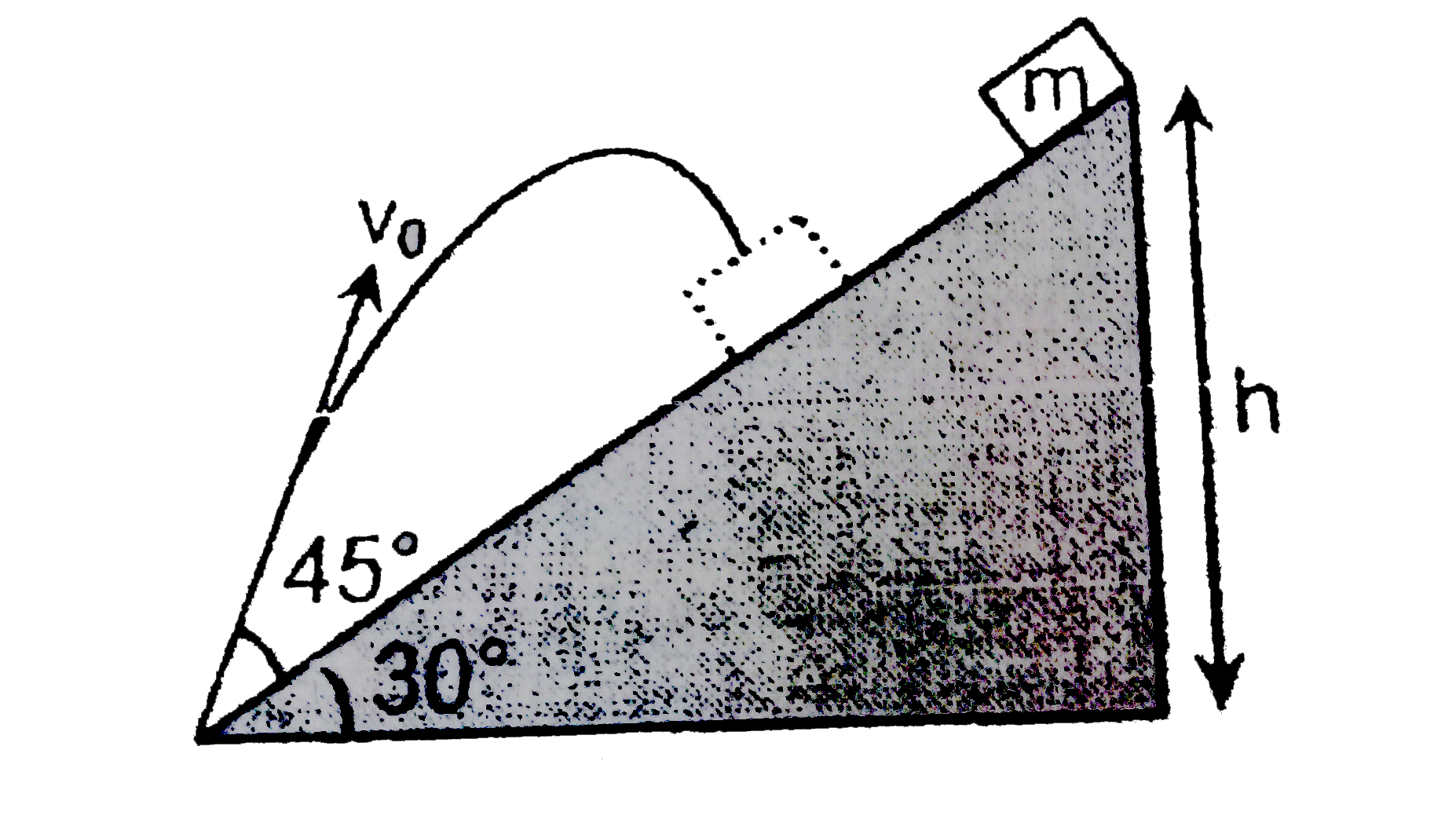
A particle of mass $m$ is projected up from the bottom of an inclined plane with initial velocity $v_{0}$ at angle $45^{\circ}$ with an inclined plane of inclination $30^{\circ}$ as shown in figure. At the same time a small block of same mass $m$ is released from rest at a height $h$ , the particle hits the block at some point on the inclined plane. Neglect friction at inclined.
Q. If the particlee stick to the block after collision the velocity of block parallel tot he inclined plane just after collision will be (take $g = 10 \frac{m}{s^{2}}$

$\frac{v_{0}}{\sqrt{2}}$

$\frac{v_{0}}{2}$

$\frac{v_{0}}{2 \sqrt{2}} (1 - \frac{4}{\sqrt{3}})$

$\frac{v_{0}}{2 \sqrt{2}} (1 + \frac{4}{\sqrt{3}})$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

From conservation of momentum along the inclined plane
$m v_{x} + m v_{x}^{'} = 2 n v_{t}$
$v_{t} = \frac{v_{x} - v_{x}^{'}}{2} = \frac{1}{2} [\frac{v_{0}}{\sqrt{2}} - \frac{g}{2} \frac{2 \sqrt{2} v_{0}}{\sqrt{3} g} - \frac{g}{2} \frac{2 \sqrt{2} v_{0}}{\sqrt{3} g}] = \frac{v_{0}}{2 \sqrt{2}} (1 - \frac{4}{\sqrt{3}})$

Updated on:21/07/2023

Class 12 PHYSICS QUESTION BANK

Knowledge Check

A particle of mass $m$ is projected up from the bottom of an inclined plane with initial velocity $v_{0}$ at angle $45^{\circ}$ with an inclined plane of inclination $30^{\circ}$ as shown in figure. At the same time a small block of same mass $m$ is released from rest at a height $h$ , the particle hits the block at some point on the inclined plane. Neglect friction at inclined.
Q. The value of height h is
A $\frac{v_{0}^{2}}{g}$
B $\frac{\sqrt{3} v_{0}^{2}}{g}$
C $\frac{2 v_{0}^{2}}{\sqrt{3} g}$
D $\frac{v_{0}^{2}}{\sqrt{3} g}$
A particle of mass $m$ is projected up from the bottom of an inclined plane with initial velocity $v_{0}$ at angle $45^{\circ}$ with an inclined plane of inclineation $30^{\circ}$ as shown in figure. At the same time a small block of same mass $m$ is released from rest at a height $h$ . The particle hits the block at some point on inclined plane.

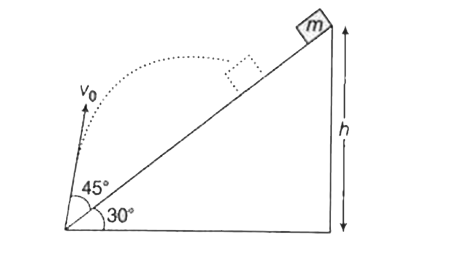
The value of height $h$ is
A $\frac{v_{0}^{2}}{g}$
B $\frac{\sqrt{3} v_{0}^{2}}{g}$
C $\frac{2 v_{0}^{2}}{\sqrt{3} g}$
D $\frac{v_{0}^{2}}{\sqrt{3} g}$
A particle of mass $m$ is projected up from the bottom of an inclined plane with initial velocity $v_{0}$ at angle $45^{\circ}$ with an inclined plane of inclineation $30^{\circ}$ as shown in figure. At the same time a small block of same mass $m$ is released from rest at a height $h$ . The particle hits the block at some point on inclined plane.

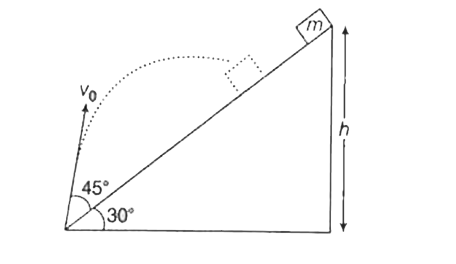
If the particle sticks to the block after collision, the velocity of block parallel to the inclined plane just after collision will be (take $g = 10 m / s^{2}$ )
A $\frac{v_{0}}{\sqrt{2}}$
B $\frac{v_{0}}{2}$
C $\frac{v_{0}}{2 \sqrt{2}} (1 - \frac{4}{\sqrt{3}})$
D $\frac{v_{0}}{2 \sqrt{2}} (1 + \frac{4}{\sqrt{3}})$

The correct Answer is:C

Similar Questions

Knowledge Check

Recommended Questions

The correct Answer is:C

From conservation of momentum along the inclined plane mvx+mv′x=2nvt vt=vx−v′x2=12[v0√2−g22√2v0√3g−g22√2v0√3g]=v02√2(1−4√3)

Similar Questions

Knowledge Check

Recommended Questions