Chemical bond results when between combining atoms :
FA = attraction force
FR=repulsion forces
P.E. = potential energy
FA>FR, P.E. minimum
FA<FR, P.E. maximum
FA=FR,P.E. minimum
FA=FR,P.E. maximum
The correct Answer is:C
Step by step video, text & image solution for Chemical bond results when between combining atoms : F_(A) = attraction force F_(R)=repulsion forces P.E. = potential energy by Chemistry experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CHEMICAL BONDING-IBook:GRB PUBLICATIONChapter:CHEMICAL BONDING-IExercise:C.Identification of Planes; Allenes
Explore 22 Videos
- CHEMICAL BONDING-IBook:GRB PUBLICATIONChapter:CHEMICAL BONDING-IExercise:D.
Explore 54 Videos
- CHEMICAL BONDING-IBook:GRB PUBLICATIONChapter:CHEMICAL BONDING-IExercise:Subjective Type
Explore 120 Videos
- CARBOXYLIC ACID AND DERIVATIVES, AMINES AND OTHER NITROGEN COMPOUNDSBook:GRB PUBLICATIONChapter:CARBOXYLIC ACID AND DERIVATIVES, AMINES AND OTHER NITROGEN COMPOUNDSExercise:Subjective type
Explore 19 Videos
- CHEMICAL KINETICSBook:GRB PUBLICATIONChapter:CHEMICAL KINETICSExercise:Subjective Type
Explore 63 Videos
Similar Questions
Knowledge Check
When total attractive forces between two atoms approaching each other is greater than total repulsive force then, potential energy of the system ________.
AincreasesBremains constantCdecreasesDis zeroWhen a chemical bond between two atom is formed, the potential energy of the system
AdecreasesBincreasesCremains the sameDcannot be predictedFormation of chemical bond between two atoms take place, when net force______ in nature.
AattractiveBrepulsiveCbothDNone
Nature Of Forces|Potential Energy|Relation Between Force And Potential Energy
View SolutionAssertion: Nuclei above bismuth-209 are unstable irrespective o f their N : Z ratio.
Reason: The force of repulsion between protons is greater than the attractive force between nucleons in nuclei above bismuth-209.
View SolutionThe given plot shows the variation of U, the potential energy of interaction between two particles with the distance separating them r,
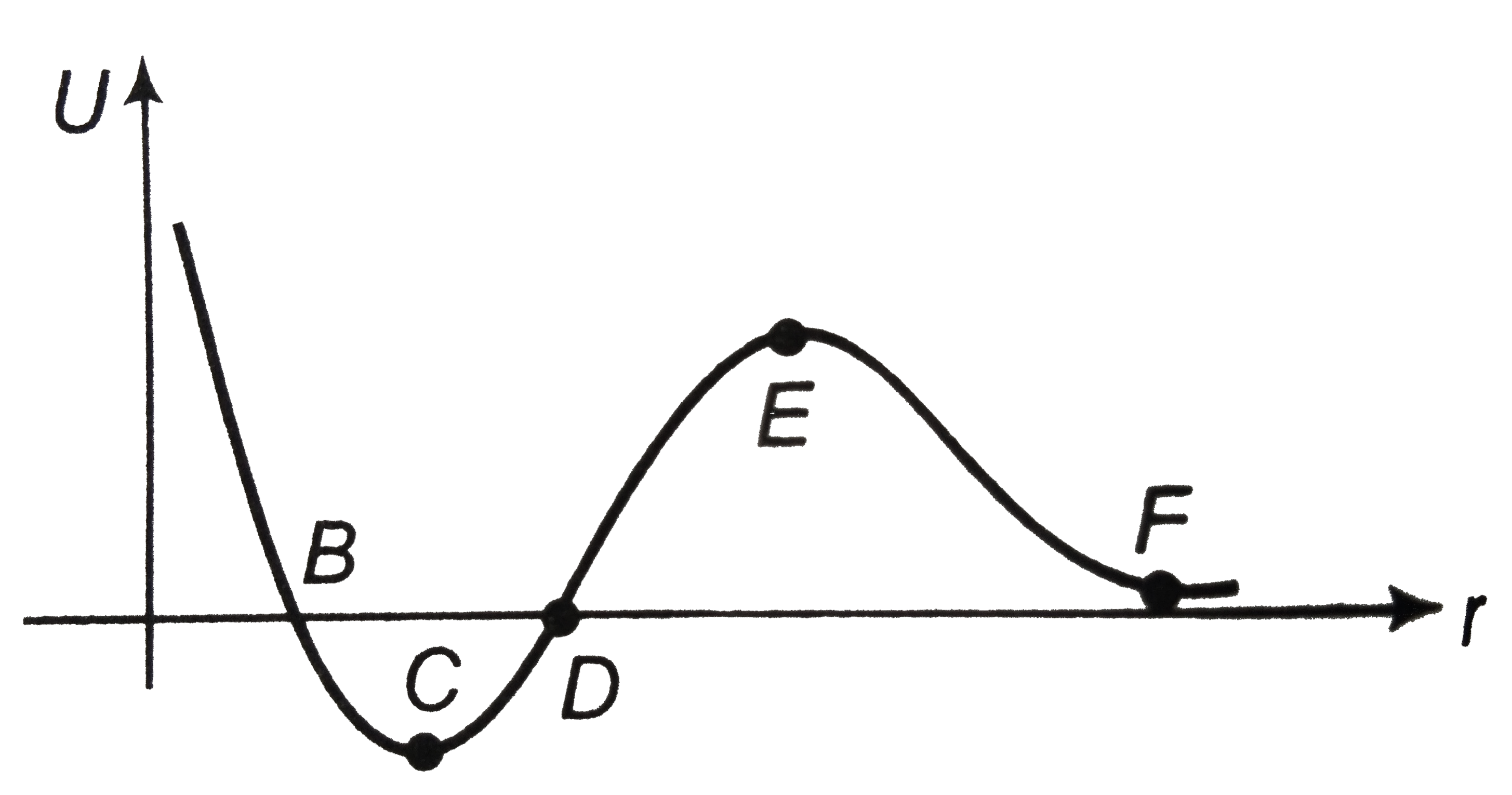
View SolutionIn a conservative force field we can find the radial component of force from the potential energy function by using F=−dUdr. Here, a positive force means repulsion and a negative force means attraction. From the given potential energy function U(r ) we can find the equilibrium position where force is zero. We can also find the ionisation energy which is the work done to move the particle from a certain position to infinity.
Let us consider a case in which a particle is bound to a certain point at a distance r from the centre of the force. The potential energy of the particle is : U(r)=Ar2−Br where r is the distance from the centre of the force and A andB are positive constants. Answer the following questions.
If the total energy of the particle is E=−3B216A, and it is known that the motion is radial only then the velocity is zero at
View SolutionIn a conservative force field we can find the radial component of force from the potential energy function by using F=−dUdr. Here, a positive force means repulsion and a negative force means attraction. From the given potential energy function U(r ) we can find the equilibrium position where force is zero. We can also find the ionisation energy which is the work done to move the particle from a certain position to infinity.
Let us consider a case in which a particle is bound to a certain point at a distance r from the centre of the force. The potential energy of the particle is : U(r)=Ar2−Br where r is the distance from the centre of the force and A andB are positive constants. Answer the following questions.
The work required to move the particle from equilibrium distance to infinity is
View Solution
