In octahedral complex Ma2b2cd, total number of steroisomers is
The correct Answer is:8
To determine the total number of stereoisomers for the octahedral complex Ma2b2cd, we need to analyze the arrangement of the ligands around the central metal atom M.
1. Identify the Coordination Number and Geometry:
- The complex Ma2b2cd has a coordination number of 6, which corresponds to an octahedral geometry.
2. Determine the Types of Ligands:
- The complex consists of two types of bidentate ligands A and B, and two monodentate ligands C and D.
3. Geometric Isomerism:
- In octahedral complexes, geometric isomers can arise from different arrangements of the ligands. For Ma2b2cd, we can have:
- Facial (fac): where the two A ligands and the two B ligands occupy adjacent positions.
- Meridional (mer): where the two A ligands and the two B ligands occupy positions such that they are opposite each other.
4. Counting Geometric Isomers:
- For Ma2b2cd:
- Facial Isomer: The arrangement can be AABBCD.
- Meridional Isomer: The arrangement can be AABCD.
- Thus, there are 2 geometric isomers.
5. Optical Isomerism:
- Optical isomers arise when a complex lacks a plane of symmetry.
- The facial isomer can have optical isomers because it lacks symmetry.
- The meridional isomer typically has a plane of symmetry and does not exhibit optical isomerism.
6. Total Stereoisomers:
- From the facial isomer, we can have 2 optical isomers (enantiomers).
- From the meridional isomer, we have 1 non-optical isomer.
- Therefore, the total number of stereoisomers is 2 (from facial) + 1 (from meridional) = 3 stereoisomers.
Final Answer:
The total number of stereoisomers for the octahedral complex Ma2b2cd is 3.
- The complex Ma2b2cd has a coordination number of 6, which corresponds to an octahedral geometry.
- The complex consists of two types of bidentate ligands A and B, and two monodentate ligands C and D.
- In octahedral complexes, geometric isomers can arise from different arrangements of the ligands. For Ma2b2cd, we can have:
- Facial (fac): where the two A ligands and the two B ligands occupy adjacent positions.
- Meridional (mer): where the two A ligands and the two B ligands occupy positions such that they are opposite each other.
- For Ma2b2cd:
- Facial Isomer: The arrangement can be AABBCD.
- Meridional Isomer: The arrangement can be AABCD.
- Thus, there are 2 geometric isomers.
- Optical isomers arise when a complex lacks a plane of symmetry.
- The facial isomer can have optical isomers because it lacks symmetry.
- The meridional isomer typically has a plane of symmetry and does not exhibit optical isomerism.
- From the facial isomer, we can have 2 optical isomers (enantiomers).
- From the meridional isomer, we have 1 non-optical isomer.
- Therefore, the total number of stereoisomers is 2 (from facial) + 1 (from meridional) = 3 stereoisomers.
The total number of stereoisomers for the octahedral complex Ma2b2cd is 3.
Topper's Solved these Questions
- BOOK - GRB PUBLICATIONCHAPTER - COORDINATION COMPOUNDSEXERCISE - MATCH THE COLUMN TYPE41videos
COORDINATION COMPOUNDS
- BOOK - GRB PUBLICATIONCHAPTER - CHEMICAL KINETICSEXERCISE - Subjective Type63videos
CHEMICAL KINETICS
- BOOK - GRB PUBLICATIONCHAPTER - D-BLOCK ELEMENTSEXERCISE - Subjective Type18videos
D-BLOCK ELEMENTS
Similar Questions
Knowledge Check
- Question 1 - Select One
Co-ordination complexes can show stereoisomerism square planar complexes of Ma2b2 type can show geometrical isomerism but not optical & octahedral complexes of Ma2b2C2,Ma3b3Mabcdef etc show stereoisomerism, for e.g. Mabcdef have 15 geometrical isomers & all are optically active so it has 15 pairs of enantiomers thus in totaly it has 30 stereoisomers. (a,b,c,d etc. are monodentate lignads while AB is bidenatate ligand)
How many pair of enantiomers exits for Ma2b2c2?A0B2C1D4 - Question 2 - Select One
Co-ordination complexes can show stereoisomerism square planar complexes of Ma2b2 type can show geometrical isomerism but not optical & octahedral complexes of Ma2b2C2,Ma3b3Mabcdef etc show stereoisomerism, for e.g. Mabcdef have 15 geometrical isomers & all are optically active so it has 15 pairs of enantiomers thus in totaly it has 30 stereoisomers. (a,b,c,d etc. are monodentate lignads while AB is bidenatate ligand)
Which of the following can show geometrical as well as optical isomerism?AM(AB)3BMabcd (tetrahedral)CMa3b3DMa4b2 - Question 3 - Select One
An octahedral complex having formula Ma2b2C2, which of the following combination is possible to exhibitd optical isomerism
Aa=Cl− B&C=(en)Ba & b & c=EDTACa & b=C2O−24, c=enDa=H2O,b=NH3,C=Cl−
Why does a tetrahedral complex of the type [MA2B2] not show geometrical isomerism ?
View SolutionThe following compounds may exist in two or more stereoisomers
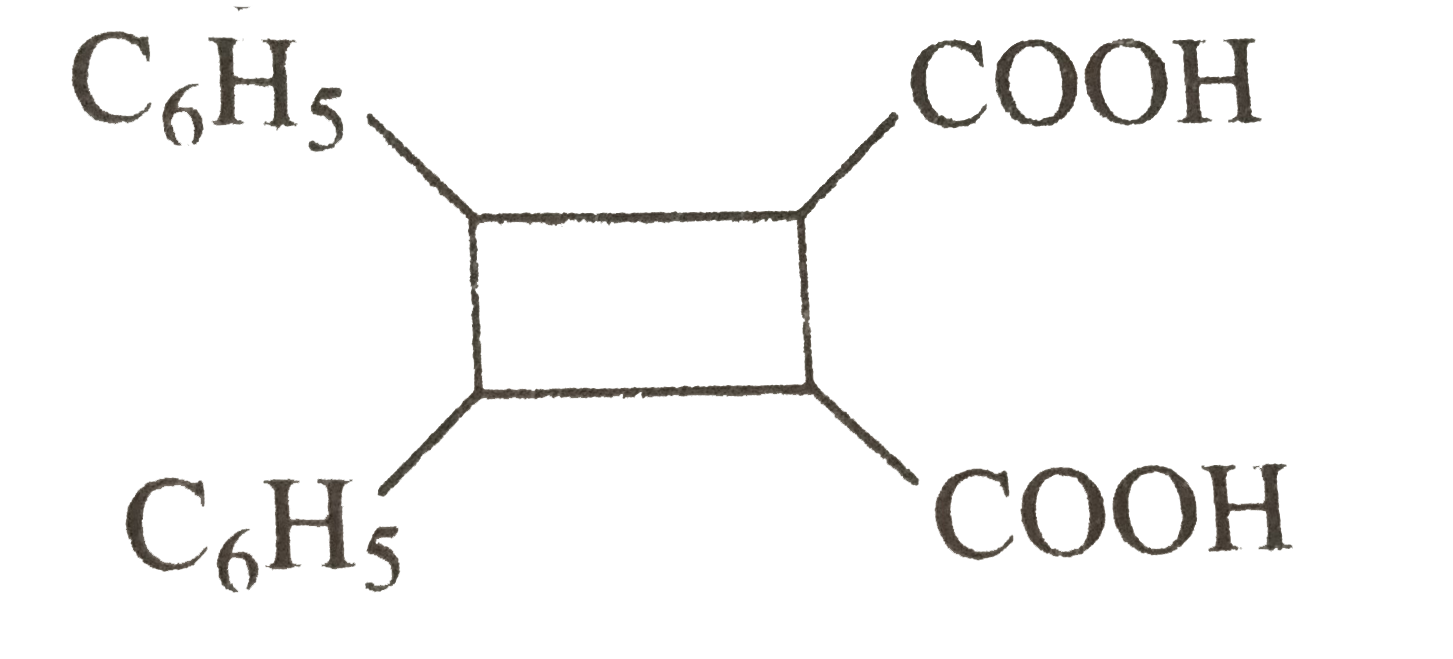
(a) Total number of steroisomers. (b) Number of enantiomeric pairs. ( c) Number of meso compounds.View SolutionWhen the following aldohexose exists in its D-configuration, the total number of stereoisomers in its pyranose form is.
OHC−CH2−CH| OH−CH| OH−CH| OH−CH2−OHView SolutionA metal complex of coordination number six having three different types of ligands a, b and c of composition Ma2b2c2 can exist in several geometrical isomeric forms, the total number of such isomers is:
View SolutionA metal complex of coordination number six having three different types of ligands a, b and c of composition Ma2b2c2 can exist in several geometrical isomeric forms, the total number of such isomers is:
View Solution

