Two substance A2(g) and B2(g) are allowed to react to form either of A2B2(l),A2B4(g) or both depending on relative amount.
Answer the following the following question, if 75 g A2 and 200gB2 are mixed and no reactant is left.
[Given :At mass of A =25 , B=50]
What is the volume of all the gases present in the mixture after completion of reaction at 760 mm of Hg and 273∘ C?
67.2 L
44.8 L
22.4 L
11.2 L
The correct Answer is:C
Step by step video, text & image solution for Two substance A_(2)(g) and B_(2)(g) are allowed to react to form either of A_(2)B_(2)(l), A_(2)B_(4)(g) or both depending on relative amount. Answer the following the following question, if 75 g A_(2) and 200g B_(2) are mixed and no reactant is left. [Given :At mass of A =25 , B=50] What is the volume of all the gases present in the mixture after completion of reaction at 760 mm of Hg and 273^(@) C? by Chemistry experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- MOLE CONCEPT, STOICHIOMETRY & CONCENTRATION TERMSBOOK - GRB PUBLICATIONCHAPTER - MOLE CONCEPT, STOICHIOMETRY & CONCENTRATION TERMSEXERCISE - Comprehension#77
1 Videos
- MOLE CONCEPT, STOICHIOMETRY & CONCENTRATION TERMSBOOK - GRB PUBLICATIONCHAPTER - MOLE CONCEPT, STOICHIOMETRY & CONCENTRATION TERMSEXERCISE - Comprehension#78
1 Videos
- MOLE CONCEPT, STOICHIOMETRY & CONCENTRATION TERMSBOOK - GRB PUBLICATIONCHAPTER - MOLE CONCEPT, STOICHIOMETRY & CONCENTRATION TERMSEXERCISE - Comprehension#75
1 Videos
- ISOMERISMBOOK - GRB PUBLICATIONCHAPTER - ISOMERISMEXERCISE - SUBJECTIVE TYPE
67 Videos
- NOMENCLATURE AND CLASSIFICATIONBOOK - GRB PUBLICATIONCHAPTER - NOMENCLATURE AND CLASSIFICATIONEXERCISE - Subjective Type
24 Videos
Similar Questions
A2+2B2→A2B4and32A2+2B2→A3B4
Two substance A2&B2 are allowed to react completely to from A2B4&A3B4 mixture of leaving none of the reactants. Using this information calculate the composition of final mixture when mentioned amount of A2&B2 are taken.
(i) If 54 moles of A2&2 moles of B2 is taken
(ii) If 72 moles of A2&6 moles of B2 is taken
(iii) If 4 moles of A2&6 moles of B2 is taken
View SolutionSubstance A2B(g) can undergo decomposition to form of set of products :
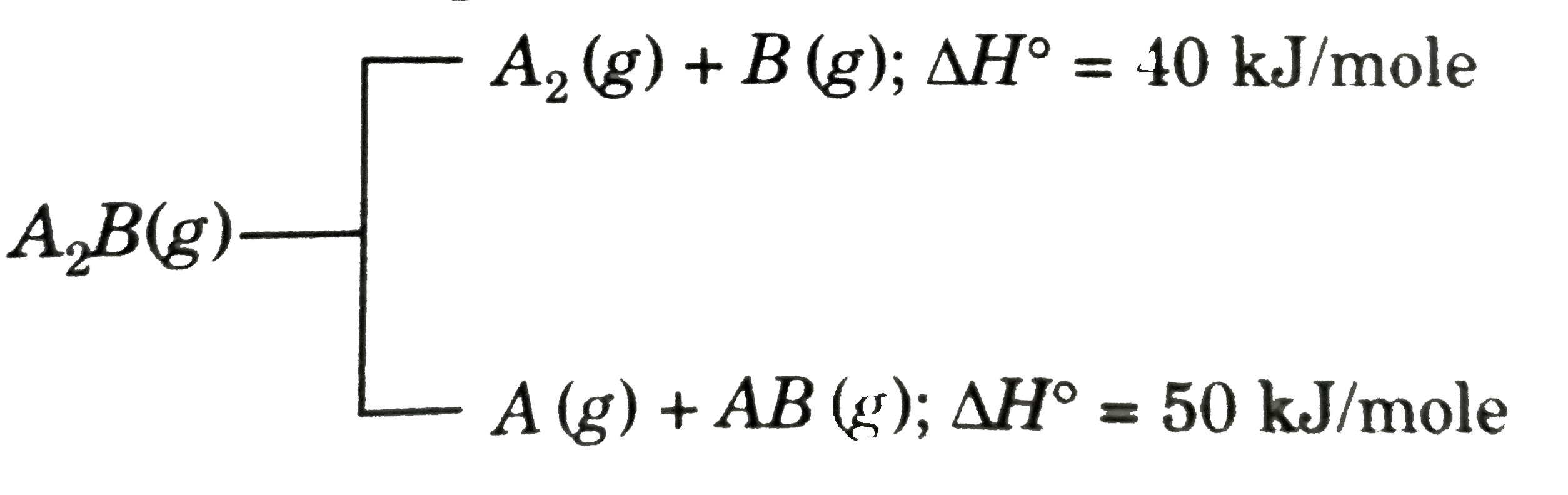
if the molar ratio of A2(g) to A(g) is 5:3 in a set of product gases, then energy involved in the decomposition of 1 mole of A2B is :
View Solution
Knowledge Check
Two substance A2(g) and B2(g) are allowed to react to form either of A2B2(l),A2B4(g) or both depending on relative amount.
Answer the following the following question, if 75 g A2 and 200gB2 are mixed and no reactant is left.
[Given :At mass of A =25 , B=50]
Now A2B4 is transferred to another container completey . At high temperature it is completely dissociated in its gaseous elements A2 and B2 . Find average molecular weight of gaseous mixture:A1253B2503C125D250In a closed vessel, 5 moles of A2(g) and 7 moles of B2 (g) are reacted in the following maner,
A2(g)+(3B2(g)→2AB3(g)
What is the total number of moles of gases present in the container at the end of the reaction?A22/3B7/3C14/3D8/3For the reaction A(g)⇔B(g)+C(g)
AKp=α3PBKp=α2(Kp+P+1)CKp=α2(Kp+P)DKp=α2(KP+PP)
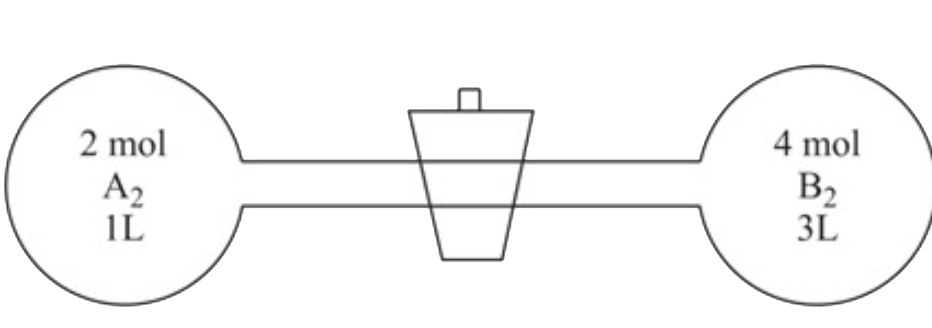
When A2andB2 are allowed to react, the equilibrium constant of the reaction at 27∘C is found (KC=4).A2(g)+B2(g)⇔2AB(g)
What will be the equilibrium concentration of AB?
View SolutionTe yield of product in the reaction
A2(g)+B2(g)⇔2AB(g),ΔH=+ve
View SolutionIn a reaction A2(g)+4B2(g)⇔2AB4(g),ΔH<0. The formation of AB4 is not favoured by
View SolutionSubstance A2B(g) can undergoes decomposition to form two set of products :
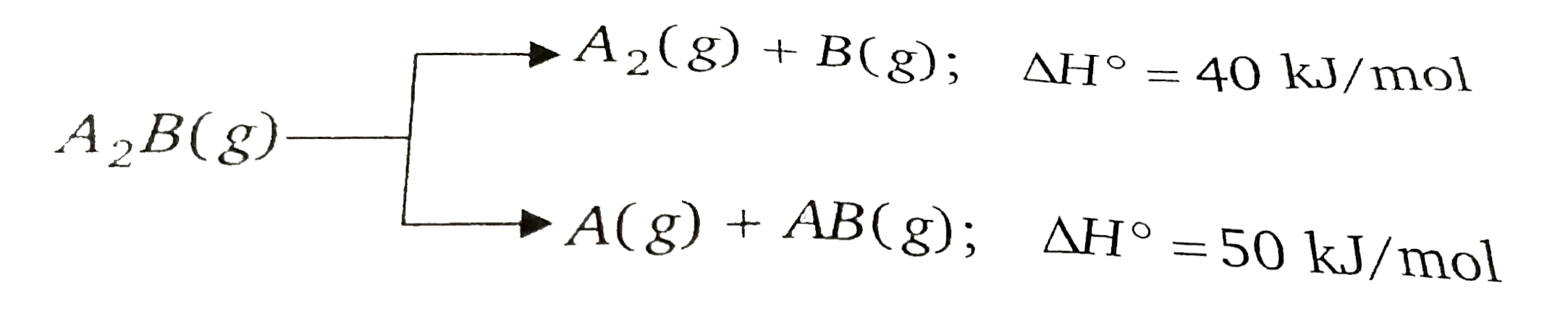
If the molar ratio of A2(g) to A(g) is 5:3 in a set of product gases, then the energy involved in the decomposition of 1 mole of A2B(g) is :
View SolutionSubstance A2B(g) can undergoes decomposition to form two set of products :
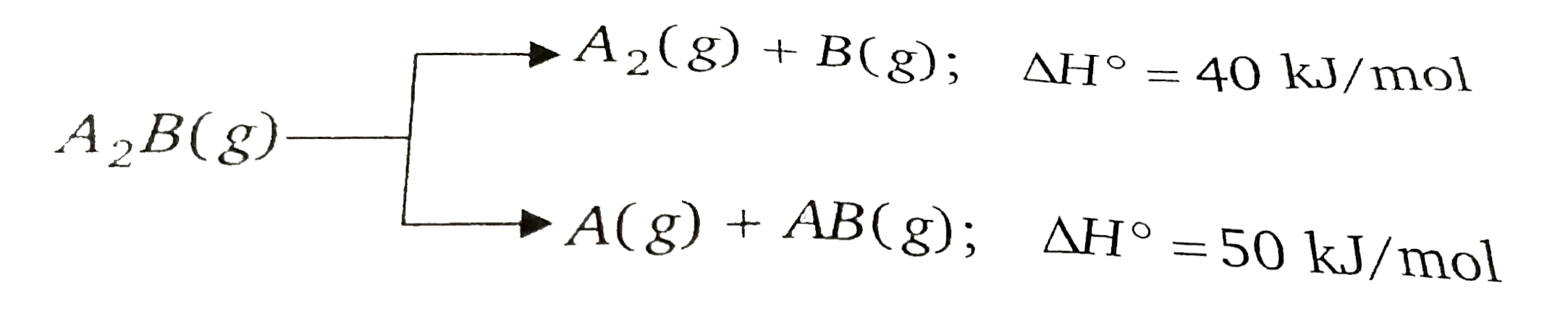
If the molar ratio of A2(g) to A(g) is 5:3 in a set of product gases, then the energy involved in the decomposition of 1 mole of A2B(g) is :
View Solution
