The number of moles of C and D produced on mixing 5 moles of A and 7 moles of B are respectively:
(3A+5B⇒7C+9D)
9 moles and 11 moles
11.66 moles and 15 moles
9.8 moles and 12.6 moles
1 mole and 13 moles
The correct Answer is:C
To solve the problem, we need to determine the number of moles of C and D produced when 5 moles of A and 7 moles of B are mixed, based on the given reaction:
3A+5B→7C+9D
Step 1: Identify the limiting reagent
First, we need to find out which reactant is the limiting reagent. The stoichiometry of the reaction tells us that:
- 3 moles of A react with 5 moles of B.
To find out how much A is needed for 7 moles of B:
- From the reaction, 5 moles of B require 3 moles of A.
- Therefore, 1 mole of B requires 35 moles of A.
- For 7 moles of B, the amount of A required is:
A required=7×35=215=4.2 moles of A
Since we have 5 moles of A available, A is in excess, and B is the limiting reagent.
Step 2: Calculate the moles of C produced
Next, we calculate how many moles of C are produced from the limiting reagent (B):
- According to the reaction, 5 moles of B produce 7 moles of C.
- Therefore, for 7 moles of B, the amount of C produced is:
C produced=7×75=495=9.8 moles of C
Step 3: Calculate the moles of D produced
Now, we calculate how many moles of D are produced from the limiting reagent (B):
- According to the reaction, 5 moles of B produce 9 moles of D.
- Therefore, for 7 moles of B, the amount of D produced is:
D produced=7×95=635=12.6 moles of D
Final Answer
Thus, the number of moles of C and D produced are:
- Moles of C = 9.8
- Moles of D = 12.6
First, we need to find out which reactant is the limiting reagent. The stoichiometry of the reaction tells us that:
- 3 moles of A react with 5 moles of B.
- From the reaction, 5 moles of B require 3 moles of A.
- Therefore, 1 mole of B requires 35 moles of A.
- For 7 moles of B, the amount of A required is:
A required=7×35=215=4.2 moles of A
Next, we calculate how many moles of C are produced from the limiting reagent (B):
- According to the reaction, 5 moles of B produce 7 moles of C.
- Therefore, for 7 moles of B, the amount of C produced is:
Now, we calculate how many moles of D are produced from the limiting reagent (B):
- According to the reaction, 5 moles of B produce 9 moles of D.
- Therefore, for 7 moles of B, the amount of D produced is:
Thus, the number of moles of C and D produced are:
- Moles of C = 9.8
- Moles of D = 12.6
Topper's Solved these Questions
- MOLE CONCEPT, STOICHIOMETRY & CONCENTRATION TERMSBOOK - GRB PUBLICATIONCHAPTER - MOLE CONCEPT, STOICHIOMETRY & CONCENTRATION TERMSEXERCISE - Percentage yield and percentage purity
18 Videos
- MOLE CONCEPT, STOICHIOMETRY & CONCENTRATION TERMSBOOK - GRB PUBLICATIONCHAPTER - MOLE CONCEPT, STOICHIOMETRY & CONCENTRATION TERMSEXERCISE - Sequential and Parallel Reactions
17 Videos
- MOLE CONCEPT, STOICHIOMETRY & CONCENTRATION TERMSBOOK - GRB PUBLICATIONCHAPTER - MOLE CONCEPT, STOICHIOMETRY & CONCENTRATION TERMSEXERCISE - Density
36 Videos
- ISOMERISMBOOK - GRB PUBLICATIONCHAPTER - ISOMERISMEXERCISE - SUBJECTIVE TYPE
67 Videos
- NOMENCLATURE AND CLASSIFICATIONBOOK - GRB PUBLICATIONCHAPTER - NOMENCLATURE AND CLASSIFICATIONEXERCISE - Subjective Type
24 Videos
Similar Questions
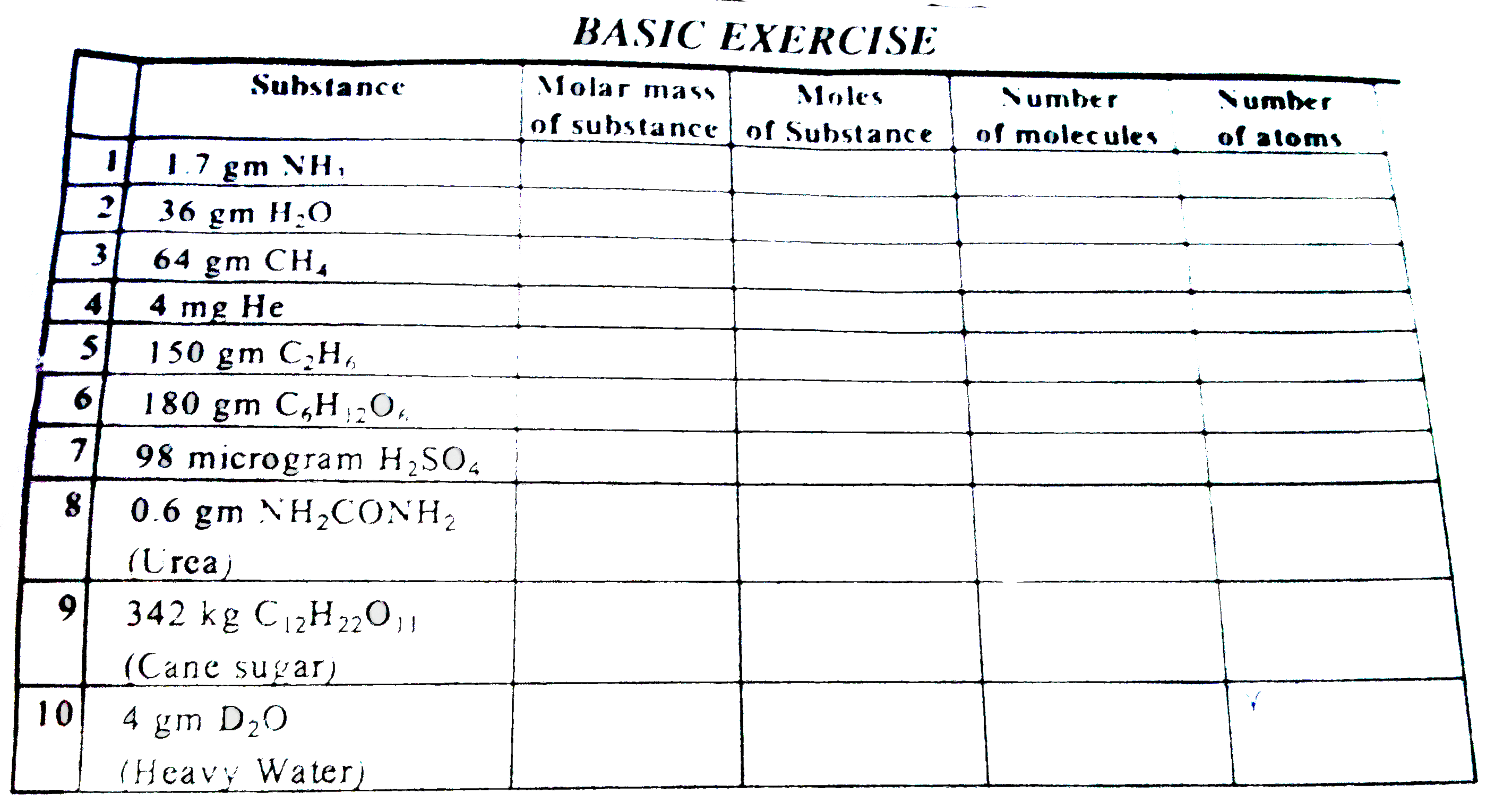
A→2B+C
3B→2D
Find moles of D produced if initially 3 moles of A are taken.
View Solution9 moles of "D" and 14 moles of E are allowed to react in a closed vessel according to given reactions. Calculate number of moles of B formed in the end of reaction, if 4 moles of G are present in reaction vessel. (percentage yield of reaction is mentioned in the reaction) Step -1 3D+4E80%→5C+A Step-2 3C+5G50%→6B+F
View Solution
Knowledge Check
For the reaction A + 2B → C, 5 moles of A and B moles of B will produce
A5 moles of CB4 moles of CC8 moles of CD13 moles of CFor the reaction A+2B→C,5moles of A and 8moles of B will produce :-
A5 moles of CB4 moles of CC8 moles of CD13 moles of CFor the reaction A+2B→C, 5 moles of A and 8 moles of B will produce
A5 moles of CB4 moles of CC8 moles of CD13 moles of C
For the reaction, X+2Y→Z
5 moles of X and 9 moles of Y will produce:
View SolutionIn the reaction 4A+2B+3C→A4B2C3, the number of moles of product formed will be if starting from 2 moles of A, 1.2 moles of B and 1.44 moles of C
View SolutionIn the reaction 4A+2B+3C⇒A4B2C3 what will be the number of moles of product formed ? Starting from 2 moles of A, 1.2 moles of B and 1.44 moles of C.
View SolutionIn the reaction 4A+2B+3C→A4B2C3 what will bw the number of moles of product formed. Starting from 2 moles of A,1.2 moles of B&1.44 moles of C:
View SolutionStatement-1: 2A+3B→C
4/3 moles of 'C' are always produced when 3 moles of 'A' & 4 moles of 'B' are added.
Statement-2: 'B'is the limiting reactant for the given data.
View Solution
