Assertion: In is dimensionless.
Reason: Because dimension of .
If both assertion and reason are true and the reason is the correct explanation of the assertion.
If both assertion and reason are true but reason is not the correct explanation of the assertion.
If assertion is true but reason is false.
If the assertion and reason both are false.
The correct Answer is:C
Step by step video, text & image solution for Assertion: In y = A sin (omega t - kx),(omega t - kx) is dimensionless. Reason: Because dimension of omega = [M^(0)L^(0)T]. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
In the relation y=rsin(ωt−kx), the dimensions of ω/k are
A[M0L0T0]B[M0L1T−1]C[M0L0T1]D[M0L1T0]if y=Asin(ωt−kx), then the value of dydx is
AAcos(ωt−kx)B−Aωcos(ωt−kx)CAKcos(ωt−kx)D−AKcos(ωt−kx)
(a) the displacement of a particle is given by s−asin(ωt=kx), where t is in second and x is in meter. Find the dimensions of ω and k.
(b) The velocity of a praticle is given by v=v0e−λt, where t is time. Find the dimensions of v0 and λ.
View SolutionIn the expression y=asin(ωt+θ), y is the displacement and t is the time . Write the dimension ofa,ωand θ .
View SolutionIn y= A sin ωt + A sin (ωt+(2π)/3) match the following table.
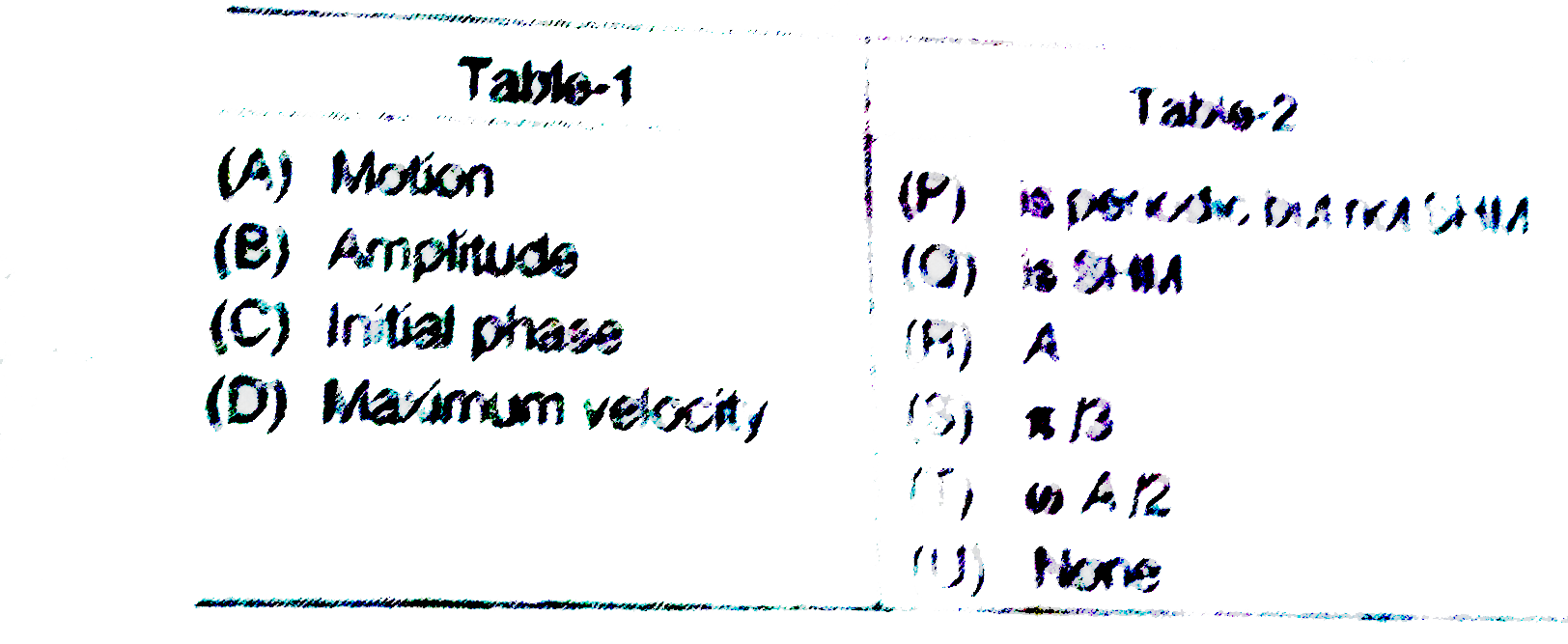
View SolutionIn the equation y= a sin (ωt+kx) t and x stand for time and distance respectively. What are the dimensions of ω/k?
View SolutionIn the relation x=cos(ωt+kx), the dimension(s) of ω is/are
View SolutionIn the equation y=Asin(ωt−kx), obtain the dimensional formula of ωandk. Given x is distnace and t is time.
View Solution
