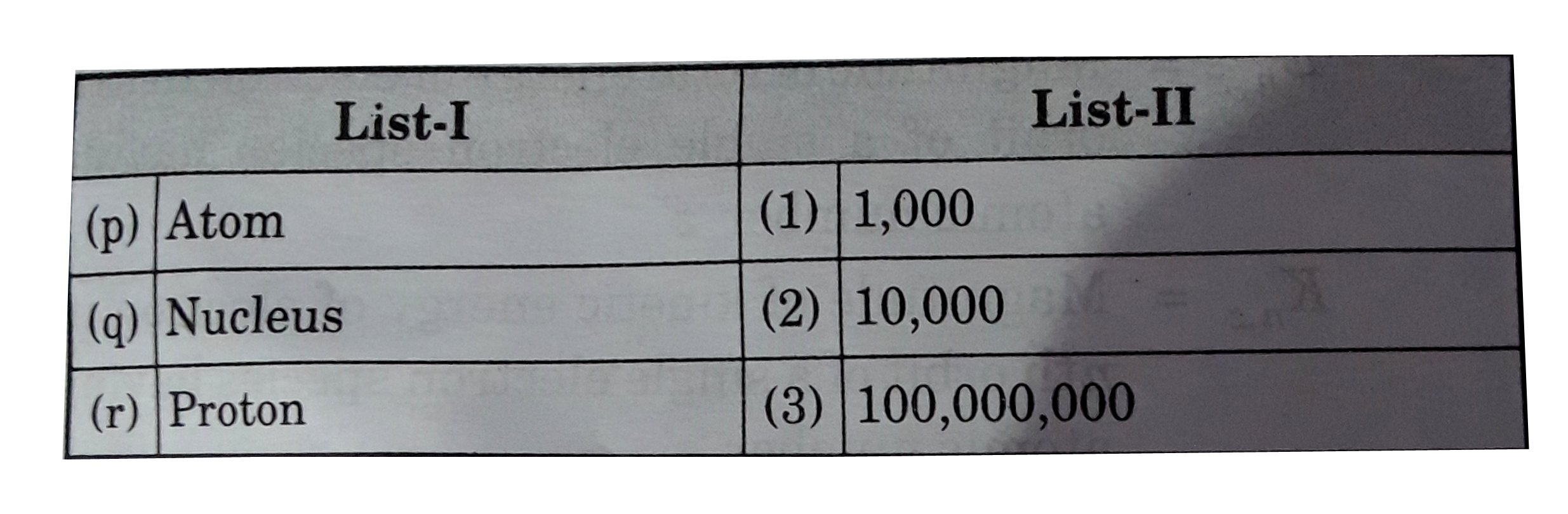
In a scale of 10−18m, match the particle with respect to their probable size:
Codes :
p q r " "p q r
1 2 3
3 2 1
1 3 2
2 3 1
The correct Answer is:(b)
Step by step video, text & image solution for In a scale of 10^(-18)m, match the particle with respect to their probable size: Codes : p q r " "p q r by Chemistry experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ATOMIC STRUCTUREBook:GRB PUBLICATIONChapter:ATOMIC STRUCTUREExercise:Subjective Type
Explore 55 Videos
- ATOMIC STRUCTUREBook:GRB PUBLICATIONChapter:ATOMIC STRUCTUREExercise:Comprehesion Type
Explore 38 Videos
- CHEMICAL EQUILIBRIUMBook:GRB PUBLICATIONChapter:CHEMICAL EQUILIBRIUMExercise:D.Le Chateliera principle
Explore 16 Videos
Similar Questions
Let p,q r be three statements such that the truth value of (p∧q)→(q∨r) is F. Then the truth value of p,q r are respectively :
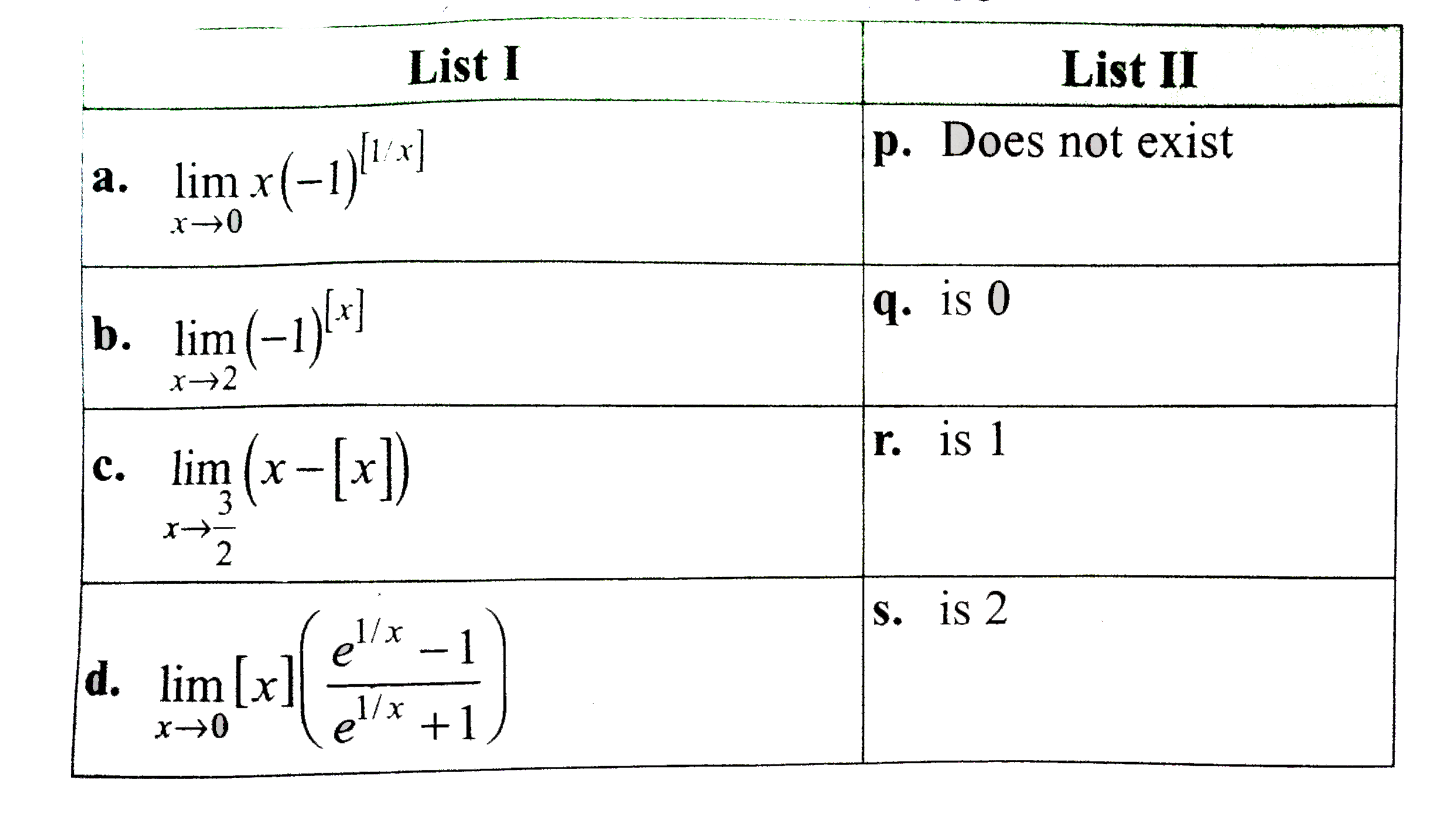
View SolutionMatch the following lists (where [x] represents the greatest integer function) and then choose the correct code.
Codes :
a b c d(1)s r q p(2)q p s p(3)s r p q(4)p p q r
View Solution
Knowledge Check
If the shortest wavelength of spectral line of H-atom in Lyman series is x, then match the following for Li2+ and select the correct code.
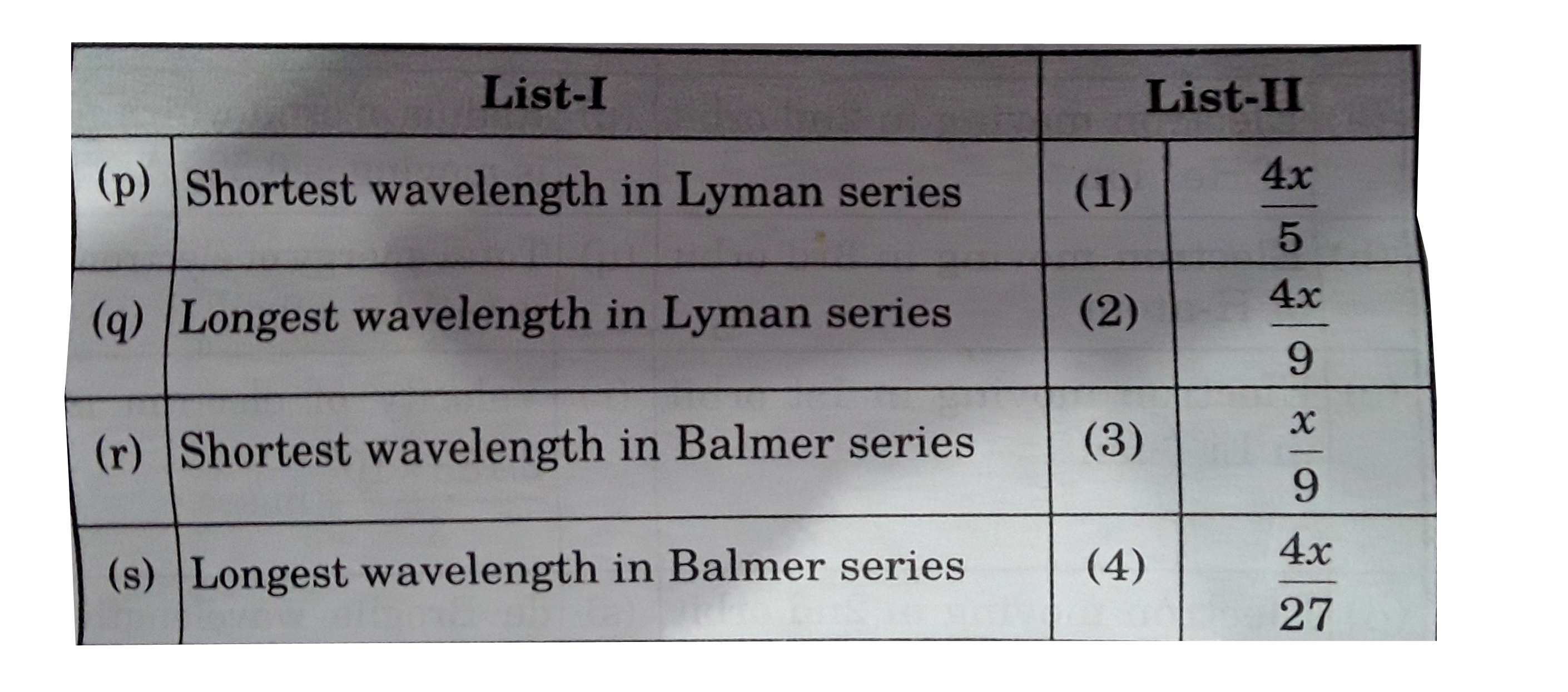
Codes :
p q r s " "p q r sA2 4 1 3B3 4 2 1C3 4 1 2D2 1 4 3If (p∧~q)→(~p∨r) is a false statement, then respective truth values of p, q and r are
AT,F,FBF, T,TCT, T, TDF,F, FLet p, q and r be three statements, then
[p→(q→r)]↔[(p∧q)→r], is aAtautologyBcontradictionCfallacyDnone of these
In Figure, ∠QPR=∠PQR and M and N are respectively on sides QR and PR of PQR such that QM=PN . Prove that OP=OQ, where O is the point of intersection of PM and QN.

View SolutionIn PQR , M and N are points on sides PQ and PR respectively such that PM=15cm and NR=8cm . If PQ=25cm and PR=20cm state whether MNQR .
View SolutionIf p, q and r are perpendicular to q + r, r + p and p + q respectively and if |p + q| = 6, |q + r| = 4√3 and |r + p| = 4, then |p + q + r| is
View SolutionIf p, q and r are perpendicular to q + r, r + p and p + q respectively and if |p + q| = 6, |q + r| = 4√3 and |r + p| = 4, then |p + q + r| is
View SolutionTwo particles p and q located at distances rp and rq respectively from the centre of a rotating disc such that rp>rq.
View Solution