A vessel of area of cross-section A has liquid to a height H . There is a hole at the bottom of vessel having area of cross-section a . The time taken to decrease the level from H1 to H2 will sec
Aa√2g[√H1−√H2]
√2gh
√2gh(H1−H2)
Aa√g2[√H1−√H2
The correct Answer is:A
To solve the problem of finding the time taken for the liquid level in a vessel to decrease from height H1 to H2 through a hole at the bottom, we can use Torricelli's law and the principles of fluid dynamics. Here’s the step-by-step solution:
Step 1: Understand the flow rate
According to Torricelli's law, the speed v of efflux of a fluid under the force of gravity through a hole is given by:
v=√2gH
where g is the acceleration due to gravity and H is the height of the liquid above the hole.
Step 2: Determine the volume flow rate
The volume flow rate Q through the hole can be expressed as:
Q=a⋅v=a⋅√2gH
where a is the area of cross-section of the hole.
Step 3: Relate the change in height to the volume
The change in volume dV of the liquid in the vessel as the height decreases by dH is given by:
dV=A⋅dH
where A is the area of cross-section of the vessel.
Step 4: Set up the differential equation
Since the volume flow rate out of the vessel equals the rate of change of volume in the vessel, we can write:
AdHdt=−a√2gH
The negative sign indicates that the height H is decreasing.
Step 5: Rearranging the equation
Rearranging gives:
dH√H=−a√2gAdt
Step 6: Integrate both sides
Integrating both sides, we have:
∫H2H1dH√H=−a√2gA∫t0dt
This leads to:
2(√H2−√H1)=−a√2gAt
Step 7: Solve for time t
Rearranging for t gives:
t=−2Aa√2g(√H2−√H1)
Final Result
Thus, the time taken to decrease the liquid level from H1 to H2 is:
t=2Aa√2g(√H1−√H2)
According to Torricelli's law, the speed v of efflux of a fluid under the force of gravity through a hole is given by:
v=√2gH
where g is the acceleration due to gravity and H is the height of the liquid above the hole.
The volume flow rate Q through the hole can be expressed as:
Q=a⋅v=a⋅√2gH
where a is the area of cross-section of the hole.
The change in volume dV of the liquid in the vessel as the height decreases by dH is given by:
dV=A⋅dH
where A is the area of cross-section of the vessel.
Since the volume flow rate out of the vessel equals the rate of change of volume in the vessel, we can write:
AdHdt=−a√2gH
The negative sign indicates that the height H is decreasing.
Rearranging gives:
dH√H=−a√2gAdt
Integrating both sides, we have:
∫H2H1dH√H=−a√2gA∫t0dt
This leads to:
2(√H2−√H1)=−a√2gAt
Rearranging for t gives:
t=−2Aa√2g(√H2−√H1)
Thus, the time taken to decrease the liquid level from H1 to H2 is:
t=2Aa√2g(√H1−√H2)
Topper's Solved these Questions
- FLUID MECHANICSBOOK - ERRORLESS CHAPTER - FLUID MECHANICSEXERCISE - Graphical Questions
5 Videos
- FLUID MECHANICSBOOK - ERRORLESS CHAPTER - FLUID MECHANICSEXERCISE - Asseration and Reason
28 Videos
- FLUID MECHANICSBOOK - ERRORLESS CHAPTER - FLUID MECHANICSEXERCISE - Fluid Laq
66 Videos
- ELASTICITYBOOK - ERRORLESS CHAPTER - ELASTICITYEXERCISE - Self Evaluation Test
11 Videos
- FRICTIONBOOK - ERRORLESS CHAPTER - FRICTIONEXERCISE - MCQ S
125 Videos
Similar Questions
A cylindrical vessel of area of cross section A is filled with a liquid up to a height H. A very small hole of area of cross section a is made at the bottom of the vellel. Find the time taken by the vessel to become empty.
View SolutionA conducting liquid is filled in a vessel of area of cross section A upto height h then resistance of the liquid is
View Solution
Knowledge Check
A cylindrical vessel of cross section A contains water to a height h. There is a hole in the bottom of radius 'a'. The time in which it will be emptied is:
A2Aπa2√hgB√2Aπa2√hgC2√2Aπa2√hgDA√2πa2√hgA cylindrical vessel contains a liquid of density ρ up to height h. The liquid is closed by a piston of mass m and area of cross section A. There is a small hole at the bottom of the vessel. The speed v with which the liquid comes out of the hole is
 A√2ghB√2(gh+mgρA)C√2(gh+mgA)D√2(gh+mgA)
A√2ghB√2(gh+mgρA)C√2(gh+mgA)D√2(gh+mgA)A wide tank of cross-section area A, containing a liquid to a height H, has an orifice at its base of areaA3 . The initial velocity of top surface of liquid is
A√gH4B3√gH4C√gH2D13√gH2
A cylindrical vessel contains a liquid of density ρ up to height h. The liquid is closed by a piston of mass m and area of cross section A. There is a small hole at the bottom of the vessel. The speed v with which the liquid comes out of the hole is

View SolutionA resistor of length L and area of cross-section A has a resistance R. Another resistors, of the same material, of length L2 and area of cross-section, 2A will have a resistance of ________.
View SolutionA cylindrical vessel of area of cross-section A and filled with liquid to a height of h1 has a capillary tube of length l and radius r protuding horizontally at its bottom. If the viscosity of liquyid is η and density ρ. Find the time in which the level of water in the vessel falls to h2.
View Solution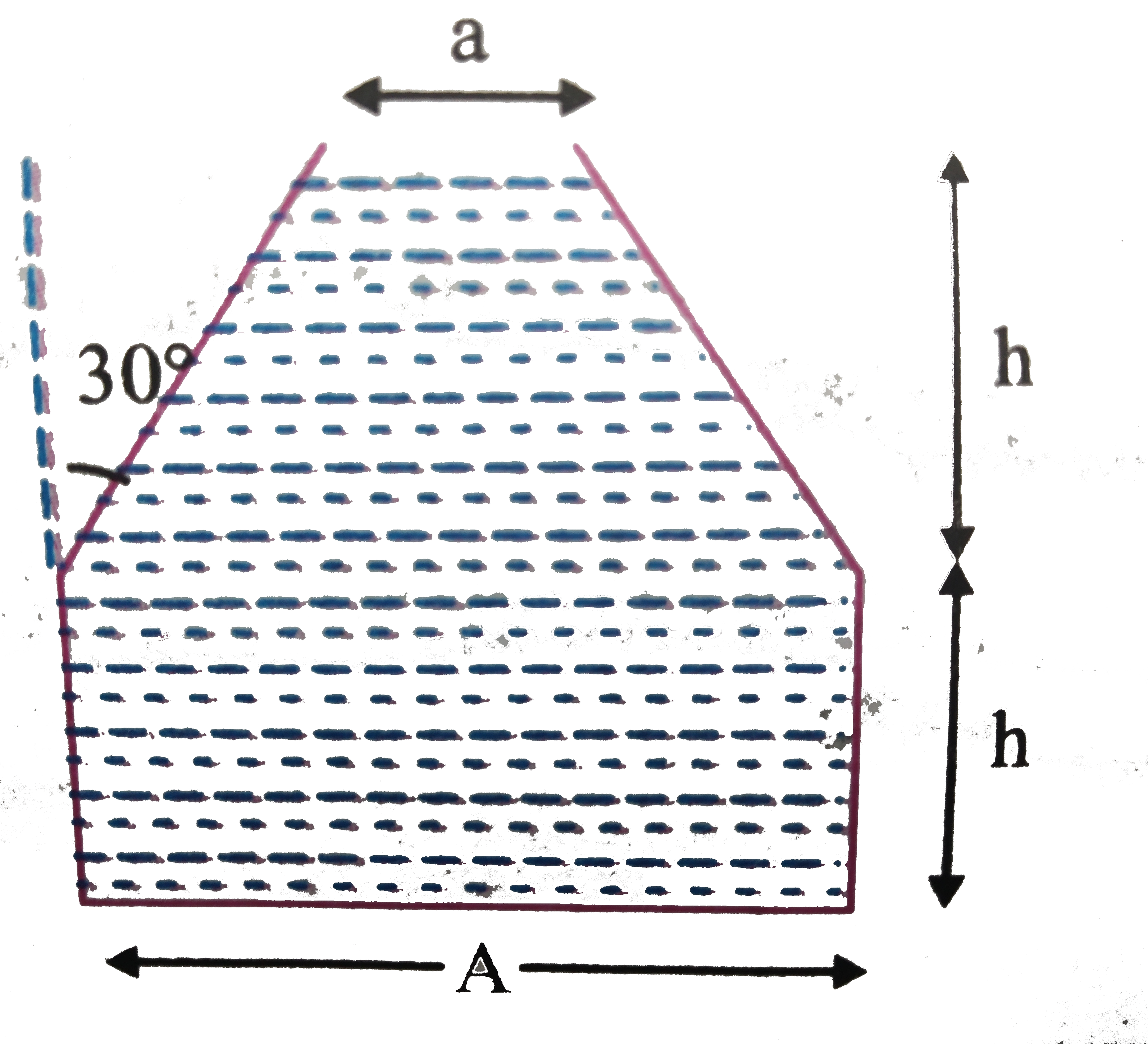
The vessel shown in the figure has two sections. The lower part is a rectangular vessel with area of cross-section A and height h. The upper part is a conical vessel of height h with base area A and top area a and the wals of the vessel are inclined at an angle 30∘ witht he vertical.A liquid of density ρ fills both the sections upto a height 2h. Neglect atmospheric pressure.
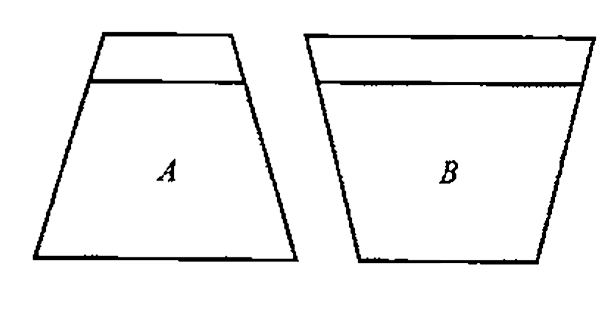
View SolutionTwo vessels A and B of cross sections as shown contain a liquid up to the same height. As the temperature rises, the liquid pressure at the bottom (neglecting expansion of the vessels ) will :
View Solution
