The velocity of kerosene oil in a horizontal pipe is 5m/s. If g=10m/s2 then the velocity head of oil wlill be
1.25m
12.5m
0.125m
125m
The correct Answer is:A
To find the velocity head of kerosene oil in a horizontal pipe, we can use the formula for velocity head, which is given by:
Velocity Head=v22g
where:
- v is the velocity of the fluid (in m/s),
- g is the acceleration due to gravity (in m/s²).
1. Identify the given values:
- Velocity of kerosene oil, v=5m/s
- Acceleration due to gravity, g=10m/s2
2. Substitute the values into the formula:
Velocity Head=(5m/s)22×10m/s2
3. Calculate (5m/s)2:
(5m/s)2=25m2/s2
4. Multiply 2×10m/s2:
2×10m/s2=20m/s2
5. Divide the two results:
Velocity Head=25m2/s220m/s2=1.25m
Final Answer:
The velocity head of kerosene oil will be 1.25m.
---
- v is the velocity of the fluid (in m/s),
- g is the acceleration due to gravity (in m/s²).
- Velocity of kerosene oil, v=5m/s
- Acceleration due to gravity, g=10m/s2
Velocity Head=(5m/s)22×10m/s2
(5m/s)2=25m2/s2
2×10m/s2=20m/s2
Velocity Head=25m2/s220m/s2=1.25m
The velocity head of kerosene oil will be 1.25m.
Topper's Solved these Questions
- FLUID MECHANICSBOOK - ERRORLESS CHAPTER - FLUID MECHANICSEXERCISE - Critical Thinking
14 Videos
- FLUID MECHANICSBOOK - ERRORLESS CHAPTER - FLUID MECHANICSEXERCISE - Graphical Questions
5 Videos
- FLUID MECHANICSBOOK - ERRORLESS CHAPTER - FLUID MECHANICSEXERCISE - Pascal s Law and Archmidies Principle
27 Videos
- ELASTICITYBOOK - ERRORLESS CHAPTER - ELASTICITYEXERCISE - Self Evaluation Test
11 Videos
- FRICTIONBOOK - ERRORLESS CHAPTER - FRICTIONEXERCISE - MCQ S
125 Videos
Similar Questions
Knowledge Check
The velocity of kerosene oil in a horizontal pipe is 5m/s. If g=10m/s2 then the velocity head of oil wlill be
A1.25 mB12.5 mC0.125mD125mVelocity of flow of water in a horizontal pipe is 10.0 m/s. Find the velocity head of water (g=10m/s2)
A9.00mB5.00 mC3.00 mDNone of theseA block slides with a velocity of 10 m/s on a rough horizontal surface. It comes to rest after covering a distance of 50 metre. If g is 10m/s2, then the coefficient of dynamic friction between the block and the surface is
A0.1B1C10D5
A projectile is thrown with a velocity of 20 m/s, at an angle of 60∘ with the horizontal. After how much time the velocity vector will make an angle of 45∘ with the horizontal (in upward direction) is (take g=10m/s2)−
View SolutionThe velocity of a particle at an instant is 10 m/s. After 5 s, the velocity of the particle is 20 m/s. The velocity at 3 s before the instant when the velocity of the particle was 10 m/s is
View SolutionA missile is fired for maximum range with an initial velocity of 20m/s. If g=10m/s2, the range of the missile is
View SolutionA particle is projected horizontally with a speed V=5m/s from the top of a plane inclined at an angle θ=37∘ to the horizontal (g=10m/s2)
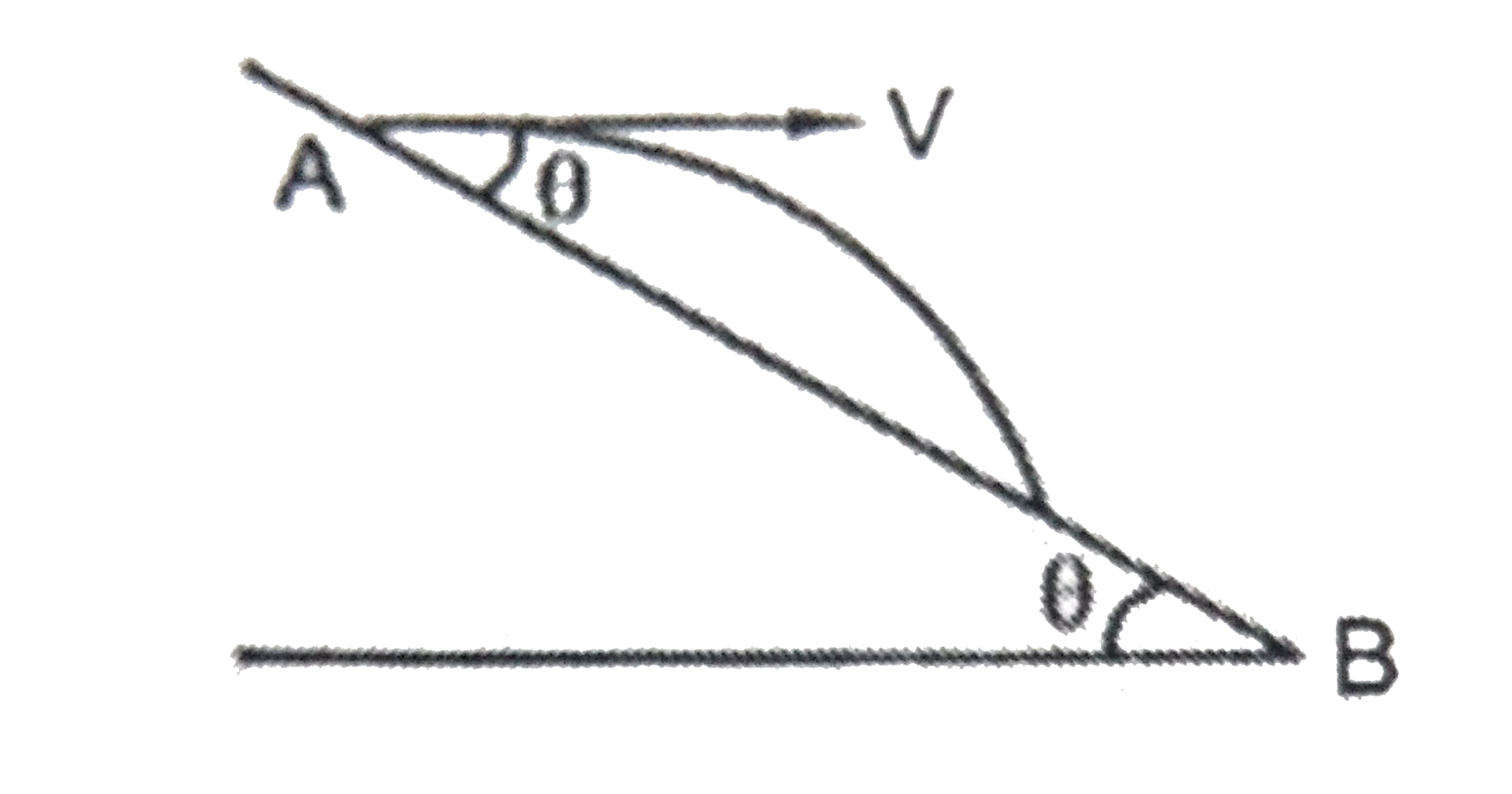
What is the velocity of the particle just before it hits the plane?
View SolutionThe velocity of a particle at an instant is 10m/s. After 5sec, the velocity of the particle is 20m/s. Find the velocity at 3 seconds before from the instant when velocity of a particle is 10m/s.
View Solution