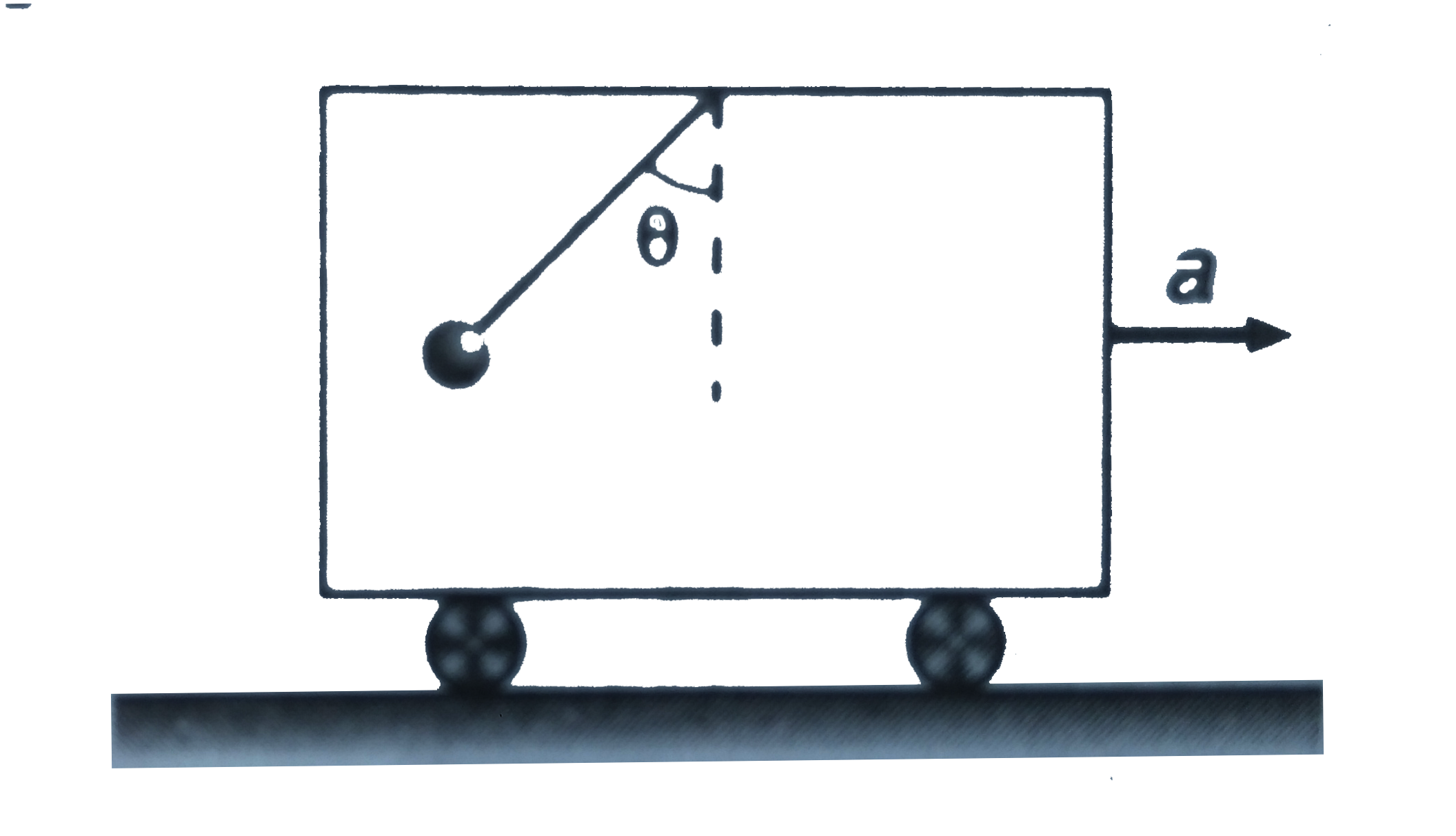
A plumb line is suspended from a ceiling of a car moving with horizontal acceleration of a . What will be the angle of inclination with vertical
The correct Answer is:A
To find the angle of inclination of a plumb line suspended from a ceiling of a car moving with a horizontal acceleration A, we can analyze the forces acting on the plumb line.
1. Identify the Forces:
- The weight of the plumb line acts vertically downward with a force W=mg, where m is the mass of the plumb line and g is the acceleration due to gravity.
- The tension T in the string acts along the string, directed towards the point of suspension.
2. Establish the Coordinate System:
- Let the angle of inclination of the plumb line with the vertical be θ.
- The horizontal acceleration A of the car will create an inertial force acting on the plumb line in the opposite direction (to the left if the car accelerates to the right).
3. Draw a Free Body Diagram:
- The vertical component of the tension T must balance the weight:
Tcosθ=mg
- The horizontal component of the tension must balance the inertial force due to the car's acceleration:
Tsinθ=ma
4. Divide the Equations:
- Dividing the second equation by the first gives:
TsinθTcosθ=mamg
- This simplifies to:
tanθ=ag
5. Find the Angle:
- To find the angle θ, take the arctangent of both sides:
θ=tan−1(ag)
Final Answer:
The angle of inclination of the plumb line with the vertical is given by:
θ=tan−1(ag)
- The weight of the plumb line acts vertically downward with a force W=mg, where m is the mass of the plumb line and g is the acceleration due to gravity.
- The tension T in the string acts along the string, directed towards the point of suspension.
- Let the angle of inclination of the plumb line with the vertical be θ.
- The horizontal acceleration A of the car will create an inertial force acting on the plumb line in the opposite direction (to the left if the car accelerates to the right).
- The vertical component of the tension T must balance the weight:
Tcosθ=mg
- The horizontal component of the tension must balance the inertial force due to the car's acceleration:
Tsinθ=ma
- Dividing the second equation by the first gives:
TsinθTcosθ=mamg
- This simplifies to:
tanθ=ag
- To find the angle θ, take the arctangent of both sides:
θ=tan−1(ag)
The angle of inclination of the plumb line with the vertical is given by:
θ=tan−1(ag)
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBOOK - ERRORLESS CHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Third Law of Motion
24 Videos
- NEWTONS LAWS OF MOTIONBOOK - ERRORLESS CHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Conservation of Linear Momentum and Impulse
27 Videos
- NEWTONS LAWS OF MOTIONBOOK - ERRORLESS CHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Self Evaluation Test
16 Videos
- MOTION IN TWO DIMENSIONBOOK - ERRORLESS CHAPTER - MOTION IN TWO DIMENSIONEXERCISE - Exercise
319 Videos
- ROTATIONAL MOTIONBOOK - ERRORLESS CHAPTER - ROTATIONAL MOTIONEXERCISE - Practice Problems (Problems based on motion of connected mass)
10 Videos
Similar Questions
A plumb line is suspended from a celling of a car moving with horizontal acceleration of a. What will be the angle of inclination with vertical?
View SolutionA bob of mass m is suspended from the ceiling of a train moving with an acceleration a as shown in figure. Find the angle θ in equilibrium position.
View Solution
Knowledge Check
A simple pendulum is suspended from the ceiling of a train which is moving with an acceleration a .The angular of inclination of the pendulum from the vertical will be
A90∘B0∘Ctan−1(ag)Dtan−1(ga)A simple pendulum with a bob of mass m is suspended from the roof of a car moving with a horizontal accelertion a. The angle made by the string with verical is
Atan−1(ag)Btan−1(1−ag)Ccos−1(ag)Dsin−1(ag)A simple pendulum is suspended from the roof of a train. If the train is moving with an acceleration 49cm/s2. Then the angle of inclination of the string about the vertical will be
A20∘BzeroC30∘D3∘
A small metallic sphere of mass m is suspended from the ceiling of a car accelerating on a horizontal road with constant acceleration a. The tension in the string attached with metallic sphere is
View SolutionA pendulum is hanging from the ceiling of a car having an acceleration a0 with respect to the road. Findthe angle made by the string with the vertical.
View SolutionA simple pendulum with a bob of mass m is suspended from the roof of a car moving with horizontal acceleration a
View SolutionA seconds pendulum is suspended from the ceiling of a trolley moving horizontally with an acceleration of 4m/s2 . Its period of oscillation is
View SolutionA simple pendulum with a bob of mass m is suspended from the roof of a car moving with a horizontal acceleration a.
View Solution
