A mass is hanging on a spring balance which is kept in a lift. The lift ascends. The spring balance will show in its reading
Increase
Decrease
No change
Change depending upon velocity
The correct Answer is:D
To solve the problem of how a spring balance reading changes when a mass is hanging in a lift that is ascending, we can follow these steps:
1. Identify the Forces Acting on the Mass:
- When the mass is hanging in the lift, two main forces are acting on it:
- The gravitational force (weight) acting downwards, which is given by mg (where m is the mass and g is the acceleration due to gravity).
- The spring force acting upwards, which is the force exerted by the spring balance, denoted as Fs.
2. Consider the Lift's Acceleration:
- Since the lift is ascending, it will have an upward acceleration, denoted as a.
- According to Newton's second law, the net force acting on the mass can be expressed as:
Fnet=Fup−Fdown=Fs−mg
3. Apply Newton's Second Law:
- The net force is also equal to the mass times its acceleration:
Fnet=ma
- Therefore, we can set the two expressions for net force equal to each other:
Fs−mg=ma
4. Rearranging the Equation:
- Rearranging the equation gives us the spring force:
Fs=mg+ma
5. Factor Out the Mass:
- We can factor out the mass m from the right side:
Fs=m(g+a)
6. Interpret the Spring Balance Reading:
- The reading on the spring balance Fs represents the apparent weight of the mass when the lift is accelerating upwards. Thus, the reading increases compared to the normal weight mg when the lift is stationary or moving at constant velocity.
Conclusion:
- The spring balance will show a reading of m(g+a) when the lift is ascending, indicating that the reading increases due to the upward acceleration of the lift.
- When the mass is hanging in the lift, two main forces are acting on it:
- The gravitational force (weight) acting downwards, which is given by mg (where m is the mass and g is the acceleration due to gravity).
- The spring force acting upwards, which is the force exerted by the spring balance, denoted as Fs.
- Since the lift is ascending, it will have an upward acceleration, denoted as a.
- According to Newton's second law, the net force acting on the mass can be expressed as:
Fnet=Fup−Fdown=Fs−mg
- The net force is also equal to the mass times its acceleration:
Fnet=ma
- Therefore, we can set the two expressions for net force equal to each other:
Fs−mg=ma
- Rearranging the equation gives us the spring force:
Fs=mg+ma
- We can factor out the mass m from the right side:
Fs=m(g+a)
- The reading on the spring balance Fs represents the apparent weight of the mass when the lift is accelerating upwards. Thus, the reading increases compared to the normal weight mg when the lift is stationary or moving at constant velocity.
- The spring balance will show a reading of m(g+a) when the lift is ascending, indicating that the reading increases due to the upward acceleration of the lift.
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBook:ERRORLESS Chapter:NEWTONS LAWS OF MOTIONExercise:Third Law of Motion
Explore 24 Videos
- NEWTONS LAWS OF MOTIONBook:ERRORLESS Chapter:NEWTONS LAWS OF MOTIONExercise:Conservation of Linear Momentum and Impulse
Explore 27 Videos
- NEWTONS LAWS OF MOTIONBook:ERRORLESS Chapter:NEWTONS LAWS OF MOTIONExercise:Self Evaluation Test
Explore 16 Videos
- MOTION IN TWO DIMENSIONBook:ERRORLESS Chapter:MOTION IN TWO DIMENSIONExercise:Exercise
Explore 319 Videos
- ROTATIONAL MOTIONBook:ERRORLESS Chapter:ROTATIONAL MOTIONExercise:Practice Problems (Problems based on motion of connected mass)
Explore 10 Videos
Similar Questions
Knowledge Check
The readings of the spring balance will be :
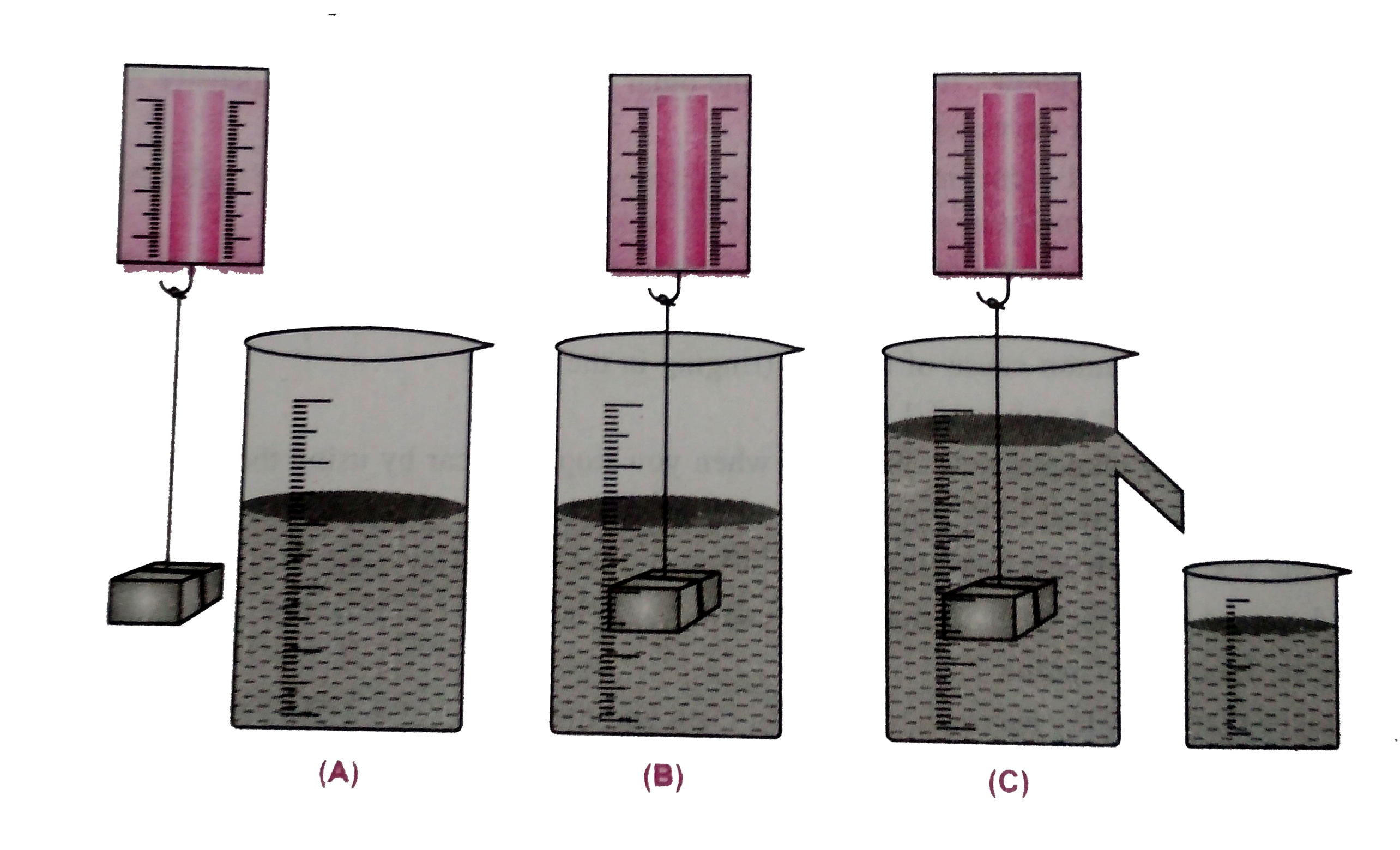 Aequal to each other in all cases A,BandC.Bequal to each other in cases AandC only.Cequal to each other in cases BandC only.Ddifferent in every case.
Aequal to each other in all cases A,BandC.Bequal to each other in cases AandC only.Cequal to each other in cases BandC only.Ddifferent in every case.A man of mass 60 kg is standing on a spring balance inside a lift. If the lift falls downwards, then the reading of the spring balance will be:-
AzeroB60 kgfC<60kgfD>60kgfA body of mass 2 kg is hung on a spring balance mounted vertically in a lift. If the lift descends with an acceleration equal to the acceleration due to gravity ‘ g ’, the reading on the spring balance will be
A2 kgB(4×g)kgC(2×g)kgDZero
A body of mass 15 kg is hung by a spring balance in a lift. What would be the reading of the balance when (i) the lift is ascending with an acceleration of 2ms−2 (ii) descending with the same acceleration (iii) descending with a constant velocity of 2ms−1.? Take g = 10 ms−2.
View SolutionA stone is tied to a spring balance. Under which of the following conditions the reading on spring balance will show the least weight?
View SolutionA boy whose mass is 50 kg stands on a spring balance inside a lift. The lift starts to ascent with an acceleration of 2ms−2. The reading of the machine or balance (g=10ms−2) is
View SolutionAn object is suspended from a spring balance in a lift. The reading is 240 N when the lift is at rest. If the spring balance reading now change to 220N, then the lift is moving
View SolutionA bird is sitting in a wire cage which is hanging from a spring balance ,if the bird starts flying inside the cage , the reading of the spring balance will :
View Solution