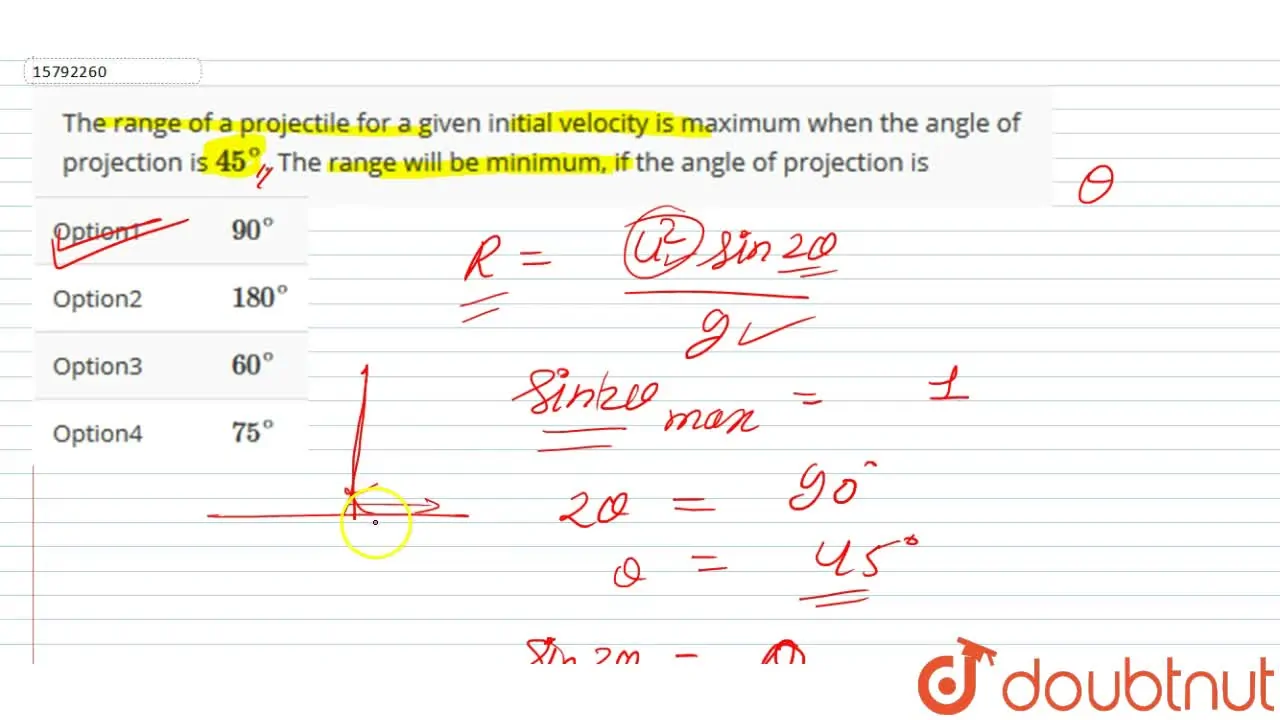
The range of a projectile for a given initial velocity is maximum when the angle of projection is $45^{\circ}$ . The range will be minimum, if the angle of projection is

A

$90^{\circ}$

B

$180^{\circ}$

C

$60^{\circ}$

D

$75^{\circ}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

|

Answer

Step by step video, text & image solution for The range of a projectile for a given initial velocity is maximum when the angle of projection is 45^(@). The range will be minimum, if the angle of projection is by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.

Updated on:21/07/2023

Class 11 PHYSICS PROJECTILE MOTION

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The horizontal range of a projectile is $4 \sqrt{3}$ times its maximum height. The angle of projection is
A $30^{\circ}$
B $45^{\circ}$
C $60^{\circ}$
DNone
Question 2 - Select One
The horizontal range of a projectile is times of its maximum height. Its angle of projection will be :-
A $45^{\circ}$
B $60^{\circ}$
C $90^{\circ}$
D $30^{\circ}$
Question 3 - Select One
The horizontal range of a projectile is $2 \sqrt{3}$ times its maximum height. Find the angle of projection.
A ${tan}^{- 1} (\frac{\sqrt{3}}{\sqrt{2}})$
B ${sin}^{- 1} (\frac{2}{\sqrt{3}})$
C ${cot}^{- 1} (\frac{2}{\sqrt{3}})$
D ${tan}^{- 1} (\frac{2}{\sqrt{3}})$

ERRORLESS -MOTION IN TWO DIMENSION-Exercise

Home
Ask Doubt
Profile