Question

A heating coil of resistance $5\Omega$ is connected to a cell . The internal resistance of the cell is $20\Omega$ Calculate the value of the shunt to be introduced , so that , the energy consumed in the heating coil will be $\frac{1}{19}$ th of the previous value .

Explanation

Let E be the emf of the cell and S be the shunt . [ Fig . 3.2]
Here ,internal resistance of the cell , `r=20Omega` and exteranal resistance , `R=5Omega` .

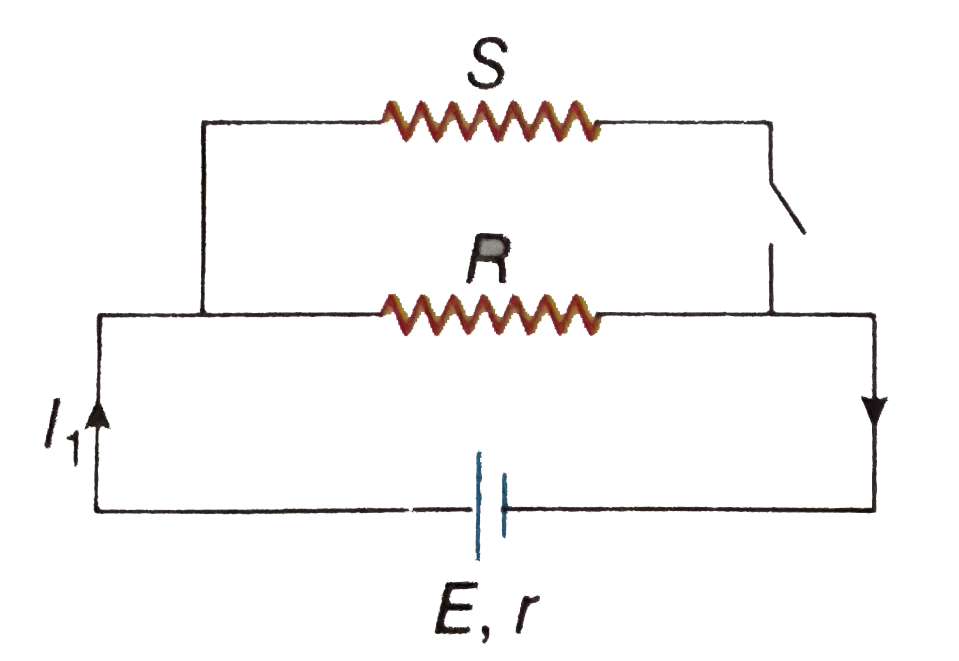
In absence of the shut , current flowing in the circuit ,
`I_(1)=(E)/(R+r)`
`therefore` In tiem t , energy consumed in the resistaance R ,
`W_(1)=I_(1)^(2)Rt=(E^(2)Rt)/(R+r)^(2)`
Now , if shunt S is connected ,current flowing in the circuit, `I_(1)=(E)/((RS)/(R+S)+r)=(E(R+S))/(RS+r(R+S))`
`therefore` current flowing ion the resistance R ,
`I_(R)=(S)/(R+S)=(ES)/(RS+r(R+S))`
`therefore` In time t , energy consumed in the resistance R,
According to the question ,
`W_(2)=(W_(1))/(9)" or,"W_(1)=9W_(2)`
`therefore (E^(2)Rt)/((R+r)^(2))=9.(E^(2)S^(2)Rt)/([RS+r(R+S)]^(2))`
`"or, "(1)/((R+r)^(2))=(9S^(2))/([RS+r(R+S)]^(2))`
`"or ,"(1)/(R+r)=(3S)/(RS+r(R+s))"or,"R+r=(RS+rR+rS)/(3S)`
`"or, " S=(rR)/(2(R+r))=(20xx5)/(2(20+5))=2Omega`