Question

A particle charged with $1.6\times {10}^{-19}C$ is in motion. It enters the space between two parallel metal plates, parallely along the midway between them. The plates are 10 cm long and the separation between them is 2 cm. A potential difference of 300V exists between the plates. Find out the maximum velocity of the charged particle at the point of entry, for which it would be unable to emerge from the space between the plates. Given, mass of the particle = $12\times {10}^{-24}kg$.

Explanation

Length of the plates A and B is
`l = 10 cm = 0.1m`
Half of the distance between the plates,
`d = 1/2 xx 2 cm = 1 cm = 0.01 cm`

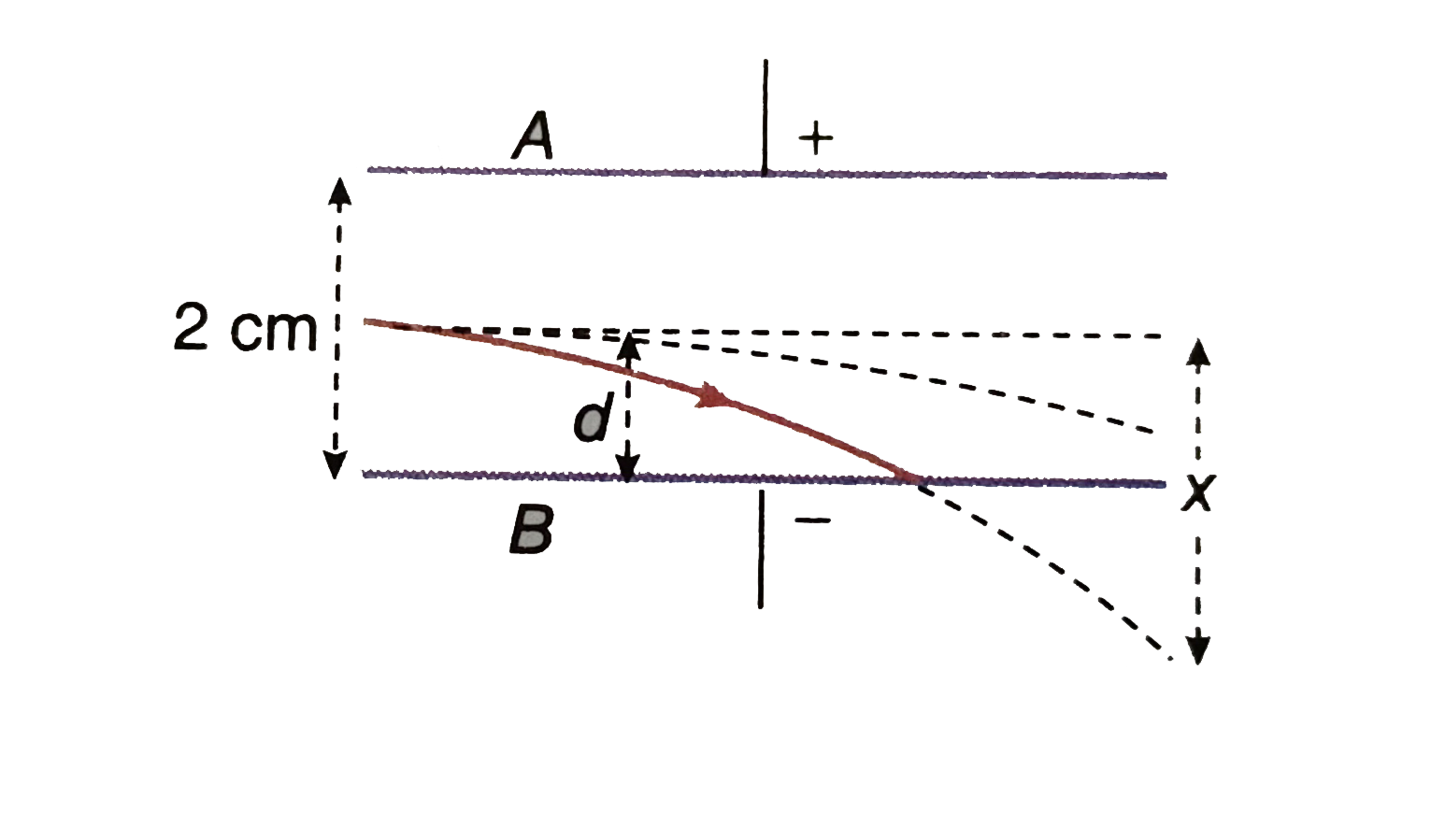
Uniform electric field in the intermediate space,
`E = (300V)/(0.02 m) = 15 xx 10^(3) V cdot m^(-1)`
Let the charge `q = 1.6 xx 10^(-19)` be positive.
So, a downward force acts on it due to the electric field E, given by
`F = qE`
`:.` Downward acceleration, `a = (qE)/(m)`
At the point of entry, let v be the velocity of the charged particle.
In the axial direction, it experiences no force, so the time taken to travel the distance l is `t = 1/v`
Again, at the point of entry, the downward component of velocity = 0, Thus the downward displacement in time t is
`x = 1/2 at^(2) = 1/2 (qE)/(m)(l/v)^(2)`
The condition, that the particle will not emerge from the space between the plates, is
`x ge d`
or `1/2 (qE)/(m)(l/v)^(2) ge d or, 1/2 (qEl^(2))/(md) ge v^(2) or, v le 1sqrt((qE)/(2md))`
Therefore, the maximum permitted velocity is
`v_(max) lsqrt((qE)/(2md)) = 0.1 xx sqrt(((1.6xx10^(-19))xx(15xx10^3))/(2xx(12xx1^(-24))xx0.01))`
`= 10^(4) m cdot s^(-1)`.