Two exactly similar wire of steel and copper are stretched by equal force. If the difference in their elongations is 0.5 cm, the elongation of each wire is
The correct Answer is:A
To solve the problem, we need to find the elongation of each wire (steel and copper) given the difference in their elongations and their respective Young's moduli.
Step 1: Understand the relationship between Young's modulus, stress, and strain
Young's modulus (Y) is defined as the ratio of stress to strain. For two wires of the same length (L) and cross-sectional area (A), the elongation (ΔL) can be expressed as:
Y=F/AΔL/L⟹Y=F⋅LA⋅ΔL
Where:
- Y = Young's modulus
- F = Force applied
- A = Cross-sectional area
- ΔL = Elongation
- L = Original length
Step 2: Set up the equations for both wires
Let ΔLS be the elongation of the steel wire and ΔLC be the elongation of the copper wire.
From the definition of Young's modulus, we can write:
YS=F⋅LA⋅ΔLS(for steel)
YC=F⋅LA⋅ΔLC(for copper)
Step 3: Relate the elongations using Young's moduli
Since the wires are similar (same dimensions), we can relate their elongations:
YSYC=ΔLCΔLS
Substituting the values of Young's moduli:
2.0×10111.2×1011=ΔLCΔLS
Calculating the ratio:
2.01.2=ΔLCΔLS⟹ΔLC=2.01.2ΔLS≈1.67ΔLS
Step 4: Use the difference in elongations
We know that the difference in elongations is given as:
ΔLC−ΔLS=0.5 cm
Substituting ΔLC in terms of ΔLS:
1.67ΔLS−ΔLS=0.5
This simplifies to:
0.67ΔLS=0.5
Step 5: Solve for ΔLS
Now, we can solve for ΔLS:
ΔLS=0.50.67≈0.746 cm≈0.75 cm
Step 6: Find ΔLC
Now, substitute ΔLS back to find ΔLC:
ΔLC=1.67ΔLS=1.67×0.75≈1.25 cm
Final Results
- Elongation of steel wire ΔLS≈0.75 cm
- Elongation of copper wire ΔLC≈1.25 cm
Young's modulus (Y) is defined as the ratio of stress to strain. For two wires of the same length (L) and cross-sectional area (A), the elongation (ΔL) can be expressed as:
- Y = Young's modulus
- F = Force applied
- A = Cross-sectional area
- ΔL = Elongation
- L = Original length
Let ΔLS be the elongation of the steel wire and ΔLC be the elongation of the copper wire.
YC=F⋅LA⋅ΔLC(for copper)
Since the wires are similar (same dimensions), we can relate their elongations:
We know that the difference in elongations is given as:
Now, we can solve for ΔLS:
Now, substitute ΔLS back to find ΔLC:
- Elongation of steel wire ΔLS≈0.75 cm
- Elongation of copper wire ΔLC≈1.25 cm
Topper's Solved these Questions
Similar Questions
Two exactly similar wires of steel and copper are stretched by equal forces. If the total elogation is 1cm. Find by how much is each wire elongated ? Given Y for steel =20×1011dyne/cm2andYforcopper=12×1011dyne/cm2
View SolutionA weight of 100N is suspended by two wires made by steel and copper as shown in figure length of steel wire is 1m and copper wire is √3m. Find ratio of change in length of copper wire (Δlc) to change in length of steel wire (Δls). given Young's modulus YSteel=2×1011N/m2,YCopper=1×1011N/m2
_PHY_E01_017_Q01.png)
View Solution
Knowledge Check
Two exactly similar wires of steel and copper are stretched by equal force. If the total elongation is 2 cm, then how much is the elongation in steel and copper wire respectively? Given, Ysteel=20×1011dyne/cm2,Ycopper=12×1011Dyne/cm2
A1.25cm,0.75cmB0.75cm,1.25cmC1.15cm,0.85cmD0.5cm,1.15cmTwo exactly similar wires of steel (ys=20×1011dyne/cm2) and copper (yc=12×1011dyne/cm2) are stretched by equal forces. If the total elongation is 1 cm, elongation of copper wire is
A3/5 cmB5/3 cmC3/8 cmD5/8 cmA copper wire of length 2.2m and a steel wire of length 1.6m, both of diameter 3.0mm are connected end to end. When stretched by a force, the elongation in length 0.50 mm is produced in the copper wire. The streching force is (Ycopper=1.1×1011Nm−2,
Ysteel=2.0×1011Nm−2)A1.8×102NB2.4×102NC3.6×102ND5.4×102N
Two wires one of copper and other of iron having same cross-section area and lengths 2 m and 1.5 m respectively are fastened end and stretched by a load 10 N. If copper wire is stretched by 2mm then find the total extension of combined wire, YCu=1×1011N/m2 and YFe=3×1011N/m2.
View SolutionTwo wires, one made of capper and other of steel are joined end to end. (as show in figure). The area of cross-section of copper wire is twice that of steel wire, They ae placed under compressive force of magnitude F. Find the ratio of their lenghts such that change in lenght of both wire are same (YS=2×1011N/m2andYC=1.1×1011N/m2)
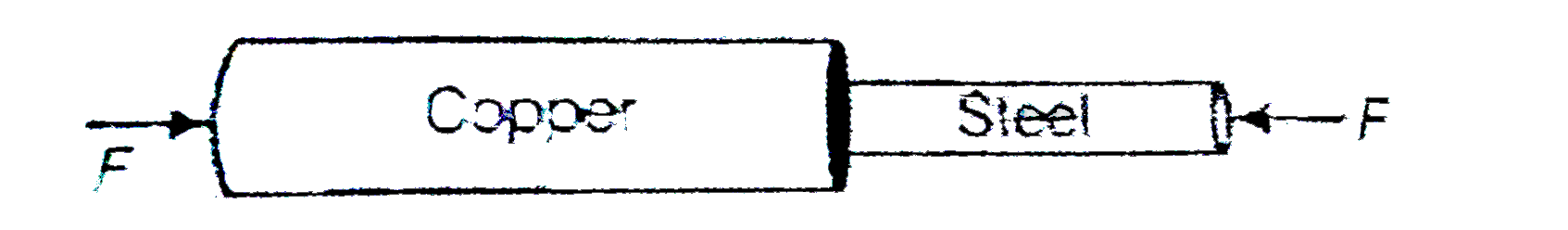
View SolutionTwo wires one of copper and other of steel having same cross-sectionl area end to end and strecteched by a load M. Ifcopper wire Is stretched by 1 mm, the total extnsion of the combined wire is
(Givwen, Young's are Ycopper=1×1011N/m2,andYsteel=2×1011N/m2)
View SolutionA copper wire of length 2.4 m and a steel wire of length 1.6 m, both of diameter 3 mm, are connected end to end. When stretched by a load, the net elongation found to be 0.7 mm. The load appled is
(YCopper=1.2×1011Nm−2,Ysteel=2×1011Nm−2)
View SolutionA brass of length 2m and cross-sectional area 2.0cm2 is attached end to end to a steel rod of length and cross-sectional area 1.0cm2. The compound rod is subjected to equal and oppsite pulls of magnitude 5×104N at its ends. If the elongations of the two rods are equal, the length of the steel rod (L) is
{YBrass=1.0×1011N/m2 and Ysteel=2.0×1011N/m2
View Solution
