Two wires A and B of same length and of the same material have the respective radii $r_{1}$ and $r_{2}$ . Their one end is fixed with a rigid support, and at other end equal twisting couple is applied. Then the ratio of the angle of twist at the end of A and the angle of twist at the end of B will be

$\frac{r_{1}^{2}}{r_{2^{2}}}$

$\frac{r_{2}^{2}}{r_{2}^{2}}$

$\frac{r_{2}^{4}}{r_{1}^{4}}$

$\frac{r_{1}^{4}}{r_{2}^{4}}$

Video Solution

Text Solution

Generated By DoubtnutGPT

The correct Answer is:C

Updated on:7/8/2024

Two rods A and B of the same material and length have radii $r_{1}$ and $r_{2}$ respective. When they are rigidly fixed at one end and twisted by the same torque applied at the other end, the ratio $[\frac{the angle of twist at the end of A}{the angle of twist at the end of B}]$ equal to
A $\frac{r_{1}^{2}}{r_{2}^{2}}$
B $\frac{r_{1}^{3}}{r_{2}^{3}}$
C $\frac{r_{2}^{4}}{r_{1}^{4}}$
D $\frac{r_{1}^{4}}{r_{2}^{4}}$
Two wires of the same length and material but different radii $r_{1}$ and $r_{2}$ are suspended from a rigid support both carry the same load at the lower end. The ratio of the stress devedoped in the second wire to that developed in the first wire is
A $\frac{r_{1}}{r_{2}}$
B $\frac{r_{1}^{2}}{r_{2}^{2}}$
C ${(\frac{r_{1}}{r_{2}})}^{5 / 2}$
D ${(\frac{r_{1}}{r_{2}})}^{1 / 2}$
The wires $A$ and $B$ shown in Fig. are made of the same material and have radii $r_{A}$ and $r_{B}$ , respectively. The block between them has a mass $m$ . When the force $F$ is $m g / 3$ , one of the wires breaks. Then

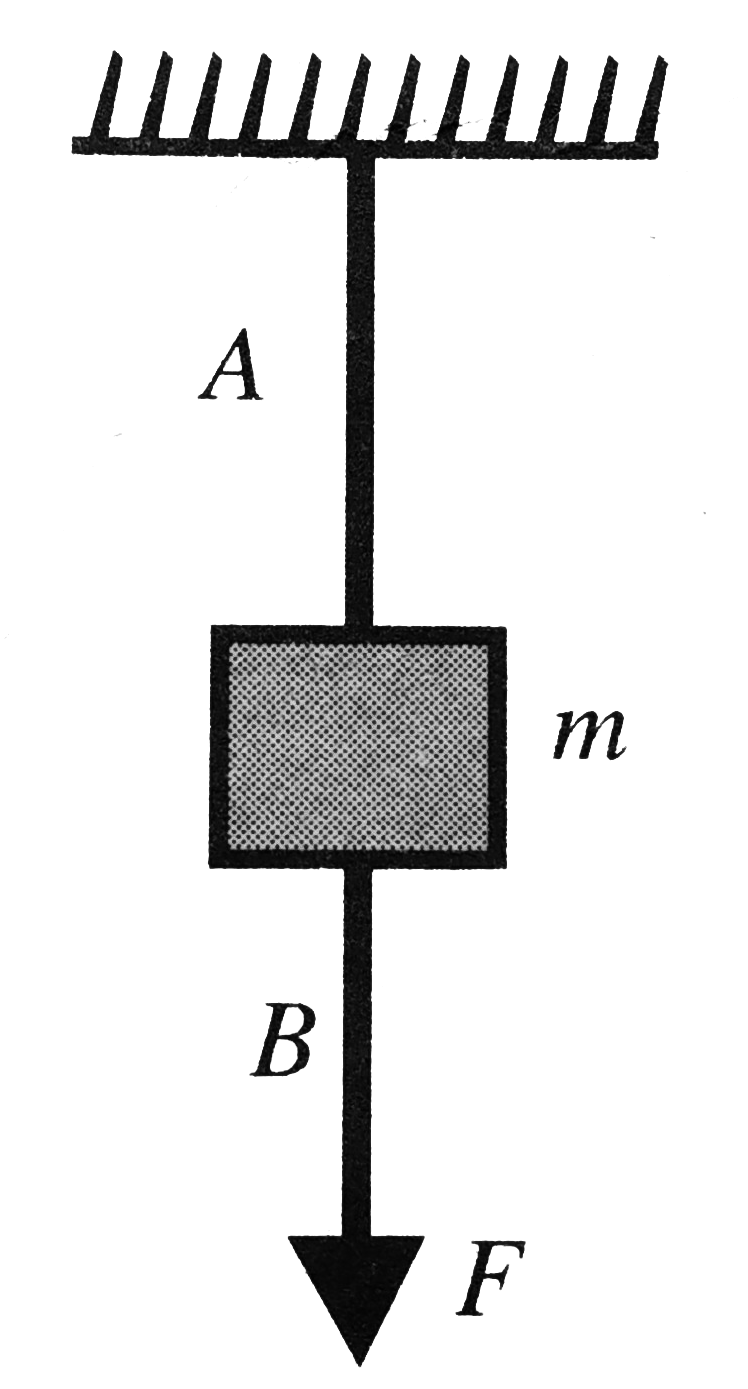A $A$ will break before $B$ if $r_{A} = r_{B}$
B $A$ will break before $B$ if $r_{A} < 2 r_{B}$
Ceither $A$ or $B$ may break if $r_{A} = 2 r_{B}$
Dthe lengths of $A$ and $B$ must be known to predict which wire will break.