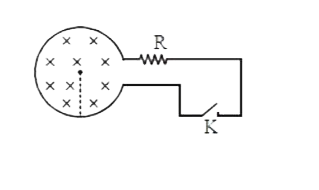
Shown in the figure is a circular loop of radius r and resistance R. A variable magnetic field of induction B=B0e−t is established inside the coil. If the key (K) is closed, the electric power developed right after closing the switch is equal to:-
B20πr2R
B010r3R
B20π2r4R5
B20π2r4R
The correct Answer is:D
Induced emf,
E=dϕdt
=ddt(BA)=AdBdt
=πr2B0ddt(e−t)
=−πr2B0e−t
At t=0, E0=B0πr2
The electric power developed in the resistor R just at the instant of closing the key is:
P=E20R=B20π2r4R
Topper's Solved these Questions
Similar Questions
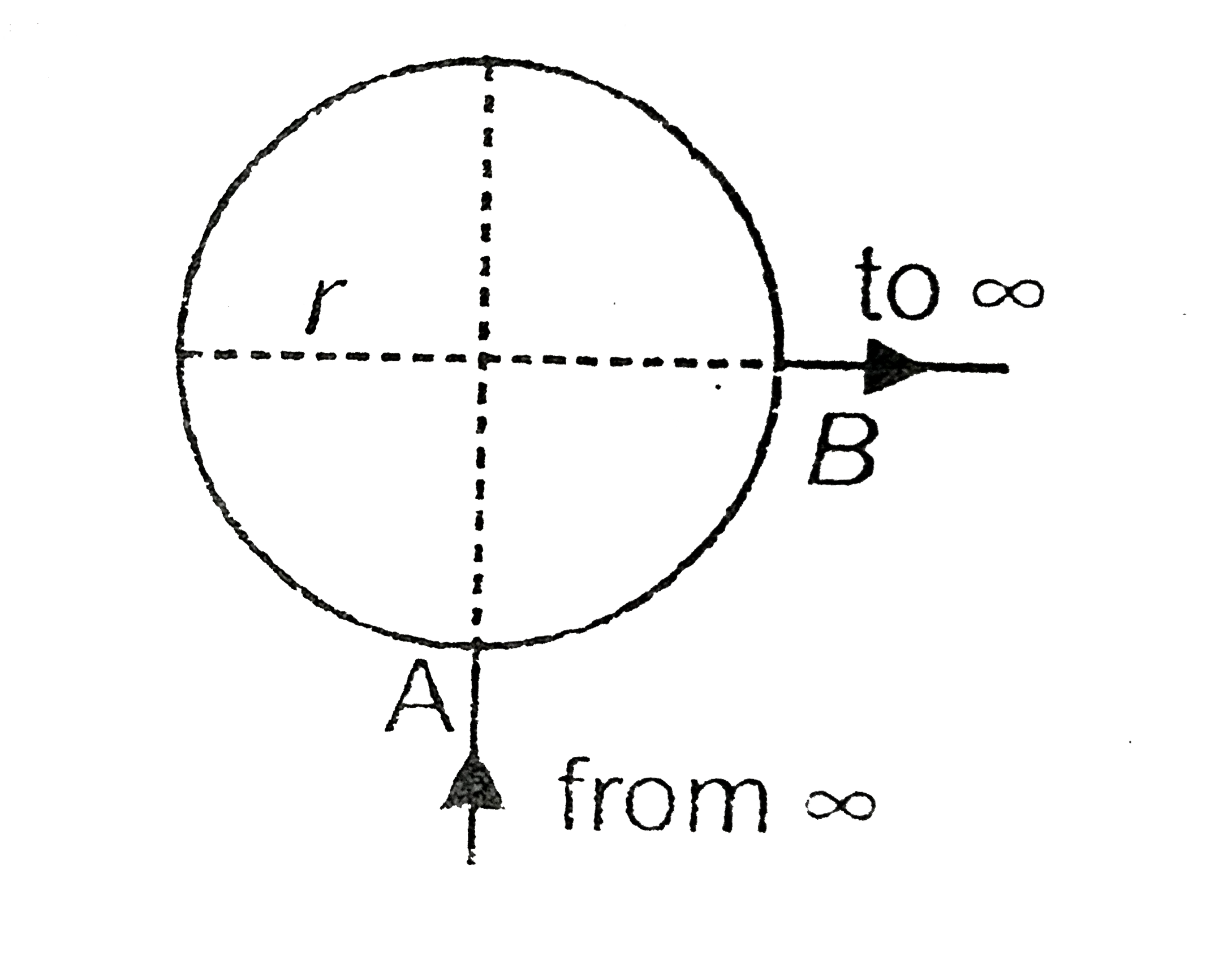
In the figure, the current l enters the circular loop of uniform wire of radius r at A and leaves it at B. The magnetic field of the centre of circular loop is
View SolutionA circular brass loop of radius a and resistance R is placed with it plane perpendicular to a magnetic field, which varies with time as B=B0sinωt. Obtain the expression for the induced current in the loop.
View Solution
Knowledge Check
Shown in the figure is a circular loop of radius r and resistance R. A varible magnetic field of induction B=B0e−1 is established inside the coil. If the key (K) is closed, the electrical power devloped right after closing the switch is equal to
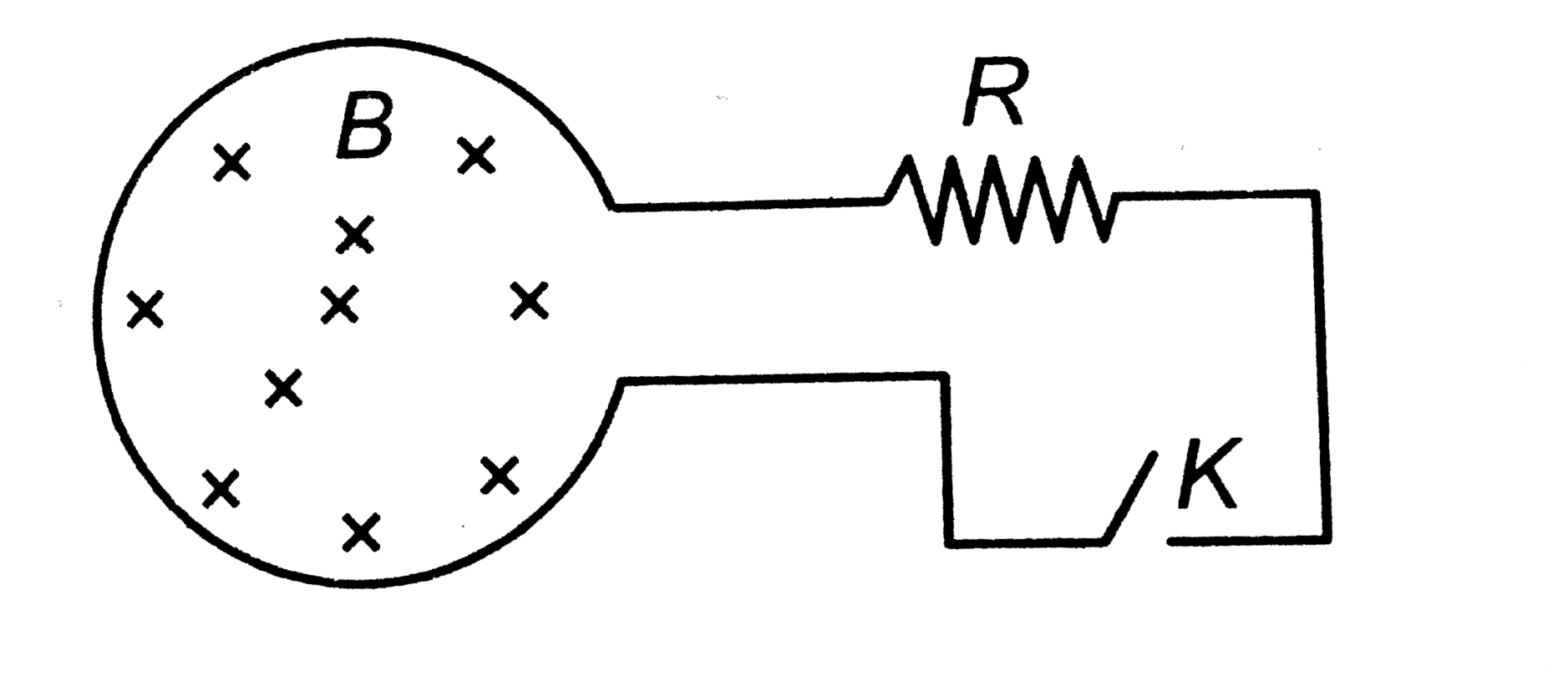 AB20πr2RBB010r3RCB20π2r4R5DB20π2r4R
AB20πr2RBB010r3RCB20π2r4R5DB20π2r4RShown in the figure is an R−L circuit. Just after the key (K) is closed
 Athe current in the circuit is zeroBpotential drop across the resistor is zeroCemf developed across the inductor equals the emf of the batteryDno heat is dissipated in the circuit
Athe current in the circuit is zeroBpotential drop across the resistor is zeroCemf developed across the inductor equals the emf of the batteryDno heat is dissipated in the circuitAs shown in the figure, the key K is closed, the direction of the induced current in the coil B will be -
 Aclockwise and instantaneousBanti-clockwise and instantaneousCclockwise and continuousDanti-clockwise and continuous
Aclockwise and instantaneousBanti-clockwise and instantaneousCclockwise and continuousDanti-clockwise and continuous
A conducting circular loop of raidus and resistance R is kept on a horiozntel plane. A vertical time varing magnetic field B=2t is switched on at time t=0. Then
View SolutionA circular flexible loop of wire of radius r carrying a current I is placed in a uniform magnetic field B . If B is doubled, then tension in the loop
View SolutionA circular loop of radius R is placed in a uniform magnetic field B=2sinp Tesla as shown. Which of the following is incorrect?
View SolutionA uniform circular loop of radius a and resistance R is pulled at a constant velocity v out of a region of a uniform plane of the loop and the velocity are both perpendicular to B. Then the electrical power in the circular loop at the instant when the arc (of the circular loop) outside the region of magnetic field subtends an angle π3 at the centre of the loop is
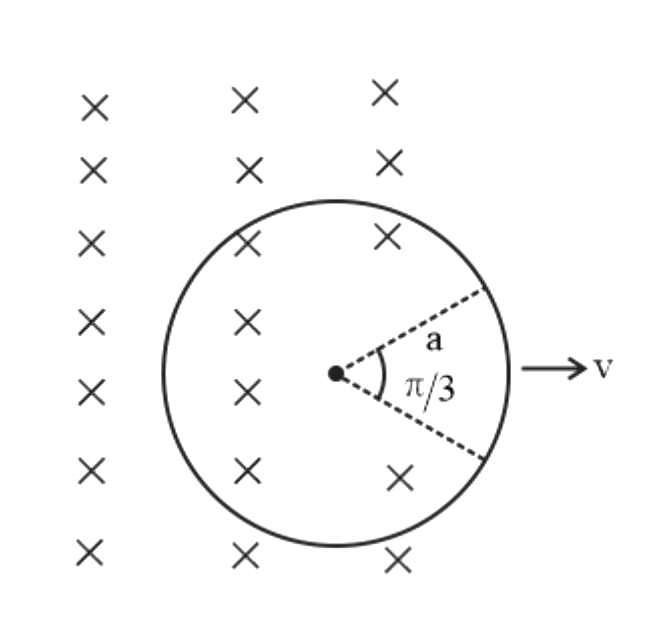
View SolutionA uniform circular loop of radius a and resistance R palced perpendicular to a uniform magnetic field B. One half of the loop is rotated about the diameter with angular velocity ω as shown in Fig. Then, the current in the loop is

View Solution