A source emitting sound of is fixed on block which is attache to free end of a spring as shown in figure. The detector fixed on block attached to free end of spring detects this sound. The blocks and simultaneously displaced towards each other thrugh a distance of and then left to vibrate. If the product of maximum and minimum freuquencies of sound detected by is . Find . Given the vibrational frequencies of each block is . speed of sound in air
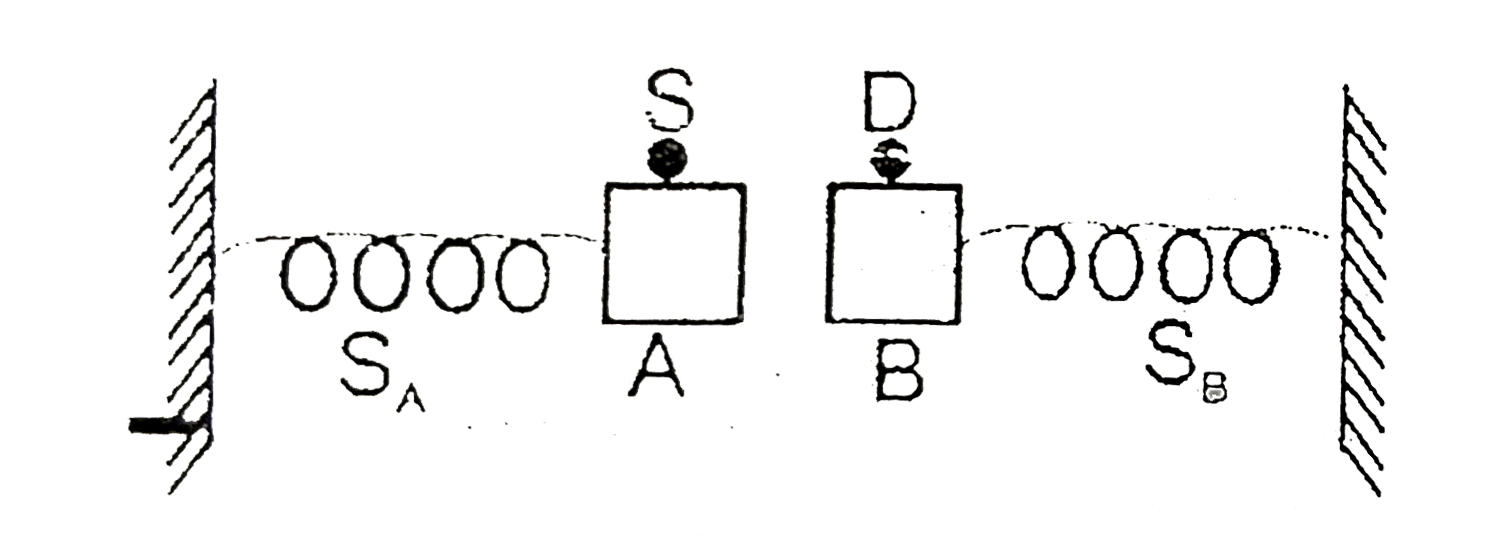
The correct Answer is:9
Step by step video, text & image solution for A source S emitting sound of 300 Hz is fixed on block A which is attache to free end of a spring S_(A) as shown in figure. The detector D fixed on block B attached to free end of spring S_(B) detects this sound. The blocks A and B simultaneously displaced towards each other thrugh a distance of 2.0m and then left to vibrate. If the product of maximum and minimum freuquencies of sound detected by D is K + 10^(4) sec^(-2). Find K. Given the vibrational frequencies of each block is 5//pi Hz. speed of sound in air = 300 m//s by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- SOUND WAVESBOOK - RESONANCECHAPTER - SOUND WAVESEXERCISE - Exercise- 2 PART - III
21 Videos
- SOUND WAVESBOOK - RESONANCECHAPTER - SOUND WAVESEXERCISE - Exercise- 2 PART - IV
8 Videos
- SOUND WAVESBOOK - RESONANCECHAPTER - SOUND WAVESEXERCISE - Exercise- 2 PART - I
25 Videos
- SIMPLE HARMONIC MOTIONBOOK - RESONANCECHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercise
28 Videos
- STRING WAVESBOOK - RESONANCECHAPTER - STRING WAVESEXERCISE - Exercise
32 Videos
Similar Questions
In a wave motion y=asin(kx−ωt), y can represent
View SolutionIn an organ pipe (may be closed or open ) of 99cm length standing wave is set up , whose equation is given by longitudinal displacement.

ξ=(0.1mm)cos(2π)0.8(y+1cm)cos(400)twhere y is measured from the top of the tube in metres and tinseconds . Here 1cm is the end correction.
The upper end and the lower end of the tube are respectively .
View SolutionWhen we clap our hands, the sound produced is best described by Here p denotes the change in pressure from the equilibrium value
View SolutionEquation of two S.H.M. x1=5sin(2πt+π/4),x2=5√2(sin2πt+cos2πt) ratio of amplitude & phase difference will be
View Solutiontwo particle of medium disturbed by the wave propagation are at x1=0andx2=1cm. The respective displacement (in cm) of the particles can be given by the equation:
y1=2sin3πt,y2sin(3πt−π/8) the wave velocity is
View SolutionA block (B) is attached to two unstriched sprig S_(1) and S_(2) with spring constant K and 4K , respectively (see fig 1) The other ends are atteched in identical support M_(1) and M_(2) not attached in the walls . The springs and supports have negligible mass . There is no friction anywhere . The block B is displaced toword wall 1 by a small distance z (figure (ii)) and released . THe block return and moves a maximum displacements x and y are musured with reoact to the equalibrum of the block B and the ratio y//x is
View SolutionA block of mass m is attached to one end of a mass less spring of spring constant k. the other end of spring is fixed to a wall the block can move on a horizontal rough surface. The coefficient of friction between the block and the surface is mu then the compession of the spring for which maximum extension of the spring becomes half of maximum compression is .
View SolutionTwo blocks A and B of equal mass m=1.0kg are lying on a smooth horizontal surface as shown in figure. A spring of force constant k=200N//m is fixed at one end of block A. Block B collides with block A with velocity v_0=2.0m//s . Find the maximum compression of the spring.
View SolutionA source S emitting sound of 300 Hz is fixed of block A which is attached to free end of a spring S_A as shown in the figure. The detector D fixed on block B attached to the free end of spring S_B detects this sound. The blocks A and B are simultaneously displaced towards each other through of 1.0 m and then left to vibrate. Find the maximum and minimum frequencies of sound detected by D if the vibrational frequency of each block is 2 Hz (velocity of sound is 340 m/s).
View SolutionOne end of a spring is rigidly fixed. A block attached to the free end of the spring is pulled through a distance x_(0) . On releasing the block, its amplitude of motion cannot exceed +-x_(0) . Why?
View SolutionA source S emitting sound of frequency 288 Hz is fixed on block B which is attached to the free end of a spring S_(2) and an observer O is on block A which is attached to the free end of spring S_(1) as shown in the figure. The blocks A and B are simultaneously displaced towards each other through a distance of 0.5 m and then left to oscillate. If the angular velocity of each blocks is 40"rad"s^(-1) , then the maximum frequency observed by the observer is (speed of sound in air is 340 ms^(-1) )
View SolutionA source S emitting sound of frequency 300Hz is fixed on block A which is attached to the free end of a spring SA as shown in figure. The detector D fixed on block B attached to free end of spring SB detects this sound. The blocks A and B are simultaneously displaced towards each other through a distance of 1.0m and then left to vibrate. The maximum and minimum frequencies of sound detected by D, if the vibrational frequency of each block is 2Hz are (Velocity of sound v=340m/s)
View SolutionA source 'S' emitting a sound of frequency 300 Hz is fixed on block ‘A’ while detector is fixed on block 'B' detects the sound. The block 'A' and 'B' are simultaneously displaced towards each other through a distance of 1 m and then left to vibrate. If the velocity of sound in air is 340ms^(-1) and S_A , S_B are identical the frequency of block A is 2Hz. Then
View SolutionA source .S. emitting sound of 300Hz is fixed on block .A. which is attached to free end on spring SA as shown in the figure. The detector .D. fixed on block .B. attached to the free end of spring SB detects this sound. The blocks .A. and "B. are simulataneously displaced towards eachother through a distance of lm and then left to vibrate. Find the maximum and minimum frequencies of sound detected by .D. if the vibrational frequency of each block is 2Hz (speed of sound 340ms−1)
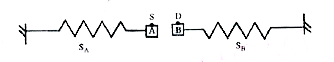
View SolutionA source .S. emitting a sound of frequency 300 Hz is fixed on block .A. while detector is fixed on block .B. detects the sound. The block .A. and .B. are simultaneously displaced towards each other through a distance of 1 m and then left to vibrate. If the velocity of sound in air is 340ms−1 and S , SA,SB are identical the frequency of block A is 2Hz. Then
View Solution
