A square brass plate of side and thickness is subjected to a force on its smaller opposite edges, causing a displacement of . If the shear modulus o brass is , the value on force is
The correct Answer is:C
To find the force F applied to the square brass plate, we can use the relationship between stress, strain, and shear modulus. Here’s a step-by-step solution:
Step 1: Identify the given values
- Side of the square plate, L=1.0m
- Thickness of the plate, h=0.0035m
- Displacement (shear), x=0.02cm=0.0002m (conversion from cm to m)
- Shear modulus of brass, η=0.4×1011N/m2
Step 2: Calculate the area of the plate
The area A on which the force is applied is given by:
A=L×h=1.0m×0.0035m=0.0035m2
Step 3: Calculate the shear strain
Shear strain γ is defined as the ratio of the displacement to the height:
γ=xh=0.0002m1.0m=0.0002
Step 4: Relate stress, strain, and shear modulus
The relationship between shear stress τ, shear modulus η, and shear strain γ is given by:
τ=η⋅γ
Step 5: Calculate the shear stress
Substituting the values into the equation:
τ=0.4×1011N/m2×0.0002=8×107N/m2
Step 6: Calculate the force F
Shear stress is also defined as the force per unit area:
τ=FA
Rearranging this gives:
F=τ⋅A
Substituting the values:
F=8×107N/m2×0.0035m2=280000N=2.8×105N
Final Answer
The value of the force F is 2.8×105N.
---
- Side of the square plate, L=1.0m
- Thickness of the plate, h=0.0035m
- Displacement (shear), x=0.02cm=0.0002m (conversion from cm to m)
- Shear modulus of brass, η=0.4×1011N/m2
The area A on which the force is applied is given by:
A=L×h=1.0m×0.0035m=0.0035m2
Shear strain γ is defined as the ratio of the displacement to the height:
γ=xh=0.0002m1.0m=0.0002
The relationship between shear stress τ, shear modulus η, and shear strain γ is given by:
τ=η⋅γ
Substituting the values into the equation:
τ=0.4×1011N/m2×0.0002=8×107N/m2
Shear stress is also defined as the force per unit area:
τ=FA
Rearranging this gives:
F=τ⋅A
Substituting the values:
F=8×107N/m2×0.0035m2=280000N=2.8×105N
The value of the force F is 2.8×105N.
Topper's Solved these Questions
- ELASTICITY AND VISCOCITYBOOK - RESONANCECHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Exercise- 2 PART - I
11 Videos
- ELASTICITY AND VISCOCITYBOOK - RESONANCECHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Exercise- 2 PART - II
8 Videos
- ELASTICITY AND VISCOCITYBOOK - RESONANCECHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Exercise- 1 PART - I
8 Videos
- DAILY PRACTICE PROBLEMSBOOK - RESONANCECHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 92 illustration
2 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - RESONANCECHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercise
43 Videos
Similar Questions
Knowledge Check
One of the square faces of a metal slab of side 50 cm and thickness 20 cm is rigidly fixed on a horizontal surface. If a tangential force of 30 N is applied to the top face and it is known that the shear modulus of the material is 4×1010N/m2, then the displacement (in m) of the top face is
A4×10−12mB4×10−10mC6×10−10mD8×10−10mHow much force is required to produce an increase of 0.2 % in the length of a brass wire of diameter 0.6 mm
(Young's modulus for brass =0.9×1011N/m2)ANearly 17 NBNearly 34 NCNearly 51 NDNearly 68 NA square lead slab of side 50 cm and thickness 10 cm is subjected to a shearing force (on its narrow face) of 9×104N. The lower edge is riveted to the floor. How much will the upper edge be displaced? (Shear modulus of lead=5.6×109Nm−2)
A0.16 mmB1.6 mmC0.16 cmD1.6 cm
A square lead slab of side 50cm and thickness 5.0cm is subjected to a shearing force (on its narrow face) of magnitude 9.0×104 N. The lower edge is riveted to the floor. How much is the upper edge displaced, if the shear modulus of lead is 5.6×1090 Pa?
View SolutionA rubber cube of side 5cm has one side fixed while a tangential force equal to 1800N is applied to opposite face. Find the shearing strain and the lateral displacement of the strained face. Modulus of rigidity for rubber is 2.4×106N/m2.
View SolutionA square lead slab of side 50 cm and thickness 10 cm is subjected to a shearing force of 9.0×104N. The lower edge of the slab is fixed to the floor. The upper edge of the slab is displaced by 0.16mm . The Youn's modulus for the lead is
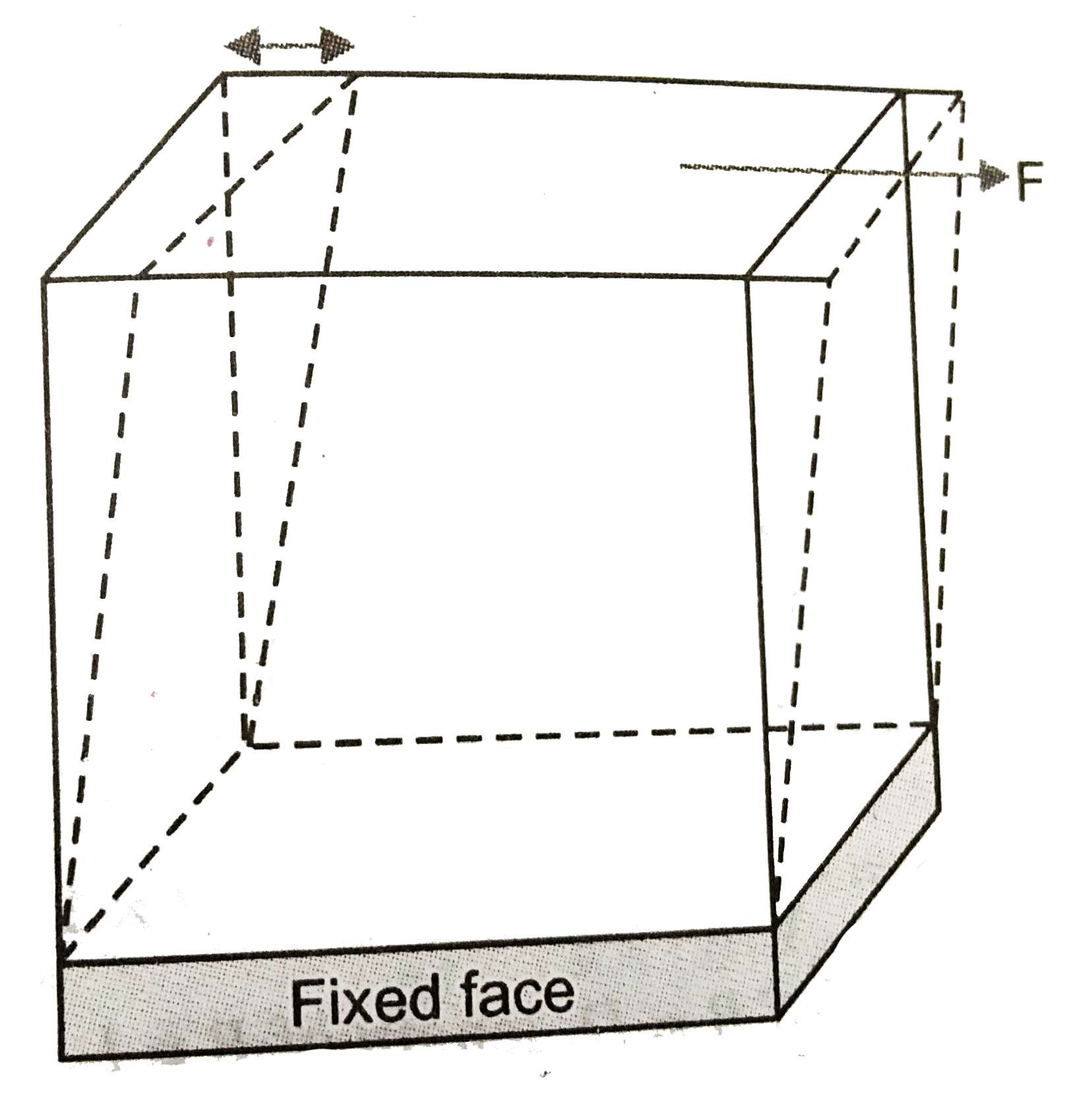
View SolutionA lead cube of side 20 cm is subjected under a shearing force of magnitude 5.6x106N. If the lower face of the cube is fixed, then the displacement in the upper face is (If shear modulus of lead is 5.6⋅106Nm2)
View SolutionThe diameter of a brass rod is 4 mm and Young's modulus of brass is 9×1010N/m2. The force required to stretch by 0.1 % of its length is
View Solution
