A soap bubble of radius r1 is placed on another soap bubble of radius r2(r1<r2). The radius R of the soapy film separating the two bubbles is :
r1+r2
√r21+r22
(r31+r32)
r2r1r2−r1
The correct Answer is:D
To find the radius R of the soapy film separating two soap bubbles of radii r1 and r2 (where r1<r2), we can use the concept of pressure differences due to surface tension. Here’s a step-by-step solution:
Step 1: Understand the Pressure Inside the Bubbles
The pressure inside a soap bubble is given by the formula:
P=P0+4σr
where P0 is the atmospheric pressure, σ is the surface tension, and r is the radius of the bubble.
Step 2: Write the Pressure Equations
For the smaller bubble (radius r1):
P1=P0+4σr1
For the larger bubble (radius r2):
P2=P0+4σr2
Step 3: Set Up the Pressure Difference
Since the pressure inside the smaller bubble is greater than that inside the larger bubble, we can express the pressure difference when moving from the first bubble to the second bubble:
P1−P2=4σr1−4σr2
Step 4: Relate the Pressure Difference to the Soap Film
The pressure difference across the soap film is also given by:
P1−P2=4σR
where R is the radius of the soap film.
Step 5: Equate the Two Expressions
Now we can set the two expressions for the pressure difference equal to each other:
4σR=4σr1−4σr2
Step 6: Simplify the Equation
We can cancel 4σ from both sides (assuming σ≠0):
1R=1r1−1r2
Step 7: Find a Common Denominator
To combine the fractions on the right side, we find a common denominator:
1R=r2−r1r1r2
Step 8: Invert to Find R
Taking the reciprocal gives us:
R=r1r2r2−r1
Final Answer
Thus, the radius R of the soapy film separating the two bubbles is:
R=r1r2r2−r1
The pressure inside a soap bubble is given by the formula:
P=P0+4σr
where P0 is the atmospheric pressure, σ is the surface tension, and r is the radius of the bubble.
For the smaller bubble (radius r1):
P1=P0+4σr1
For the larger bubble (radius r2):
P2=P0+4σr2
Since the pressure inside the smaller bubble is greater than that inside the larger bubble, we can express the pressure difference when moving from the first bubble to the second bubble:
P1−P2=4σr1−4σr2
The pressure difference across the soap film is also given by:
P1−P2=4σR
where R is the radius of the soap film.
Now we can set the two expressions for the pressure difference equal to each other:
4σR=4σr1−4σr2
We can cancel 4σ from both sides (assuming σ≠0):
1R=1r1−1r2
To combine the fractions on the right side, we find a common denominator:
1R=r2−r1r1r2
Taking the reciprocal gives us:
R=r1r2r2−r1
Thus, the radius R of the soapy film separating the two bubbles is:
R=r1r2r2−r1
Topper's Solved these Questions
- SURFACE TENSIONBOOK - RESONANCECHAPTER - SURFACE TENSIONEXERCISE - Exercise- 2 PART - II
15 Videos
- SURFACE TENSIONBOOK - RESONANCECHAPTER - SURFACE TENSIONEXERCISE - Exercise- 2 PART - III
3 Videos
- SURFACE TENSIONBOOK - RESONANCECHAPTER - SURFACE TENSIONEXERCISE - Exercise- 1 PART - II
17 Videos
- STRING WAVESBOOK - RESONANCECHAPTER - STRING WAVESEXERCISE - Exercise
32 Videos
- UNITS, DIMENSION & MEASUREMENTBOOK - RESONANCECHAPTER - UNITS, DIMENSION & MEASUREMENTEXERCISE - Exercise
27 Videos
Similar Questions
Two soap bubbles of radius r1 and r2 combine. Find radius of curvature of the common surface separating them.

View SolutionA soap bubble of radius r is formed inside another soap bubble of radius R(>r). The atmospheric pressure is P0 and surface tension of the soap solution is T. Calculate change in radius of the smaller bubble if the outer bubble bursts. Assume that the excess pressure inside a bubble is small compared to P0.
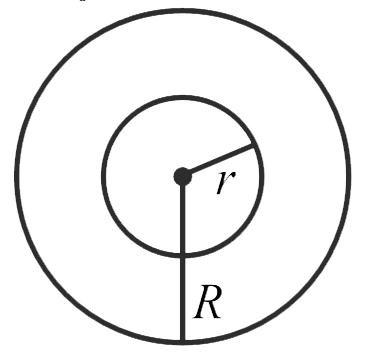
View Solution
Knowledge Check
A soap bubble of radius r is placed on another bubble of radius 2r. The radius of the surface common to both the bubbles is
A2r3B3rC2rDrA soap buble of radius 1.0 cm is formed inside another soap bubble of radius 2.0 cm . The radius of an another soap bubble which has the same pressure difference as that between the inside of the smaller and outside of large soap bubble , in metres is
A6.67×10−3B3.34×10−3C2.23×10−3D4.5×10−3Prove that if two bubbles of radii r1 and r2(r1<r2) come in contact with each other then the radius of curvature of the common surface r=r1r2r2−r1
Ar2−r1Br2−r1r1r2Cr1r2r2−r1Dr2+r1
A soap bubble of raidus R is surrounded by another soap bubble of radius 2R, as shown. Take surface tension =S. Then the pressure inside the smaller soap bubble, in excess of the atmosphere presure will be
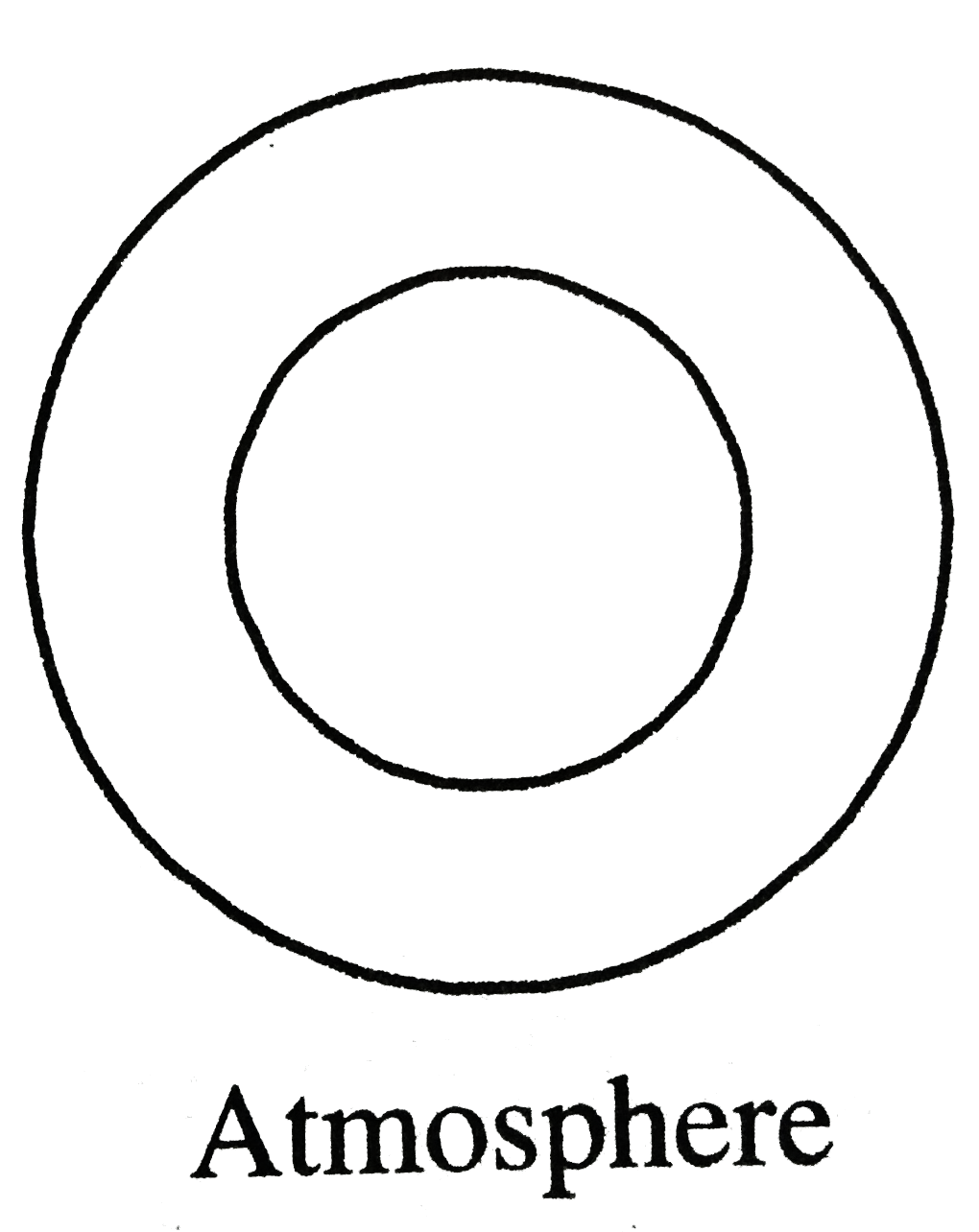
View SolutionIf a soap bubble of radius 3 cm coalesce with another soap bubble of radius 4 cm under isothermal conditions the radius of the redultant bubble formed is in cm
View SolutionA soap bubble of radius 6 cm and another bubble of 8 cm coalesce under isothermal xonditions in vacuum. The radius of the new bubble is
View SolutionA soap - bubble with a radius 'r' is placed on another bubbles with a radius R (figure). Angles between the film at the point of contact will be -

View SolutionIf two soap bubbles of equal radii r coalesce then the radius of curvature of interface between two bubbles will be
View Solution
