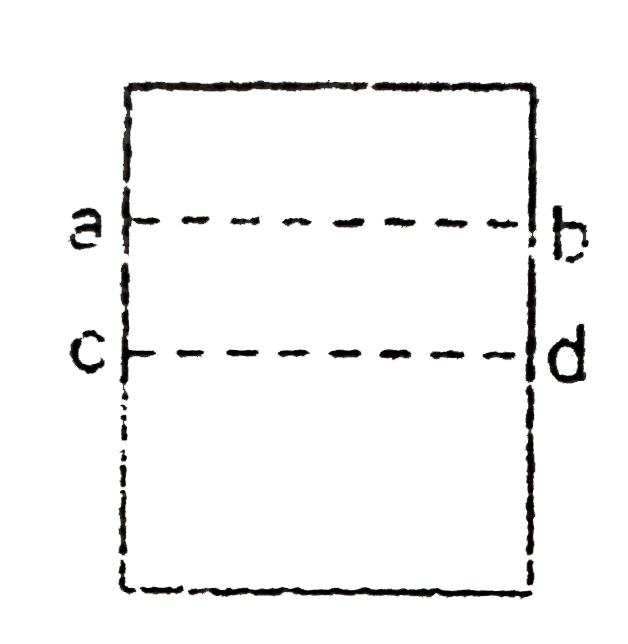
A soap film in stretched over a reclangular vertical wire frame as shown in the figure, what forces hold section abod in equilibrium ?
The correct Answer is:Surface tension forces Fab,Fcd and weight. Equilibrium only Fab>Fcd and this is due to difference in concentration of soap solution in film.
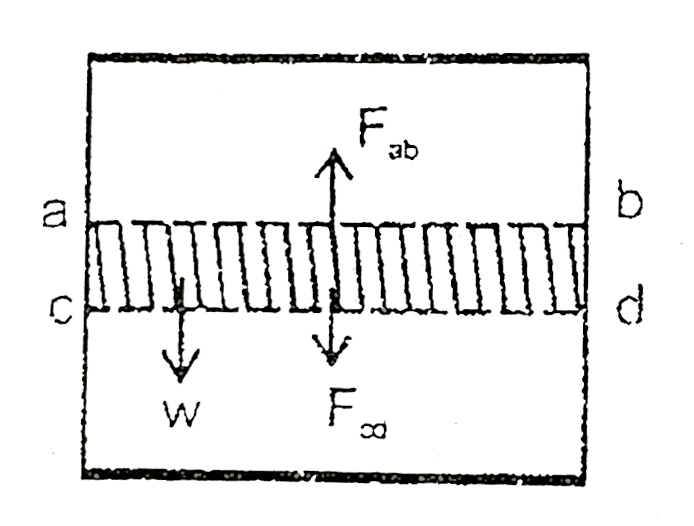
The FBD of part abod is as shown. The surface tension forces Fab upward, Fcd downward and weight (w) of part abod are holding the part abcd in equilibrium.
Fab−Fcd=w.
Clearly Fab>Fcd and this is due to difference in concentration of soap solution in film.
Topper's Solved these Questions
- SURFACE TENSIONBook:RESONANCEChapter:SURFACE TENSIONExercise:Exercise- 1 PART - II
Explore 17 Videos
- SURFACE TENSIONBook:RESONANCEChapter:SURFACE TENSIONExercise:Exercise- 2 PART - I
Explore 7 Videos
- SURFACE TENSIONBook:RESONANCEChapter:SURFACE TENSIONExercise:Advanced Level Problems
Explore 18 Videos
- STRING WAVESBook:RESONANCEChapter:STRING WAVESExercise:Exercise
Explore 32 Videos
- UNITS, DIMENSION & MEASUREMENTBook:RESONANCEChapter:UNITS, DIMENSION & MEASUREMENTExercise:Exercise
Explore 27 Videos
Similar Questions
Knowledge Check
A soap film is created in a small wire frame as shown in the figure. The sliding wire of mass m is given a velocity u to the right and assume that u is small enough so that film does not break. Plane of the film is horizontal and surface tension is T, then time to region the original position of wire is equal to :
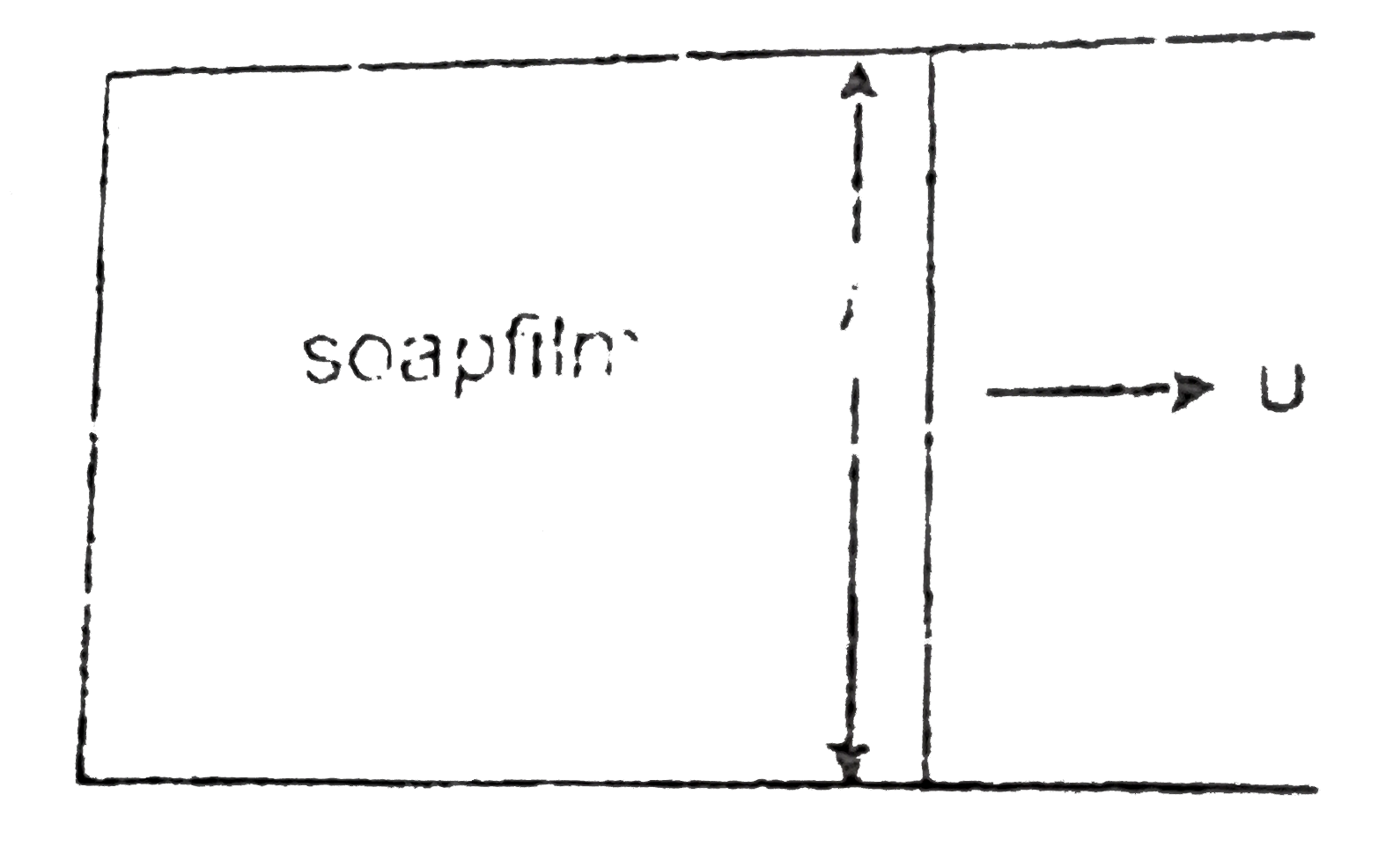 AumTlBTlumCmu2lTDIt will never regain original position
AumTlBTlumCmu2lTDIt will never regain original positionA uniform bar of mass m is supported by a pivot at its top about which the bar can swing like a pendulum. If a force F is applied perpendicular to the lower end of the bar as shown in figure, what is the value of F in order to hold the bar in equilibrium at an angle (θ) from the vertical
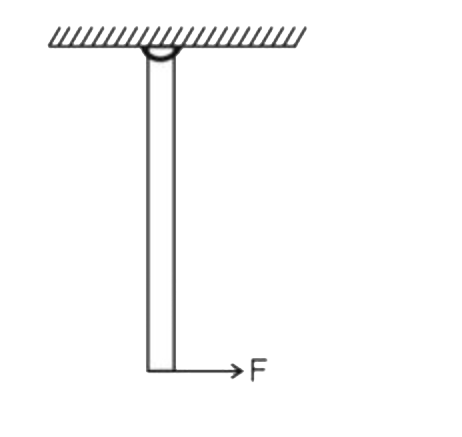 A2mgsinθBmgsinθCmg2sinθDmg2cosθ
A2mgsinθBmgsinθCmg2sinθDmg2cosθA thin and light thread of sufficient length L is attached to a wire frame. The frame (along with the thread) is dipped into soap solution. When the frame is taken out of the solution, the thread takes the form of a semicircle with a soap film extending between the frame and the thread as shown in the figure (a). Now the thread is deformed into two semicircles by applying a force of F at the middle of the thread, as a result the film gets strectched as shown in the figure. (b). Calculate the surface tension of the soap solution.
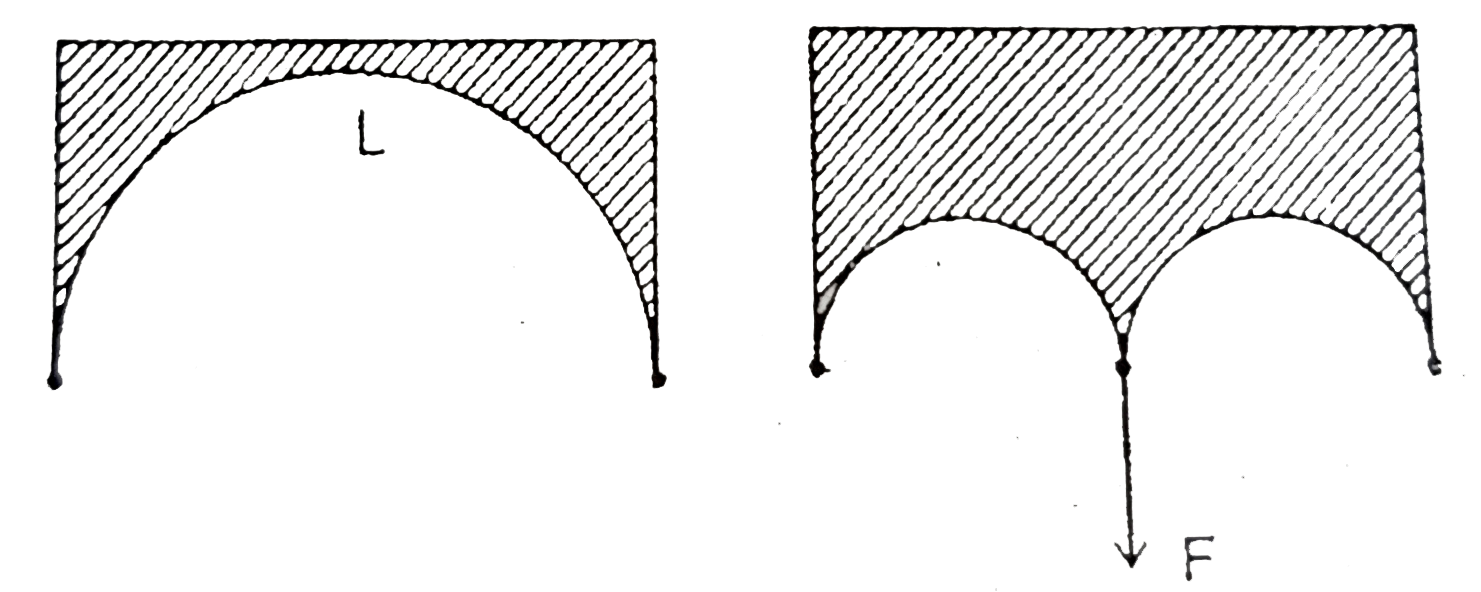 AπF2LBπFLC2πFLD14πFL
AπF2LBπFLC2πFLD14πFL
A light wire AB of length 10 cm can slide on a vertical frame as shown in figure. There is a film of soap solution trapped between the frame and the wire. Find the load W that should be suspended from the wire to keep it in equilibrium. Neglect friction. Surface tension of soat solution =25dy≠cm−1.Takeg=10ms−2

View SolutionA wire wound over a rod and placed over a scale, as shown in figure. Calculate the radius of the wire.

View SolutionA wire is wound over a pencil and placed over a scale as shown in the figure. The diameter of the given wire is ________.
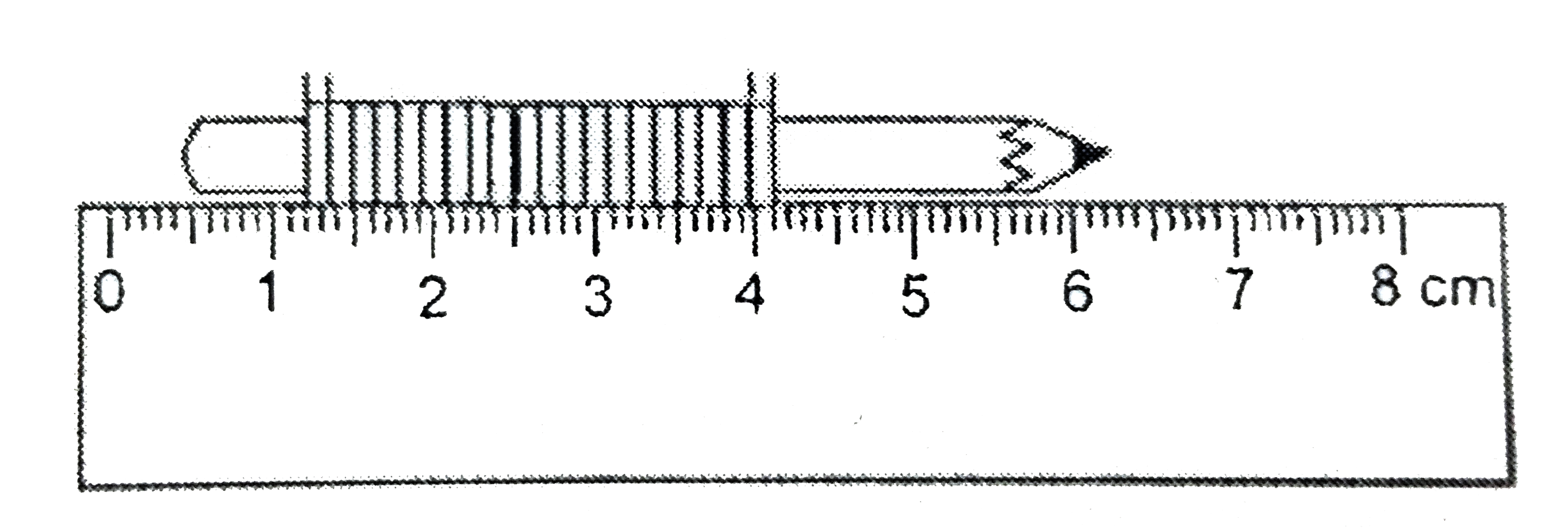
View SolutionA wire is wound over a pencil and placed over a scale as shown in the figure. The diameter of the given wire is ________.

View SolutionA wire is wound over a pencil and placed over a scale as shown in the figure. The diameter of the given wire is ________.

View Solution